Build Mathematical Literacy 1-2 Answers

Grades k–2

Growing Mathematical Ideas in Kindergarten

by Linda Schulman Dacey and Rebeka Eston Kindergarten is an important beginning. It can be the positive start of a child's lifelong exploration of mathematical ideas or it can lay the first stones in what can become an impenetrable wall between "real math" and "school math." In Growing Mathematical Ideas in Kindergarten, Linda and Rebeka…

View Lesson

Grades k–2

Classroom Discussions: Using Math Talk in Elementary Classrooms

Teachers typically are comfortable leading classroom discussions when teaching literature or providing social studies instruction. They value these discussions and rely on them to support students' learning. However, many teachers aren't as comfortable making use of classroom discussions for mathematics instruction. In Classroom Discussions: Using Math Talk to Help Students Learn, Grades 1–6 (Math Solutions…

View Lesson

Grades k–2

"What's This One Called?"

All too often young children fail to remember the names of pattern block shapes. Over the years, Kristin Garrison has found that if teachers don't give students frequent opportunities to use pattern block names and become familiar with the attributes of the shapes, when handling pattern blocks after they leave kindergarten and first grade, they…

View Lesson

Grades k–2

How Many Feet in the Bed?

In this lesson, students hear the story How Many Feet in the Bed? by Diane Johnston Hamm, and predict how many feet are in the bed as, one after another, family members hop into Mom and Dad's big bed on a Sunday morning. This book helps young children develop algebraic thinking skills by having them…

View Lesson

Grades k–2

My Map Book

Sara Fanelli's My Map Book (HarperCollins, 1995) is filled with maps of things that are of importance in a child's life—"Map of My Day," "Map of My Neighborhood," "Map of My Face," and more. In this lesson, excerpted from Jamee Petersen's Math and Nonfiction, Grade K–2 (Math Solutions Publications, 2004), second-grade students draw maps of…

View Lesson

Grades k–2

Place Value Foundations

The key to learning mathematics is understanding the "why" behind the "how". HMH Into Math emphasizes the importance of establishing conceptual understanding and reinforces that understanding with procedural practice. The learning model asks students to first develop their reasoning before connecting their understanding to concepts and skills. HMH Into Math is more than just a solution,…

View Lesson

Grades k–2

Hundred Chart Game

First grade students practice adding and subtracting by tens in this hundred chart game. The key to learning mathematics is understanding the "why" behind the "how". HMH Into Math emphasizes the importance of establishing conceptual understanding and reinforces that understanding with procedural practice. The learning model asks students to first develop their reasoning before connecting their…

View Lesson

Grades k–2

Hold and Fold

A Lesson for First and Second Graders by Chris Confer This whole-class lesson is adapted from the Math By All Means replacement unit Geometry, Grades 1–2, written by Chris Confer. By folding a square of paper in several predetermined ways, children investigate and record the different shapes they can make. This activity gives children valuable…

View Lesson

Grades k–2

Identifying and Describing Polygons

A Geometry Lesson Overview In this lesson, students learn to identify and describe polygons and compare and contrast them with figures that are not polygons. Prior to the lesson, students are introduced to vocabulary words that they will need to use as they learn about polygons. Students are taught various sentence frames and use the…

View Lesson

Grades k–2

Modeling Subtraction

Before taking my class for a leaf-collecting walk, I distributed 9-by-12-inch drawing paper and asked the children each to draw a picture of a tree. "Don't put any leaves on your tree," I said. "We'll collect leaves on the playground and you'll arrange those on your trees." Before we went outside, I gave one…

View Lesson

Grades k–2

Growing Mathematical Ideas in Kindergarten

View Lesson

Grades k–2

Solving Real-World Problems

A Lesson for First Graders by Chris Confer When children use mathematics to solve real-world problems, they learn that mathematics is not just something to do for the teacher's sake, but it offers them important tools to shape their world. In this lesson, which appears in Chris Confer's new book Teaching Number Sense, Grade 1…

View Lesson

Grades k–2

Post-Assessment Tasks K-2

Open-ended problems can make for excellent post-assessment. Wondering how you can design effective post-assessment tasks for your students? This lesson gives a four-step plan, including a K–2 sample task and corresponding authentic student responses. The lesson is adapted from Math for All: Differentiating Instruction, Grades K–2, by Linda Dacey and Rebeka Eston Salemi. Visit www.mathsolutions.com…

View Lesson

Grades k–2

More than One

A Lesson for First and Second Graders By Jamee Petersen Miriam Schlein's More than One (New York: Greenwillow, 1996) introduces the concept that one unit can be made up of more than one thing. One pair is always two, one week is seven days, but one family can be two, three, or more people. Here,…

View Lesson

Grades k–2

The Door Project

by Rosemarie Dyer Rosemarie Dyer has taught for almost 30 years, primarily in kindergarten and first grade. She taught this activity, in which children collect information about the doors in the school, to her first-grade class in the Warren Consolidated School District, located in suburban Detroit. The lesson gives the children experience recording, organizing, and…

View Lesson

Grades k–2

Numbers  at  School

A  Lesson  for  Kindergartners by  Chris  Confer In  this  lesson,  excerpted  from  Chris  Confer's  new  book  Teaching  Number  Sense, Kindergarten  (Math  Solutions  Publications,  2005),  children  learn  that  numbers  are  used  for  different  purposes. They  search  for  numbers  in  their  school,  draw  pictures  of  things  that  have  numbers, discuss  how  numbers  help  people, as  well  as  talk  to  adults  in…

View Lesson

Grades k–2

More or Less?

A Lesson with Kindergartners and First and Second Graders by Rusty Bresser and Caren Holtzman The concepts of more, less, and the same are basic relationships contributing to the overall concept of number. In this activity, students gain experience with these concepts and are also asked to think about part-part-whole relationships. (For example, students see that…

View Lesson

Grades k–2

Collecting, Representing, & Interpreting Data

A Lesson for Kindergartners and First and Second Graders by Linda Dacey and Rebeka Eston The collection and display of data are important to our lives, and through their own investigations, young children begin to understand how they can find and communicate information in data, charts, and graphs. In the following excerpt from Show and Tell: Representing…

View Lesson

Grades k–2

Working with Counting Cups

by Ann Carlyle Children need a good deal of practice counting objects. Second language learners especially need many opportunities to deal with words such as more, less, fewer, most, least, fewest, some, in order, and equal. Working with Counting Cups can be repeated from time to time throughout the year to reinforce children's counting, ordering,…

View Lesson

Grades k–2

Math in the Early Childhood Classroom

by Ann Carlyle Ann Carlyle, recent recipient of the George Polya Memorial Award from the California Mathematics Council, has been teaching kindergarten through sixth grade in Goleta, California, for the past 34 years. As a Math Solutions consultant, she often receives letters from course participants asking her for specific help with their math instruction. The…

View Lesson

Grades k–2

Quick as a Cricket

by Lisa Rogers Audrey Wood's book Quick as a Cricket (Child's Play, 1982) uses rhymes and beautiful illustrations to take children on a joyful celebration of self-awareness. The book features a boy who compares himself to a variety of animals—"loud as a lion," "quiet as a clam," "tough as a rhino," and "gentle as a…

View Lesson

Grades k–2

Helping English Language Learners Make Sense of Math Word Problems

Rusty Bresser, Kathy Melanese, and Christine Sphar Alison Williams's second-grade class has the full range of English language learners (ELLs), from beginning to advanced, including a few native English speakers. Native languages spoken by the students include English, Vietnamese, Spanish, Somali, and Laotian. Alison knows that ELLs typically experience difficulty understanding and therefore solving math…

View Lesson

Grades k–2

Five Speckled Frogs

A Lesson for Grades K–1 by Dana Islas Materials • The children's rhyming story Five Little Speckled Frogs, by Nikki Smith (Nikki Smith Books, 2006) • Cubes, 5 per student Overview of Lesson In the delightful rhyme Five Little Speckled Frogs, five frogs sitting on a log gulp bugs and jump into a pool one…

View Lesson

Grades k–2

Ten-Frames Lesson: Race to 20

Overview In this game, students roll a die and place that number of counters on a double ten-frame in an eﬀort to reach 20 ﬁrst. This game builds students' understanding of landmark numbers, speciﬁcally ten and twenty. Through the use of two colors of counters, students decompose the number twenty and use number strings…

View Lesson

Grades k–2

Farmer's Math

by Ann Carlyle Ann Carlyle, a Math Solutions Inservice consultant, has been an elementary teacher in Goleta, California, for more than 30 years. She is the 1993 Presidential Award winner for Excellence in Mathematics Teaching and the 2000 recipient of the George Polya Memorial Award from the California Mathematics Council. Farmer's Math grew out of…

View Lesson

Grades k–2

Teaching The Greedy Triangle

by Lynn Sherman Lynn Sherman is a teacher candidate at York University in Toronto, Ontario, where she recently taught a geometry class based on The Greedy Triangle, by Marilyn Burns (Scholastic, 1994), to a class of second graders. She gave the story an added dimension with the creation of her Greedy Triangle "prop." We're sure…

View Lesson

Grades k–2

The Icky Bug Counting Book

by Vicki Bachman The end of the school year is both an exhilarating and a challenging time. Unless you're in a year-round school, everyone is getting excited about the summer vacation ahead. Getting—and holding—the children's attention requires planning, flexibility, and energy. A balance of novelty, familiar routines, and physical activity—customized to your class and personal…

View Lesson

Grades k–2

Developing Operations Sense

by Stephanie Sheffield Stephanie Sheffield begins this lesson in her first-grade classroom by reading aloud Ann Jonas's book Splash! Each page shows what is above and below the water of a young girl's backyard pond. This girl narrates the story about animals jumping into and out of the pond and continually asks the reader, "How many are…

View Lesson

Grades k–2

Mary Wore Her Red Dress: A Lesson in Counting Attributes

by Dana Islas Overview of Lesson Mary Wore Her Red Dress and Harry Wore His Green Sneakers is a favorite book to share with students at the beginning of the school year. In the book, animals in assorted brightly colored clothes assemble for their friend Katy's grand birthday gala. After reading the book, students look…

View Lesson

Grades k–2

Counting Crocodiles

by Andrea Holmes Andrea Holmes knew that the kindergarten children in Mrs. Fisher's class had had numerous opportunities to listen to and engage with counting books. She also knew that additional practice allows children to continually think about numbers from one to ten and provides a glimpse of the problem- solving strategies children rely upon…

View Lesson

Grades k–2

Big and Little

by Jamee Petersen Understanding the concept of scale is not easy for young children, but Steve Jenkins's book Big and Little (Houghton Mifflin, 1996) can help. The illustrations in the book show animals at the same scale, making it easy to compare their sizes visually. In this lesson, Jamee Petersen uses the book to introduce…

View Lesson

Grades k–2

Comparing Handfuls 1st Grade

by Leyani von Rotz and Marilyn Burns Learning the meaning of the less than, greater than, and equals signs is important to children's numerical and algebraic understanding. In this lesson, first-grade children use the symbols >, <, and = to compare numbers. This activity appears in Leyani von Rotz and Marilyn Burns's new book, Lessons…

View Lesson

Grades k–2

The Button Game

Pour your bag of buttons onto a plate so that everyone can easily see them. Hold up a button and discuss the article of clothing that it might have been attached to. Hold up another button and call attention to the ways in which the buttons are alike and different.

View Lesson

Grades k–2

Alexander Used to Be Rich

Jane Crawford Overview of Lesson This lesson is appropriate for children who recognize coins and have been introduced to coin values. Follow the instructions in the lesson that allow different levels of ability to participate. Many children can relate to Alexander's quandary in the book Alexander, Who Used to Be Rich Last Sunday. Feeling rich…

View Lesson

Grades k–2

Caterpillars

A Lesson with First Graders by Leyani von Rotz and Marilyn Burns Building, extending, and describing growth patterns is an important aspect of developing children's algebraic thinking. In this lesson, children investigate caterpillars that grow, record on a T-chart, and then extend the pattern. The activity appears in Leyani von Rotz and Marilyn Burns's new…

View Lesson

Grades k–2

Rod Towers

by Vicki Bachman In this activity, children build and then determine the height of Cuisenaire rod towers. Students build a tower with a partner, decide the measurement tool to use, measure their tower, and then in a whole-class discussion make comparisons between the towers. Rod Towers is excerpted from Vicki Bachman's new book, Sizing Up…

View Lesson

Grades k–2

Evens and Odds

by Stephanie Sheffield Many first-grade children have learned to count by twos, at least up to ten. Some have learned the chant" Two, four, six, eight, who do we appreciate." And some know that when things are the same, we often say that they are "even." However, most first graders have not thought about the…

View Lesson

Grades k–2

Breaking Numbers Apart

by Rusty Bresser and Caren Holtzman The lesson is excerpted from Minilessons for Math Practice, Grades K–2, by Rusty Bresser and Caren Holtzman (Math Solutions Publications, 2006). The book provides engaging, quick activities to help students practice math concepts, skills, and processes in a variety of problem-solving contexts throughout the day. This sample offers two…

View Lesson

Grades k–2

Guess My Number K-2

by Rusty Bresser and Caren Holtzman In this activity, students guess a secret number from within a range of numbers and keep refining their guesses based on whether the teacher tells them the secret number is greater or lesser than their guesses. Guess My Number, which appears in Rusty Bresser and Caren Holtzman's Minilessons for…

View Lesson

Grades k–2

"What's This One Called?"

A Lesson for Kindergartners and First Graders by Kristin Garrison All too often young children fail to remember the names of pattern block shapes. Over the years, Kristin Garrison has found that if teachers don't give students frequent opportunities to use pattern block names and become familiar with the attributes of the shapes, when handling…

View Lesson

Grades k–2

The Shape of Things

A Lesson for Kindergartners By Marilyn Burns Dayle Ann Dodds wrote that she developed the idea for her book The Shape of Things (Candlewick, 1994) to help children see "how a few simple shapes make up a lot of things we have in the world." After reading the book to a kindergarten class, Leyani von…

View Lesson

Grades 2–3

Categorical Data Collection

The need to interpret data accurately looms large in today's world. By modeling ways to gather, represent, and interpret data, teachers can make young children feel more comfortable in this arena; children can then do these activities independently. Categorical Data Collection appears in the "December" chapter of Nancy Litton's new book, Second-Grade Math: A Month-to-Month…

View Lesson

Grades 2–3

The Long and Short of It

Each two-page spread in Cheryl Nathan and Lisa McCourt's book The Long and Short of It (BridgeWater Books, 1998) shows two animals and compares the size of some part common to both of them by comparing each part to an everyday object. For example, the chameleon's tongue is described as being "longer than a fire…

View Lesson

Grades 2–3

Chasing Vermeer

In Blue Balliett's novel Chasing Vermeer (Scholastic, 2005), Petra and Calder, the main characters, are in the same class but barely know each other. Their friendship grows, however, and they work together to recover a stolen painting—a valuable Vermeer. Pentominoes are included in the clues they need to decode. Maryann Wickett uses this book as a…

View Lesson

Grades 2–3

Telephone Number Totals

Children are surrounded by things containing numbers — license plates, addresses, room numbers, shoe sizes, signs, even telephone numbers. One way that students can develop number sense is to think about the numbers they encounter in the everyday world. This lesson by Bonnie Tank and Lynne Zolli provides a playful experience with numbers that are…

View Lesson

Grades 2–3

The 512 Ants on Sullivan Street

A Lesson for Third Graders by Maryann Wickett and Marilyn Burns This lesson is excerpted from Maryann Wickett and Marilyn Burns's new book, Teaching Arithmetic: Lessons for Extending Place Value, Grade 3 (Math Solutions Publications, 2005). Children's understanding of place value is key to their arithmetic success with larger numbers, and this book is important…

View Lesson

Grades 2–3

Revisiting Addition and Subtraction

Students beneﬁt from repeated practice with addition and subtraction throughout the year. In her book, Third-Grade Math: A Month-to-Month Guide (Math Solutions Publications, 2003), Suzy Ronfeldt provides a midyear perspective on providing practice, suggesting fresh approaches to computing with larger numbers that are suitable for older students as well. The problems are useful not only…

View Lesson

Grades 2–3

Amanda Bean's Amazing Dream: A Mathematical Story

Amanda Bean loves to count anything and everything. But sometimes she just can't count fast enough. Her teacher tries to convince her that multiplying might help, but Amanda will have none of it—until she has an amazing dream about free-wheeling sheep on bicycles, speed-knitting grandmas, and who knows how many long-sleeved sweaters. Only then does…

View Lesson

Grades 2–3

Relationships in the Metric System

The metric system is particularly easy to work with since its units relate to each other in the same way that units in place value relate to each other: powers of ten. This activity helps make that connection for students. Here students compare centimeter cubes, decimeter rods, and meter sticks and find all the ways…

View Lesson

Grades 2–3

Body Measurements

This lesson appears in Bonnie Tank and Lynne Zolli's new book, Teaching Arithmetic: Lessons for Addition and Subtraction, Grades 2–3 (Math Solutions Publications, 2001). Based on Rolf Myller's book How Big Is a Foot?, this lesson gives children experience with comparing quantities in the context of measuring a variety of lengths. Partners measure, record, and…

View Lesson

Grades 2–3

Learning Vocabulary with Tangrams

Vocabulary instruction is a large part of geometry instruction throughout the elementary grades. To learn geometric terms and their meanings, students need opportunities to interact with and use the language of geometry. In this lesson, Maryann Wickett used the experience of making tangrams as an opportunity to help a class of third graders expand their…

View Lesson

Grades 2–3

Developing Algebraic Thinking Using Manipulatives

Algebra Content Standards • Create numeric patterns that involve whole-number operations. (3-3.1) • Apply procedures to find missing numbers in numeric patterns that involve whole-number operations. (3-3.2) • Illustrate situations that show change over time as increasing. (3-3.4) Lesson Process Standards • Generate descriptions and mathematical statements about relationships. (3-1.4) …

View Lesson

Grades 2–3

Looking at Data

This activity, Looking at Data, is excerpted from Mini-lessons for Math Practice, Grades 3–5, by Rusty Bresser and Caren Holtzman (Math Solutions Publications, 2006). The book presents ideas for providing opportunities for students to practice the things they have learned, with practice defined broadly to include understanding as well as skill. In this instance, students…

View Lesson

Grades 2–3

Divide and Ride

Dividing a number into equal-size groups with remainders is the main focus of Stuart J. Murphy's book Divide and Ride (Harper Trophy, 1997). In this story, eleven friends go to a carnival. When they must get into groups of two to ride the roller coaster, groups of three for the satellite wheel, and groups of…

View Lesson

Grades 2–3

What Could Be the Sum?

It's important to make connections among the different areas of mathematics, and this lesson presents an addition problem in a geometric context that is appropriate for third graders. The problem also is good for supporting mental computation and for giving children experience with a math problem that has more than one solution. The idea for…

View Lesson

Grades 2–3

Estimation Jar

Estimation Jar is excerpted from Susan Scharton's book, Teaching Number Sense, Grade 2 (Math Solutions Publications, 2005), part of a three-book series for grades K, 1, and 2 that focuses on the critical role number sense plays in students' math learning. This lesson is one in a series of estimation activities that Susan includes in…

View Lesson

Grades 2–3

Which Has More?

Lessons for Introducing Multiplication, Grade 3 is a revision that replaces Multiplication, Grade 3, the Math By All Means unit I wrote in 1991 that has been used by more than 85,000 teachers. Over the years since I wrote the original unit, I've learned a good deal more about teaching multiplication to third graders from…

View Lesson

Grades 2–3

Everybody Wins!

This excerpt is from the introductory lesson in Maryann Wickett, Susan Ohanian, and Marilyn Burns's book, Teaching Arithmetic: Lessons for Introducing Division, Grades 3–4 (Math Solutions Publications, 2002). This book is a revision of the popular Math By All Means unit Division, Grades 3–4, and this lesson is one of the new additions. The context…

View Lesson

Grades 2–3

Understanding and Memorizing Multiplication Facts

I prepared a baggie for each pair of children, each with one hundred identical objects such as cubes, milk jug lids, pennies, beans, tiles, and so on. For several days, the children used these materials to build different-size arrays. For instance, I'd ask them to build a row of four, six times, and walk around…

View Lesson

Grades 2–3

Exploring Halves

In this lesson, students explore halves, looking for patterns between numerators and denominators. Maryann Wickett created this simple yet powerful fractions lesson and then built on it, doing an activity from Marilyn Burns's Teaching Arithmetic: Lessons for Introducing Fractions, Grades 4–5 (Math Solutions Publications, 2001). My-third grade students had experience using fraction kits to informally…

View Lesson

Grades 2–3

Twenty Questions: A Lesson Using the Hundreds Chart 3-5

Overview of Lesson This lesson is a math variation of the popular 20 questions game. The teacher chooses a secret number on the 1–100 chart. Students ask 20 questions to try to ascertain the secret number. Students mark their 1–100 charts to keep a visual record of information they have gathered and to see the…

View Lesson

Grades 2–3

Exploring Ones, Tens, and Hundreds with Base Ten Blocks

In this lesson, excerpted from Maryann Wickett and Marilyn Burns's new book, Teaching Arithmetic: Lessons for Extending Place Value, Grade 3 (Math Solutions Publications, 2005), children use base ten blocks to cement their understanding of how ones, tens, and hundreds relate to our number system. Before class, I gathered the base ten blocks and enough…

View Lesson

Grades 2–3

Ten Friends

Bruce Goldstone's book Ten Friends (Henry Holt, 2001) uses rhymes and illustrations to suggest different ways to invite ten friends to tea. At the back, he lists all of the ways to represent ten using two addends, three addends, and so on up to ten addends. Marilyn Burns read the story to second graders and…

View Lesson

Grades 2–3

The Game of More

As part of their classroom routine, Bonnie Tank and Lynne Zolli regularly ask children to figure out answers to questions like "How many more?,""How many less?," and "What's the difference?" The Game of More provides a context for asking these questions. This card game gives children practice with basic facts and with adding and subtracting…

View Lesson

Grades 2–3

It All Adds Up! Engaging 8-to-12 Year-Olds in Math Investigations

When I ﬁrst saw a copy of It All Adds Up!, by Australian teacher Penny Skinner, I began reading it eagerly. I was searching for ways to teach arithmetic with the same excitement I had for the other areas of the math curriculum. In the introduction, Penny explains that her book explores teaching strategies for…

View Lesson

Grades 2–3

The Game of Tens and Ones

This game gives children practice with adding and subtracting ones and tens. Using a special die, two 0–99 charts, and two markers, children play in pairs. During the course of a game, they calculate between 20 and 30 addition and subtraction problems. The Game of Tens and Ones appears in Maryann Wickett and Marilyn Burns's…

View Lesson

Grades 2–3

Hand Spans

Hand Spans uses a measurement activity to give students experience with the grouping model of division and practice with rulers and tape measures. The students measure their hand span and the length of their arm, and then figure out how many of their hand span lengths are in their arm length. This lesson appears in…

View Lesson

Grades 2–3

Place Value Game

Second grade students use 3-digit numbers and their understanding of place value in this game. The key to learning mathematics is understanding the "why" behind the "how". HMH Into Math emphasizes the importance of establishing conceptual understanding and reinforces that understanding with procedural practice. The learning model asks students to first develop their reasoning before connecting…

View Lesson

Grades 2–3

Introduction to Coordinate Graphing

Along with teaching students how to use ordered pairs of numbers as coordinates to plot points, this lesson gives students background understanding about our system of graphing and helps them see how axes — intersecting perpendicular number lines — make it possible to locate points anywhere on a plane. The activity appears in Maryann Wickett,…

View Lesson

Grades 2–3

Area Partner Game

In this partner game, third grade students use their multiplication foundation to solve for the area of rectangles. The key to learning mathematics is understanding the "why" behind the "how". HMH Into Math emphasizes the importance of establishing conceptual understanding and reinforces that understanding with procedural practice. The learning model asks students to first develop their…

View Lesson

Grades 2–3

Estimating Length: Differentiating Within a Unit

A Lesson with Second Graders by Linda Dacey and Rebeka Eston Salemi All teachers have students with a range of mathematical abilities and understandings in their classrooms. In this lesson on estimation and measurement, the teacher differentiates three aspects of the curriculum—content, process, and products. This lesson is excerpted from Math for All: Differentiating Instruction,…

View Lesson

Grades 2–3

One Hundred Hungry Ants

This lesson is from Marilyn Burns's book Teaching Arithmetic: Lessons for Introducing Multiplication, Grade 3 (Math Solutions Publications, 2001), a revision of the Math By All Means: Multiplication, Grade 3 unit she wrote in 1991. This book presents five completely new whole-class lessons plus five new lessons in the Additional Activities section. Also, four children's…

View Lesson

Grades 2–3

Stranger in the Woods

Dana Islas is a Math Solutions consultant, kindergarten teacher at Pueblo Gardens Elementary School in Tucson, Arizona, recipient of the Presidential Award for Excellence in Mathematics and Science Teaching, and author of the multimedia resource How to Assess While You Teach Math: Formative Assessment Practices and Lessons, Grades K–2. Overview of Lesson This lesson is…

View Lesson

Grades 4–5

Leftovers with 100

Maryann Wickett and Marilyn Burns's new book, Teaching Arithmetic: Lessons for Extending Division, Grades 4–5 (Math Solutions Publications, 2003), builds on the concepts and skills presented in Teaching Arithmetic: Lessons for Introducing Division, Grades 3–4 (Math Solutions Publications, 2002). Their new book helps students calculate with multidigit divisors and dividends (using a method that makes sense to them!) and also deepens students' understanding of divisibility, relationships between dividends and divisors, and the meanings of remainders. The lesson presented below teaches students a game that reinforces all of these goals. (Note: Other lessons in the book address speciﬁcally how to teach long-division skills. Also, if you are familiar with The Game of Leftovers from the ﬁrst division book, you'll see here how to extend that game for older students.)

"Today I'm going to teach you Leftovers with 100," I began the lesson. "To show you how to play, I need a volunteer."

I chose Skylar and, as he walked to the front of the room, I wrote on the board:

I explained as I pointed to what I'd written on the board, "When you play, you and your partner will make one recording sheet that looks like this. Be sure to write both your names on your recording sheet."

"This time I'm going to go ﬁrst to show what to do," I continued. "Something important to remember is at the end of the game, both players add their remainders, and the player with the larger sum wins. The ﬁrst player starts with a start number, or dividend, of one hundred. Because I'm the ﬁrst player, I start with one hundred and I have to choose a divisor from the numbers listed from one to twenty. Then I'll divide one hundred by the divisor I chose, and Skylar will record on our sheet. What divisor would be a good choice?"

Lucas explained, "If you want to win, then you have to choose a divisor that will give a big remainder."

"What would be a bad choice?" I asked.

Lupe said, "One would be bad because one goes into all numbers with no remainder."

"Ten would be bad," Alberto said. "Ten time ten equals one hundred, so there wouldn't be any remainder."

"What number on the list would make a good choice?" I asked.

"Three," Derek said. "With three there would be a remainder of one because if you count by threes, you land on ninety-nine, and that's one away from one hundred."

"Nineteen," Zoe said. "It would have a remainder of ﬁve. Five times twenty is one hundred. Nineteen is one less than twenty, so I think ﬁve times nineteen would be ninety-ﬁve. One hundred minus ninety-ﬁve is ﬁve and that ﬁve would be left over."

I recorded Zoe's thinking on the board:

5 x 20 = 100

5 x 19 = 95

100 – 95 = 5

"I like Zoe's idea of using nineteen as the divisor," I said. "Since it's my turn to pick a divisor and divide, Skylar will record on our recording sheet, 'One hundred divided by nineteen equals ﬁve remainder ﬁve.' Circle the remainder and write my initial since it's my turn." Skylar recorded:

I continued, "Before Skylar can have his turn, we have to do two things. He has to cross off nineteen from the list of divisors. We only get to use each number listed once in a game." Skylar crossed off the 19. "Then we have to subtract my remainder of ﬁve from my start number of one hundred to create a new start number for Skylar to use."

Skylar told me his start number was ninety-ﬁve, and the class agreed.

"Since it's Skylar's turn, I record," I said. I wrote 95 under the Start Number column. Skylar looked overwhelmed, so I called on Kenzie for advice.

"If you use twenty as the divisor, then you can get a remainder of ﬁfteen. Four times twenty is eighty, and ninety-ﬁve minus eighty is ﬁfteen."

Skylar told me to write 95 ÷ 20 = 4 R15. He reminded me to circle the remainder of 15, to put his initial beside the problem, and to cross off 20. I did.

Joey said, "Remember to subtract the remainder from ninety-ﬁve. Ninety-ﬁve minus ﬁfteen is eighty."

"I agree," I responded. "Since it's my turn, Skylar gets to record." He recorded 80 in the Start Number column.

Joanna suggested I use eighteen. She explained, "Ten times four is forty. Eight times four is thirty-two. So forty and thirty-two make seventy-two."

"I'll use your suggestion of eighteen," I said. Skylar recorded on the board:

"What's the score so far?" I asked.

Johanna said, "Skylar has ﬁfteen and you have thirteen."

I asked how to create the next starting number, and Kylie explained, "You subtract the remainder from the last starting number. Eighty minus eight is seventy-two."

Skylar decided to use eleven as his divisor. He said, "My dividend is seventy-two. So seventy-two divided by eleven is six with a remainder of six. I get a score of six."

I recorded Skylar's turn on the board:

"Sixty-six would be the next starting number," Korina said, "because seventy-two minus six is sixty-six." I agreed.

The students seemed to understand how to play and I wanted to give them ﬁ rsthand experience with the game, so I said, "The game ends when the start number is zero, but Skylar and I are going to stop here so that you can play. To ﬁgure out who is the winner, add the remainders."

"Skylar has twenty-one and you only have thirteen," Kerri said.

"I agree," I said and wrote our scores under our recording to model for the students how to record their scores when they played:

"Why don't you get started playing with your partners now?" I said, and I left the recording of the game between Skylar and me on the board as a model for the students.

Observing the Students

After a few minutes, I noticed Sean and Lucas were involved in an intense discussion. Sean asked me, "Is it possible to have a remainder as big as ﬁfteen? I don't think it's possible, but Lucas does."

Lucas said, "I think any number can be a remainder. What can be a remainder depends on the divisor."

"What's the divisor in the problem you're discussing?" I asked.

"It's nineteen," Lucas said. "I think as long as the remainder is smaller than the divisor, it's OK because there isn't enough for another group."

I replied, "If the divisor is nineteen, then it's possible to have a remainder of ﬁfteen."

I noticed as I continued to circulate that Skylar and Sasha showed how they did the dividing and, after two rounds, changed their way of recording their work.

Figure 2. Skylar and Sasha changed how they recorded the game but showed their thinking clearly. While Sasha made an error when he multiplied 12 x 6, it didn't affect the next start number.

Elli and Lupe recorded their division using another method.

Figure 3. Lupe made an error when she divided 40 by 11. However, it didn't affect the next start number. Elli used scratch paper to show how she ﬁgured her answers.

After the students had played for a while, I asked for their attention.

A Class Discussion

"What did you think about the game Leftovers with 100?" I asked.

Zoe said,"Elli and I discovered something. At the end, you can get a lot of leftovers once the starting number gets below twenty. We got to thirteen as the starting number, and fourteen was left as a divisor, so I took fourteen and got all thirteen because thirteen divided by fourteen is zero with a remainder of thirteen."

Kenzie said, "Beth and I ﬁgured that out, too, but not until the starting number was ﬁ ve. Then Beth ﬁgured out she could divide ﬁve by six and the answer would be zero remainder ﬁve, and she got the ﬁve."

Beth said, "I have a question. Everett and Derek added up all their leftovers together and it came out to one hundred. When Kenzie and I added ours up, it was only eighty-eight. I don't get it."

I asked the class, "Why does it make sense that the total of both players' remainders should total one hundred if you ﬁnish the game?"

Niki said, "If you start with one hundred, and you keep dividing until you get to zero, then the remainders have to add up to one hundred because the only way to make the starting numbers go down is to subtract out the remainders."

"Oh yeah!" replied several students.

I suggested to Beth and Kenzie that it was possible they had made an error somewhere and perhaps they needed to go back and check their work. They went to the back of the room to check their work together, and after a few moments blurted out, "We found the mistake."

They reported on the subtraction and division errors they had made and commented on what they had discovered. "We discovered that if you look at the divisors that haven't been used and try to think of a multiple of one of the divisors that's just a little bigger than your dividend, or starting number, you can get a big remainder," explained Beth. "For example, if your dividend is ﬁfteen and eight is a divisor you could still choose, it would be a good choice because the multiples of eight go eight, sixteen, and sixteen is just one bigger than ﬁfteen, so there should be a big remainder. There would be a remainder of seven for that problem."

"We also noticed that the new start number is always the same as the whole number part of the previous quotient times the previous divisor," Kenzie shared. "If you look at the board, you can tell. Look where it says eighty divided by eighteen equals four remainder eight. Four times eighteen is seventy-two, which is the next start number. In the top problem where it says one hundred divided by nineteen equals ﬁve remainder ﬁve, ﬁve times nineteen is ninety-ﬁve, which is the new start number."

There were no other comments. Over the next several days, children continued to play when they had free time. It was wonderful to see them so happily engaged while getting practice with division.

Related Publication:
Teaching Arithmetic: Lessons for Introducing Division, Grades 3–4
by Maryann Wickett, Susan Ohanian, and Marilyn Burns

From Online Newsletter Issue Number 12, Winter 2003–2004

View Lesson

Grades 4–5

Dividing Brownies

In this excerpted lesson, students divide "brownies" into halves by using either a spatial approach (focusing on the shapes of the fractional parts) or a numerical approach (calculating the number of square units in the shapes they create). In the full-length lesson of Dividing Brownies, which appears in Marilyn Burns's Teaching Arithmetic: Lessons for Extending Fractions, Grade 5 (Math Solutions Publications, 2003), students continue with the investigation, dividing brownies into fourths and eighths.

Before class began, I drew on the board six 4-by-4 square grids like the ones on the worksheet the students would be using.

I asked, "Let's see if we can figure out how to divide each of these grids in half in a different way. Think of each as a brownie that you'll cut so that two people would each get the same amount to eat." I called on Daniel.

"Just draw a line down the middle from the top to the bottom," Daniel said.

"Do you mean a vertical line like this?" I asked, using the word vertical to reinforce this terminology as I drew a line. Daniel nodded "yes."

"That's what I was thinking," Ali said.

"I know another way," Eli said. "Draw the line across the middle."

"Do you mean a horizontal line, like this?" I asked, drawing on the second grid.

"That's it," Eli said.

"Another way?" I asked. I called on Katia.

"Make a slanty line. Start on the top, on the left, and then come down to the bottom on the right," she said.

"Do you mean a diagonal line, like this?" I asked, drawing on the third grid. Katia nodded.

"Another way?" I asked.

"Draw a diagonal line like Katia's, but in the other direction, from the top right down to the bottom left," Sophia said.

I did so on the fourth grid.

"Another way?" I asked.

The class was silent.

I waited a bit more and then said, "Try to think of ways that don't use one straight cut but that still cut the brownie into two pieces that are the same size. Talk at your tables and see what you can come up with."

The noise level in the class rose as students began to talk. Some got out pencils and paper to sketch. It's typical for a class to get stuck after these first four suggestions, but I find that if I give students time to talk together, they typically come up with other ways that work. After a few moments, I called the class back to attention. Lots of hands were raised. I called on Andrew.

He said, "This is really hard to explain. Can I come up and draw it?" I agreed, so he came up to the board and drew his idea.

"How do you know for sure that the two pieces are halves?" I asked.

"They both have eight squares in them," Andrew said, dividing the grid into squares to show what he meant. "See, the whole thing is sixteen, so eight is half."

I turned to the class. "Thumbs up if you understand what Andrew said, thumbs down if you're not sure." Carolyn and Lindsay had thumbs down, so Andrew explained again.

"See, there are sixteen small squares," he said, counting them one by one to prove this. "So eight is half because eight plus eight is sixteen." Carolyn and Lindsay were both satisfied.

Emmy added, "You can see they're both the same. One is kind of upside down from the other."

"Yes, I can see how you could rotate one and it would fit exactly on the other," I said and then asked, "Who would like to divide the last brownie into halves in another way?" I called on Maria. She came up with her paper and drew on the last grid.

"I checked like Andrew did," she explained. "They're both eight."

"Thumbs up if you agree, thumbs down if you want to challenge or would like more explanation," I said. Everyone had a thumb up.

I then asked the class to look again at the brownies cut with diagonal lines, as Katia and Sophia had suggested. "Can you count the squares on these to be sure that each half is worth eight squares?" I asked. I wanted the students to think about counting and combining halves of squares. A buzz broke out in the class and I waited a moment before asking the students for their attention. Then I called on Claudia. She came up to the board and explained, using Katia's brownie.

"See, there are six whole squares," she said, drawing lines and marking Xsto show them on the bottom diagonal half. "Then these two halves make one more, and that's seven, and two more halves make eight." She looked at me.

I looked at the class and asked, "Questions?"

"Could you do that again?" Carolyn asked.

"OK," Claudia said, and repeated her explanation. Carolyn was satisfied and there were no other questions.

I then told the students what they were to do next. I showed them the worksheet of grids and said, "I'm going to give each of you one of these. Look for ways to divide each brownie on the worksheet in half in a different way. For each brownie you divide, be sure that you can explain how you know that the two pieces really are halves."

"Can we work together?" Melissa wanted to know.

"Yes," I said, "but you each must record on your own paper."

"Can we use the ones on the board?" Eli asked.

"No," I said, "see if you can find others."

"I think it will be hard to fill the sheet," Nick said.

"Just see how many you can find," I said.

The students got to work. As I circulated, I noticed that some students relied on counting squares before drawing while others drew, then counted, and made corrections if necessary. As they worked, I erased the brownies we had divided on the board and drew six blank grids for a class discussion. When about ten minutes remained in the period, I called the class to attention. No one had completed the entire page, but they had done enough to make me feel confident that they understood the assignment. In the last few minutes of class, I had six students come to the board, divide one of the blank grids in half, and explain to the class how they had divided the grid into two equal pieces.

From Online Newsletter Issue Number 11, Fall 2003

Related Publication:
Teaching Arithmetic: Lessons for Extending Fractions, Grade 5
by Marilyn Burns

View Lesson

Grades 4–5

Math Homework that Counts

Homework: If 3 is 5% of a number, what is 30% of that number?

This assignment, at ﬁrst glance, doesn't seem very difﬁcult. But when I asked my sixth graders to solve this problem for homework, I also asked them to take notes about how they solved it. When we shared our approaches, I was convinced that the rote teaching of the three methods of solving percent problems is far less satisfactory than developing students' wider sense of number.

Deema said, "I found out that one percent of the number is three divided by ﬁve, since three is ﬁve percent. The answer is three ﬁfths, which I know is point six. Since I want thirty percent, I just multiply point six by thirty, and I get eighteen. The answer is eighteen." I wrote what she said in shorthand on the board:

Find 1% and then x 5 by 30.

Joan said, "I knew that there were six ﬁves in thirty, so there must be six threes in the number. That number is eighteen." I wrote:

5 x 6 = 30 so 3 x 6 = the number we're looking for.

Simone said, "I made two ratios. The ﬁrst was to ﬁnd the whole number. I wrote three over n equals ﬁve over one hundred. Then I cross-multiplied, and I knew that ﬁve n is the same as three hundred, so the whole number is sixty. Then I wrote n over sixty is the same as thirty over one hundred to see what thirty percent of the whole number would be. I got eighteen also."

Charlotte said, "If three is ﬁve percent of a number then six must be ten percent of the number and if six is ten percent, then three times six, or eighteen, is thirty percent of the number."

"Wow, that makes so much sense!" said John, amazed by Charlotte's simple explanation.

"So, John," I asked, "how did you solve this problem?"

"Well, my dad always says to draw a picture, and that's a good idea, but I don't always know what picture to draw."

"Let's see if we can help," I suggested. I drew a big rectangle and divided it into ten long thin rectangles. "Each of these is ten percent."

Sam chimed in, "Just cut them in half, and then each will be ﬁve percent."

I colored one twentieth in and labeled it ﬁve percent. John got excited. "I know, you count by ﬁves and color one of those sections in each time until you get to thirty. Let's see, you'd color in six of them. That's sixty percent."

"No," said Mike, correcting John, "I thought about it that way too, but without the picture. I said that ﬁve percent is one twentieth and twenty times three is sixty, so that means sixty is the whole number. Finding thirty percent of sixty is eighteen."

"Wait," said John. "What does that have to do with my picture? I didn't have to ﬁgure out the whole number, I just know that I colored in six blocks and each one is worth three. Oh yeah, that means eighteen is thirty percent. I agree with Mike."

I used to worry about spending so much class time discussing a homework assignment. However, as the TIMSS' (1996–98) results suggest, what we really want is thorough understanding as opposed to an acquaintance with a wide variety of topics.

From Printed Newsletter Issue Number 28, Fall/Winter 2000–2001

Related Publication:
Math Homework That Counts, Grades 4-6
by Annette Raphel

View Lesson

Grades 4–5

Factor Game

Playing the Factor Game provides an engaging format in which students can become familiar with the factors of numbers from two to thirty by playing a two-person board game. To play Factor Game, each player chooses a number while the other player finds the sum of the available factors of that number.

While playing this wonderfully simple and effective game,

children quickly realize that prime numbers are poor choices after the first move of the game, although they may not be able to define the numbers as such . . . yet;
realize that larger numbers are not necessarily the best choices;

learn that some numbers are overwhelming choices because they have so many factors;
understand that some odd numbers are good choices and even numbers can be poor choices; and
above all, how their ability to factor a number relates to their understanding of multiplication and division.

This investigation offers students opportunities to:

review multiplication and division facts
relate dividing and finding factors of numbers
classify numbers as prime or composite
recognize that some numbers are rich in factors while others have few
recognize that some products are the result of more than one factor pair
identify and articulate the relationship between factors and multiples

Duration

2–3 class periods and played throughout the unit or year

Materials

Factor Game game boards (see reproducible at the end of this lesson), 1 overhead copy and 1 copy per pair of students
Factor Game directions (see reproducible at the end of this lesson)
2 colored pencils (a different color for each player)

Vocabulary

factor, factor pair, multiple, product

Lesson Outline

Focus or Warm-Up

1. My method of introducing the Factor Game to my class is not standard. Many texts suggest that you discuss the term factor and how it relates to this game. Although this works, I wondered if the children could identify the relationships between the numbers chosen and the points scored without telling them the objective of the game, which would allow them to identify the game's purpose. I purposefully introduced the game on the overhead with no instructions other than we would be keeping score and the team with the most points would "win." (The actual instructions are referenced in the reproducibles following this lesson for your preparation.) The title of the game board was available to the children, but they paid no attention to it.

2. I constructed a T-chart on the board to keep track of the points scored.

Introduction

3. I told the children that I would go first . . . after all, I was the teacher! I told them that I was choosing 29 and earned those 29 points. I crossed 29 off on the game board. The, I told them that they would receive 1 point as the result of my choice and crossed off 1. Now it was their turn to choose.

4.The class worked together to choose a number—and invariably chose 30. After all, it was the largest number on the board! I crossed off 30 and posted it on their side of the T-chart while keeping a running total. The class now had 31 points. I deliberately thought out loud as I calculated my points. "Let's see—I get six and five, two and fifteen, oh yeah, and three and ten. That gives me a total of forty-one points!" Tortured cries were heard from the class. As I was thinking out loud, the children were buzzing about how I was earning my points—and how they were losing theirs!

5.I then chose 25—knowing that the children had to earn points for at least one factor in order for the move to be "legal." So I posted 25 and asked the class for their choice. Animated mathematical conversation erupted. Some children were aware of some of the rules of the game at this point. They realized that when one player chose a number, the other player earned points related to the numbers multiplied together to get that particular number.

The language of factors, multiples, and products was not yet being used, but that was fine at this point in the game. When I first began to play the game in this manner, I was astounded at the inefficiency of the discussion accompanying the game without the availability of this terminology. What a great lesson to learn about the power of mathematical language!

6. As we played one or two more rounds, I began to share a few of the rules—the first being that when you choose a number, the other player must be able to earn points. If the other player can earn no points from your choice, you lose your turn. The language of prime and composite had not yet been introduced, but the children quickly learned that they needed to stay away from prime numbers after that first move because they could not earn any points on the resulting move.

7. After several rounds, I introduced language that would be helpful as the children discussed potential moves. As words were discussed, I wrote them on the board for accessibility. The class was familiar with the term product, but not at ease with its application in their casual mathematical conversations. Walk-by interventions, as I call them, are crucial early in the school year. If I hear children playing and referring to "answers" in multiplication problems, a hand on the shoulder and a reminder to use the work product helps to focus the mathematical conversation, making it more efficient and concise. The game title identified the new term factor and its meaning in reference to the game being played. You can also introduce multiple, but be prepared for its misuse. Because of the newness of the language, many fifth graders will interchange factor and multiple. They will often use factor correctly in isolation, but run into difficulty when asked to construct a sentence with both factor and multiple. Please refer to the "Reading, Writing, and Vocabulary" section of Chapter 2 in Enriching Your Math Curriculum, Grade 5 for further discussion of the scaffolding for appropriate use of these terms.

An entire class period was devoted to this introduction of the Factor Game. I was delighted with the mathematical observations, insights, and discussions that occurred within this format. The language of factors, multiples, and products was immediately meaningful because it supported the children as they discussed and analyzed their number choices. They also learned an important lesson about the importance of implementing appropriate mathematical language. Kira reminded us several times that it was just plain easier to use the word product than the phrase ". . . the answer to a multiplication problem."

Exploration

8. Once the initial games are played, the children can set off with partners to play a game or two on their own. As the children play the game, circulate through the room, making note of interesting strategies. You may also want to note who continues to struggle with the recall of their basic multiplication facts. A lack of fluency with the multiplication tables can make playing this game difficult and tedious. As you move around the room, you may wish to visit some of the pairs and ask them the following questions:

Is it better to have the first move when you start the game? Why?
What is the best first move? Why?
What is the worst first move?
How do you know when the game is over?
How do you know when you have found all the factors of a number?

Summary

9. Pulling the class together for a processing session is important and necessary after the children have had the opportunity to play several rounds of the game. Processing the game gives mathematical meaning to the activity. The children need to realize that although games can be great fun, as this one certainly is, good mathematical games also have purpose.

Crafting, asking, and answering good questions can further the mathematical understanding of just about any activity. Good questions can set the stage for meaningful classroom discussion and learning. Students are no longer passive receivers of information when they asked questions that deepen and challenge their mathematical understandings and convictions. Good questions

help students to make sense of the mathematics;
are open-ended, whether in answer or approach;
empower students to unravel their misconceptions;
not only require the application of facts and procedures, but encourage children to make connections and generalizations
are accessible to all students in their language;
lead children to wonder more about a topic. (Good Questions, Schuster and Canavan Anderson 2006)

Questions such as those that follow can help to scaffold and articulate new understandings that have come about as a result of playing the Factor Game. Processing questions in a whole-class format also gives you the opportunity to implement talk moves. Asking the children to restate classmates' ideas or strategies can help to keep them focused on the mathematics of the game, while asking children to add on to others' ideas can deepen insights and observations. You can help to establish respectful discourse by asking for agreement or disagreement. Revoicing can emphasize important mathematics, insights, or strategies.

Is it better to go first or second? Why?
What is the best first move?
What is the worst first move? Why?
How do you know when you have found all the factors of a number?
How do you know when the game is over?
Is there a way to finish the game with all the numbers circled on the game board?
What was your strategy for choosing numbers?
After the first round, what types of numbers did you stay away from? Why?

Follow-Up Lessons

You can have follow-up lessons that draw upon the understandings constructed from the Factor Game. Lessons exploring prime and composite numbers, odd and even numbers, and square numbers take on greater meaning because of the children's exposure to and application of these concepts while playing the Factor Game. My class explores perfect, abundant, and deficient numbers as well because of the connections they can make to number choices on the Factor Game game board. Exploring and applying divisibility rules also now have a place and purpose in the curriculum. Being mathematically proficient goes far beyond being able to compute accurately and proficiently. It involves understanding and applying various relationships, properties, and procedures associated with number concepts (Math Matters, Chapin and Johnson 2006). The Factor Game and the lessons that it subsequently supports can do just that.

Reproducible

The Factor Game Directions

You need:

a partner
colored pencils in two different colors
The Factor Game game board for 30 (see the next reproducible)

Directions

1.Player A chooses a number on the game board and circles it. This will be Partner A's score for that round.
2.Using a different color, Partner B circles all the proper factors of Player A's number. The proper factors of a number are all the factors of that number except the number itself. Partner B lists the factors. The sum of those factors is Partner B's score for that round.
3.Player B then circles a new number. Player A circles all the remaining factors of that number. Then, play continues in this manner.
4.The players take turns choosing numbers and circling factors.
5.If a player circles a number that has no factors left which have not been circled, then that player does not get points for the number circles and loses his or her turn.
6.The game ends when there are no more numbers left with uncircled factors.
7.The player with the larger sum of factors and products is the winner.

Extension: Play a game on a 49 game board! (See the corresponding reproducible.)

The Factor Game is referenced in various publications:

About Teaching Mathematics (Burns 2007)
Prime Time (Connected Mathematics 2) (Lappan, Fey, Fitzgerald, Friel, and Phillips 2006)

A similar game called Factor Captor is referenced in: Everyday Mathematics: The University of Chicago School Mathematics Project, Grade 5 (Everyday Learning Corporation 2002).

Reproducible

The Factor Game Game Board for 30

Reproducible

The Factor Game Game Board for 49

Featured in Math Solutions Online Newsletter, Issue 36

Related Publication:
Teaching Arithmetic: Lessons for First Grade
by Stephanie Sheffield

View Lesson

Grades 4–5

A Measurement Problem

For this lesson, I planned to have the students work individually to solve a measurement problem involving fractions. Before the period began, I drew on the chalkboard a line segment that measured 22 1/2 inches. Also, I put thirty-three Uniﬁx cubes into a plastic bag.

"When I snap these cubes together," I said, "do you think the train will be longer, shorter, or about the same length as the line segment I drew?" The students had no way of making a reasonable prediction by looking at the bag of cubes, but they were willing to guess.

After all who wanted to had voiced an opinion, I asked, "How could we ﬁnd out?"

"Do it!" they answered in unison.

Two students snapped the cubes together. They matched the train to the line segment and found that the train was about 2 inches longer than the line segment. We discussed what "about the same length" means when measuring. We had a lively conversation about when it was necessary to be accurate (building shelves for a bookcase, measuring fabric for a dress, or timing a soft-boiled egg) and when approximations would sufﬁce (cutting paper and ribbon to wrap a gift, measuring water for cooking spaghetti, or dishing out equal portions of mashed potatoes).

Then I asked them to make a different estimate. "How long do you think the line segment is?" I asked.

"It's shorter than the yardstick," Mark said. The yardstick was resting on the chalkboard tray.

"Maybe thirty inches," Marcie said.

"How many cubes long is it?" Peter asked. Peter's question led us in the direction I had planned.

I held the train up to the line segment and removed three cubes so that their lengths matched. Then I split the train into tens. There were thirty cubes in all.

"So the line segment is as long as a train of thirty cubes," I said. "Can that information help you ﬁgure out the length of the line segment?"

"How big are the cubes?" Amy asked.

"They're three-quarters of an inch on each side," I said. "What else do you need to know?"

There were no more questions. I then said, "I'd like you to ﬁgure out how long the line segment is. When you record your answer, be sure to explain why it makes sense." The students got to work.

The room became quiet with the kind of quiet that test taking often produces. Some students started to write about their ideas; some did calculations on their papers; others gazed into the distance, apparently thinking.

The students' papers gave me much to think about. Scott's paper was representative of many of the students who couldn't make any headway. He wrote: I have not ﬁgured this out because I don't know how. I'm stuck!

Some students made some progress, but ran into snags. Jonathan, for example, wrote:

³⁄x • 30 =

. First I multiplied

x 30. I think this is a good way of doing this because all you have to do is multiply the numbers and you have your answer. Jonathan didn't look at the line segment on the chalkboard to notice that an answer of less than 10 inches made no sense.

Karine came up with an interesting beginning. She wrote: I know its less than 30 inches because the cubes are smaller than 1 inch. Its more than 15 because that would be half and is more. She was then stumped and had no place to turn.

Mark made a good start but then took a false turn. He wrote: Two cubes make 1
inch. 4 cubes make 3 inches. So 8 x 3 makes 24 inches.

Jessica was one of three students for whom the problem was easy, even trivial. She wrote:

22½ I multiplied ³⁄x •30. 30 is equal to 30/1. I multiplied and then I reduced to get my answer. It makes sense because you're doing 30 times. It's easy to multiply it.

What I had done was put the students in a testing situation, not a learning situation. Dealing with fractions is difﬁcult for many students, and they need as much support as possible to learn about them. By having students struggle individually, I didn't provide any way for them to get feedback on their thinking or hear about other students' approaches. And when they are working individually, there's no way that I can get around to help all of them.

Cathy Humphreys presented the same problem to seventh graders. She introduced it as I had. However, rather than have the students solve the problem and write individually, she had them work in groups of four. That way, the students could talk with one another and draw from their collective thinking.

To promote further communication in the class, Cathy gave each group an overhead transparency and marker. "Record your solutions and your thinking on the transparency," she said. "Then each group will present its thinking."

The groups' interactions were animated and their explanations revealed that the students used a variety of approaches. Group 6 wrote:

22½ because we know that each cube equals ¾ inches. We rounded ¾ to 1 whole inch. Then we multiply 30, because there is 30 cubes by 1, which equals to 30. We drew ten sticks. 1 inch equals to 4/4 and so we need more to make 1 inch. Four of ¼ = 1 inch out of 10 sticks it equals to 2½. If we do that for 3 times it equals to 7½. We subtract 30 by 7½ which equals to 22½. (See Figure 1.)

Figure 1. Group 6 figured the length of the train if the cubes were 1 inch long and then adjusted. (Grade 7)

Group 3, however, used both decimals and fractions to ﬁgure out the problem. They wrote:

1.00 =4/4 so 3/4= .75. so we multiplied .75 x 30 = 22.5 which is 22.5 which is 22½ inches. Our answer is 22½ inches. Another way we ﬁgured it out was 30 x 3 ÷ 4 = 22.5. (See Figure 2.)

Figure 2. Group 3 used a combination of fractions and decimals. (Grade 7)

Group 1 wrote: The total inches are 22.5. We think its 22.5 because each cube is of an inch and their is 30 cubes so you split one each into 4 parts and you times 30 cubes by 3. And you get 90. And then divide 90 by 4. They showed how they did the calculation.

Group 7 had a different approach. They wrote: The answer is 22½. Our group ﬁgured it out by putting two cubes together. Two cubes = 1½ inches.

Then we multiplied 1½ by 15 because we used 2 cubes to make 1½ we cut 30 in half which = 15. That's how we got our answer.

Figure 3. Group 5 gave two solutions, first figuring the length of six cubes and then figuring the length of two cubes. (Grade 7)

From Online Newsletter Issue Number 5, Spring 2002

Related Publication:
Writing in Math Class: A Resource for Grades 2–8
by Marilyn Burns

View Lesson

Grades 4–5

A Fifth-Grade Fractions Lesson

In order to introduce my students to problems that involve division with fractions, I use problem situations that draw on familiar contexts. I keep the focus of their work on making sense of the situation and explaining their strategies and solutions. Some of the problems I use don't require that students necessarily arrive at a precise answer, but rather allow them to use the information at hand to arrive at a correct solution. In this way, the students' experiences mirror how we often encounter problems in real life.

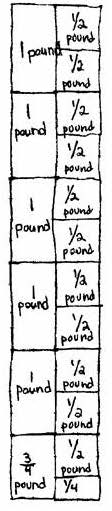
For example, I gave my fifth graders the following problem:

I purchased one giant 5 3/4-pound bag of DAJM (Swedish chocolates). I want to separate the candy into 1/2-pound bags to give to my fifth-grade students. How many 1/2-pound bags can I make? Will there be enough for each person in the class? If not, how much more will I need to buy?

By dividing wholes into halves, Jamie solved the problem.

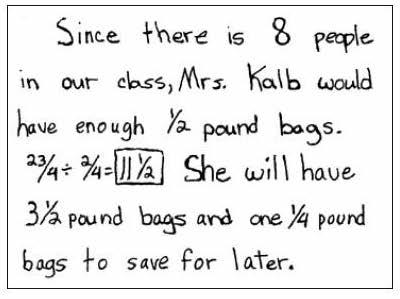
Randy also solved the problem by drawing a picture.

After students shared their answers and the methods they used, I gave them other problems to solve, using other amounts for the sizes of the large and small bags. I didn't write the chocolate scenario over and over again but presented the situations only with division problems. This helped students connect the original situation to the correct mathematical representation. The problems they solved included the following: 2 2/3 ÷ 1/3 = ? 1 1/2 ÷ 1/4 = ? 1 1/2 ÷ 1/6 = ? 2 5/6 ÷ 1/3 = ? As before, the students were asked to explain the methods they used. The next day, I asked the students to write a word problem to match one of two equations: 1 3/4 ÷ 1/2 = ? or 4 2/5 ÷ 1/3 = ? The problems they wrote helped me assess their ability to connect an equation involving division with fractions to a real-world context.

Here is just a sampling of the problems they wrote:

Tom was buying wood for his woodshop class. Each student needed 1/2 foot of wood to complete his or her project. If Tom buys 1 3/4 feet of wood, how many students can complete their projects? How much wood is left over?

Matt has 4 2/5 candy bars. (Each candy bar has five equal parts.) Matt's group of friends wants 1/3 of a candy bar each. How many friends can Matt give 1/3 of a candy bar to?

Betty went to the local fabric store for fabric to make curtains. She bought 4 2/5 of a yard. If she needs 1/3 yard to make one curtain, how many curtains could she make? How much fabric is left over?

The students shared their word problems, resulting in some very interesting discussions. After hearing the problem about Betty buying fabric for curtains, for example, I pointed out that if I went to buy fabric to make curtains, I would measure and know ahead of time how much fabric to buy and how many curtains I would be making. However, if I happened to be in the fabric store looking at remnant pieces and found one I liked that was 4 2/5 yards long, I might consider what I could make with it— pillows, aprons, etc.

To follow this experience, I next gave them the assignment to solve the Pinewood Derby problem:

Charles makes Pinewood Derby kits from 8-foot stock. Each model requires a piece of stock that is 7 3/8 inches long. Each cut consumes an additional 1/16 inch of material. How many 8-foot pieces of stock are required to fill an order for 500 kits?

The students understood that they needed to add 7 3/8 to 1/16, and they correctly did that, understanding that one kit needed exactly 7 7/16 inches of wood. After that, no one knew what to do next. I encouraged them to make a model. First they cut out a strip from used file folders that measured 7 7/16 inches long. Then we measured and marked with masking tape 8 feet (or 96 inches) on the classroom floor. At this point, the students were off and running. They carefully measured out 12 pieces of 7 7/16 inches each from 96 inches and then went back to their desks and finished the calculations.

Here is how one student expressed her thinking in writing.

View Lesson

Grades 4–5

Millions

For almost two months before David Schwartz's visit, our school took on the challenge of collecting 1,000,000 pennies.All of the considerations, from storing to rolling them, were an interesting challenge. In seven weeks, we collected 250,000 pennies, and we plan to continue at least until the end of the year to see how close we get to 1,000,000.

When students bring in the pennies, they toss them into a tub that is about the size of a file drawer. At first, when the tub was not even quite full, some made the comments, "We must be close." "Whoa! That must be a million pennies." After some investigation, however, we estimated that a full tub would hold about 30,000 pennies. Then we figured out that we needed more than thirty tubs of pennies to make 1,000,000. That shocked them — and me, too! In preparation for David Schwartz's visit, all of the classes in the school did a variety of large-number projects, some inspired by his book The Magic of a Million Activity Book (Scholastic, 1999). I created an open-ended activity to do with my class:

"If one million fifth graders . . . , they would . . . ." The assignment for the students was to come up with a situation involving a million fifth graders and then solve the mathematics necessary to describe the situation.

The students' examples included the following:

If one million fifth graders each bought a Big Grab Bag of Hot Cheetohs, the Cheetohs would completely fill three of our very high ceiling classrooms that are about 10m-by-10m-by-4.8m.

If one million fifth graders lined up fifteen feet apart and passed a football from one end of the line to the other, the ball could travel from Merced, California, to Antarctica!

If one million fifth graders each ate a paper plate of lasagna and threw the plates away, the garbage would weigh as much as three blue whales and would fill a hole that is seventy-three cubic feet.

As you can see, we've had a lot of fun with this project!

From Online Newsletter Issue Number 9, Spring 2003

View Lesson

Grades 4–5

French Fries

Before I began this lesson, I checked with a local hamburger restaurant and learned that there are about forty french fries in a single serving. I asked the class, "If a single serving of french fries has forty fries, how many friends would one thousand french fries feed?" I gave them a few minutes to think and then asked for their ideas.

"You could divide forty into one thousand," Tina began.

Mia said, "Some people might not be able to divide by double digits. So you could take a zero away from the forty to make four and a zero away from the one thousand and make it one hundred and then figure out how many fours in one hundred."

I doubted that others, or even Mia, knew why her idea made sense, so I asked, "How many fours are there in one hundred?"

"Twenty-five," Carol said.

"Is that the answer to dividing forty into one thousand?" I asked Tina.

She shrugged. "I'm not sure. I didn't figure it out yet."

I knew that by removing a zero from both the 40 and the 1,000, Mia made a more manageable problem that was proportional to the original problem and, therefore, would produce the same answer. But this is a difficult concept for students to grasp. I recorded on the board:

1,000 ÷ 40 = 100 ÷ 4

100 ÷ 4 = 25

"Who has another idea?" I asked. "Maybe we can check if twenty-five is right."

Abdul raised his hand. "I thought that there were five forties in two hundred. You can count forty, eighty, one hundred twenty, one hundred sixty, two hundred, so that's five. There are five two hundreds in one thousand. Two hundred, four hundred, six hundred, eight hundred, one thousand, that's five two hundreds. So I think you could multiply five by five and that would make twenty-five servings." I recorded Abdul's thinking on the board.

5 40s in 200

40, 80, 120, 160, 200

5 200s in 1,000

200, 400, 600, 800, 1,000

5 x 5 = 25 (The first 5 is the number of 40s in 200 and the second 5 is the number of 200s in 1,000.)

"Are there any questions about how Abdul solved this problem?" I asked the students.

"I don't get it at all!" Mark said.

"Do you understand where the first five comes from?" I asked as I pointed to 5 x 5 = 25.

"I'm not sure," Mark said.

"Count by forties to two hundred," I suggested. Mark did. "How many forties did you count?" I asked.

"Oh, I see, there were five forties and there are five groups of two hundred in one thousand. I get it now!" Mark said.

"Does someone have another way?" I continued.

"You can use division," Jim said. He came to the board and wrote:

4√1000

Then he used the standard algorithm to figure out the answer.

"Why did you use division?" I asked.

"Well you are trying to figure out equal groups of forty, I think, and how many groups of forty there would be in one thousand. I used one thousand because that's the number of french fries and I used forty because that's how many are in each group," Jim explained.

"What do you notice about Jim and Abdul's work?" I asked the class.

"One used multiplication and the other did division and they got the same answer," Carol replied.

"I can use multiplication to prove my answer is correct," Jim added. "If I multiply forty by twenty-five I'll get one thousand. This proves my answer is right."

"I think they're both right. They just thought about it a little differently. They got the same answer," Becky said.

I then wrote a different problem on the board: Sam's Burgers sells fries with 52 fries per bag. How many fries would be needed if everyone in our class ordered one bag of fries?

I explained to the students what they were to do. "You'll each work independently to solve this problem. You may use any of the ideas on the board or that you have heard before that you think would help you solve this problem. You may also use your own ideas. Please be sure to show me your thinking clearly using words, pictures, and numbers." The students got to work. I circulated and gave help as needed.

Later we had a discussion about the answer and the methods they used. Some children used the standard algorithm and I asked them to show me a second way they could solve the problem. Many made use of finding partial products in a nonstandard way. Having children work on an assignment like this during class gives me the opportunity to check on children's understanding and evaluate if I need to redirect their thinking.

A student's reasoning on how many fries total will be in everyone's bag, if there are fifty fries per order. | Math Solutions, Classroom Lessons

Carol used partial products to solve 52 x 24.

A student uses small addition to answer the question of how many fries will there be, if each order has fifty-two fries, and twenty-four students. | Math Solutions, Classroom Lessons

Josh also made use of finding partial products to solve the problem.

Related Publication:
Teaching Arithmetic: Lessons for Extending Multiplication, Grades 4–5
by Maryann Wickett and Marilyn Burns

View Lesson

Grades 4–5

More About Dates

Materials

A collection of coins dated before 1990, placed in a clear plastic bag

Overview of Lesson

Marilyn is always on the lookout for ways to provide students experience with computing mentally. Her colleague Jane Crawford gave her the idea of presenting older students with the problem of figuring out the ages of coins. To prepare for the lesson, Marilyn collected loose change for several days, choosing coins that were made before 1990. Marilyn planned to ask the students to figure in their heads rather than use paper and pencil. Her goal was for them to focus on making sense of the numbers and to discuss the different strategies they used for figuring. Marilyn tried this lesson with Annie Gordon's fourth and fifth graders in Mill Valley, California.

The lesson below includes Marilyn's account of what transpired when she taught the lesson.

Lesson Outline

Focus or Warm-Up

1. Show the class the plastic bag of coins. List on the board how many of each coin are in the bag. For example, my bag contained the following:

19 pennies
7 nickels
1 dime
3 quarters

2. Ask students to figure out in their heads how much money there is in the bag.

3. Ask several students to explain how they figured what the coins were worth (in my case, they totaled $1.39). Record their answers on the board as they report. In my class, as Dylan reported, I wrote:

25 × 3 = 75
75 + 10 = 85
7 × 5 = 35
85 + 35 = 120
120 + 19 = 139
139¢ = $1.39

Introduction

4. Now tell the students that you have another problem for them to solve mentally. Choose one of the pennies, and show them where, for example, 1978 appears on it. Ask the students, "What does the nineteen seventy-eight tell us?" Usually students know that it is the year the coin was made. Now ask students, "How old is this penny?" Tell students to talk with their partners about this.

5. Ask one student in each pair to raise his or her hand and explain how they figured out their answer.

When I taught this lesson to a class in the year 2000, students reported several different methods of figuring that the penny was twenty-two years old. Lucy said, "I know that nineteen seventy is thirty years away from two thousand because seventy is thirty away from one hundred. Then I subtracted eight from thirty to get twenty-two."

Benny reported, "I knew that nineteen eighty was twenty years away from two thousand. I still had two more years because nineteen seventy-eight is two years from nineteen eighty. So twenty plus two is twenty-two."

Gabe said, "Ten years gets to nineteen eighty-eight and ten more years gets to nineteen ninety-eight and two more gets to two thousand."

Write on the board as students report, to model for them how to record their thinking.

6. Pose the same question for another coin, again recording while students report. I posed the same question for a 1983 nickel.

Exploration

7. Then have students each take one of the coins and, working individually, calculate its age. "First figure in your head," I directed the students I was working with, "and then write an explanation of how you got your answer." (See Figure 1.)

Figure 1. In a classroom in 2000, after explaining how she knew that her 1987 penny was thirteen years old, Rachel showed her calculations.

Lesson Notes for the Teacher

This activity is good to repeat from time to time. By figuring out problems themselves and hearing others' strategies, students become more adept at mental calculations.

Featured in Math Solutions Online Newsletter, Issue 34

View Lesson

Grades 4–5

Line Segments on the Geoboard

Geometry comes to life in this lesson, as Rusty Bresser has fourth graders use geoboards to explore making pairs of line segments that touch exactly nine pegs, record them on dot paper, and label them as parallel, intersecting, or perpendicular. Rusty is the author of the new book Math and Literature, Grades 4–6, Second Edition (Math Solutions Publications, 2004).

Robin Gordon's fourth graders had been studying geometry, and I visited the class to provide them with an additional experience. The students watched attentively as I switched on the overhead projector and showed them my clear plastic geoboard and two geobands.

"Today we're going to use geoboards in our math lesson," I told the students. "Since you haven't worked with geoboards yet this year, I'm going to give you about five minutes to explore and see what sorts of shapes you can make."

I directed one student from each table to get one geoboard and two geobands for each student at the table. As soon as the students received their materials, they got to work.

After five minutes, I interrupted them and gave a new direction: "Now I'd like you to come to the rug area, but I want you to leave your geoboards and geobands at your table. We'll use them later in the lesson."

Once everyone was seated on the rug, I said, "I know that you've been studying geometry lately. One of the things you've learned about is lines. Think about what you know about lines and then turn to someone sitting next to you and take turns sharing what you know." After a couple of minutes, I called them back to attention and elicited ideas.

"They go on infinitely," said Brandon.

I drew a line on the whiteboard like this:

"When mathematicians draw lines, they add something," I began.

"Arrows!" several students exclaimed.

"That's correct," I said, adding arrows on each side of the line I'd drawn:

"The arrows mean that the line goes on forever, or infinitely, in both directions and in a straight path," I said. "What else do you know about lines?"

"When two lines intersect, they can be perpendicular," said Dana.

"Can you come up and show us?" I asked. Dana drew two lines on the whiteboard:

"These lines intersect because they have a point in common," I said and then drew a dot where the lines crossed. "What makes them perpendicular?" I asked.

"They make four right angles," Dana responded. I wrote the words perpendicular lines next to Dana's drawing.

"What else do you know about lines?" I asked.

"Lines can cross," Eduardo said. "Just like Dana's."

"What do mathematicians call it when lines cross one another?" I asked.

"Intersect!" several students exclaimed. I wrote the word intersect on the whiteboard and pointed to where Dana's lines intersected.

"Some lines have a beginning point and then they go on forever in one direction," said Hernan. I drew on the whiteboard what I thought Hernan meant. Hernan nodded to show his agreement.

"What do mathematicians call Hernan's idea?" I asked the class.

"A ray," several students replied.

I wrote the word ray next to the drawing and explained, "A ray has one endpoint and extends infinitely in one direction."

"Anything else you know about lines?" I probed.

Nina added the last idea. "There are lines that have two endpoints," she said. "I think it's kind of like part of a line. Line segments!"

"That's correct, Nina," I confirmed. "A segment is part of a line that has two endpoints and a definite length." I wrote line segment and drew an example for students to see:

To introduce the problem that the students would explore, I held up a geoboard for the class to see and stretched a geoband around the five pegs in the second row.

I said, "Talk with your neighbor about why I can show an example of a line segment on my geoboard, like this, but not of a line or a ray." After a moment, I called the students to attention and talked with them about how the pegs are always endpoints, so they don't allow us to show a line or a ray because each has an infinite length.

Next I said, "Now I'm going to use another geoband to show two line segments on my geoboard. See what you notice about the line segments I make."

After a moment, I called on Enrique. "They have two endpoints each," he observed.

"They cross . . . I mean they intersect," added Esmerelda.

I asked, "How many pegs do both line segments touch altogether?"

After some counting aloud, several students responded, "Nine!"

To verify, I touched each peg as we counted together. I explained, "When both geobands touch the same peg, you can count that peg only once."

Next I explained what they were to do. "In a minute, you'll make your own line segments on your geoboards back at your tables," I said. "Your challenge is to make different examples of two line segments, but each time they should touch exactly nine pegs. When you have an example, copy it onto geoboard dot paper." I held up a piece of dot paper for the class to see. To model recording, I quickly sketched a geoboard on the whiteboard and drew on it the two line segments I had made.

"After you draw a picture of your two line segments, write a description underneath," I directed. I wrote the words intersecting line segments underneath my sketch on the whiteboard.

I then changed the position of the geobands, making a new design to be sure that the students understood that two line segments with a common endpoint are also intersecting. I showed the class my new design and then sketched another geoboard, drew what I had made, and wrote intersecting line segments underneath.

I said, "Do you think I did this correctly? Do they touch nine pegs? Is my label OK? Talk with your neighbor." There was disagreement among the students about my label, and this gave me the opportunity to point out to them that as long as two line segments had a point in common, they were intersecting.

Finally, I made one more example on my geoboard—two line segments that were parallel:

I held up the geoboard for students to see. "What do you notice about these two line segments?" I asked.

"They're parallel," observed Esmerelda.

"And they touch nine pegs, just like your other examples," Charles added.

As with the other examples, I quickly sketched a geoboard on the whiteboard, drew on it the two line segments I'd made, and wrote parallel line segments underneath. "If these segments extended in both directions and became lines, they would never intersect," I said.

I then explained, "When you return to your desk, use your two geobands and your geoboard to find as many different ways as you can to make two line segments that touch exactly nine pegs. When you find a way, copy it on your geoboard dot paper and then label it as I showed."

As the students got to work, I heard them using correct math terminology (perpendicular, parallel, intersecting) to identify their pairs of line segments; they seemed excited and challenged by the task of finding as many different arrangements as possible. However, I noticed a few students recording line segments that touched fewer or more than nine pegs. I asked for the students' attention.

"Once you've made two line segments on your geoboard, show your work to someone next to you to check whether they touch exactly nine pegs. Then you can make a drawing of your line segments on your geoboard dot paper and label it," I told them.

As I watched the students continue working, I noticed that some had duplicate designs drawn on their papers. For example, Eduardo had drawn two parallel line segments on his geoboard dot paper. If he rotated that drawing, the line segments would be exactly the same as another design on his paper.

I decided not to call examples like these to students' attention. Rather, I kept the focus on making intersecting, parallel, and perpendicular line segments and correctly labeling the designs.

Figure 1. Henry's work

Figure 2. Rain's work

Related Publication:
Math and Literature, Grades 4–6, Second Edition
by Rusty Bresser

From Online Newsletter Issue Number 17, Spring 2005

View Lesson

Grades 4–5

More or Less Than One-Half

In this lesson, Marilyn Burns shows fifth-grade students a fraction and they decide if it's more or less than one-half and then explain their reasoning. This focus on one-half helps establish it as an important and useful benchmark. The lesson also provides practice with mental computation of whole numbers as students compare numerators and denominators. More or Less Than One-Half will appear in Marilyn's forthcoming book Teaching Arithmetic: Lessons for Introducing Fractions, Grades 4–5, to be published in fall 2001 by Math Solutions Publications.

I used this activity when I had some time left at the end of a periodor as a warmup at the beginning of class. I'd write a fraction on theboard and ask if it was more or less than one-half. Students who answered also had to explain their thinking. After each student's response, I'd ask, "Does anyone have another way to explain that?" In this way, students focused not only on answering and explaining their reasoning but also on trying to think of different ways to explain answers. I'd continue discussing the fraction until all students who wanted had had a chance to explain.

Here's how the activity went with a class of fifth graders in the fall of the year. I wrote on the board:

2/3

"Is this more or less than one-half?" I asked.

Davy began by saying, "There are three thirds in a whole, and two-thirds is more than halfway to the whole."

"How do you know it's more than halfway?" I probed. Davy wasn't sure.

"Listen to other ideas," I said, "and see if they can help you explain more." I called on Ramon next.

"On a measuring cup, the line for two-thirds is above the one-half line," Ramon said. "It's like halfway to a whole cup after half a cup."

Leslie asked to come to the board. She drew a circle and divided it into three equal-size wedges. She said, "If you had a cookie cut into thirds like this, you can see that one-third is less than one-half. If there were two people and you each took one-third, then you'd have to share some more to get one-half each. So one-half is one-third plus some more."

Rachel's explanation was more abstract. "If two-thirds was the same as one-half, then two would have to be half of three. But it's more, so two-thirds has to be more."

One day, I chose one and one-quarter for the fraction. When I wrote it on the board, some students laughed and others blurted out comments: "Simple!" "That's easy." "No-brainer." Practically every student raised a hand.

"More or less?" I asked. "Let's all say the answer softly together." That took care of the answer.

"Who can explain why one and a fourth is more than one-half?" I then asked. Again, there were lots of volunteers.

"It's obvious," Daniel said, "because one-half is less than one and one and one fourth is more than one, so it has to be more than one-half."

"It's more than twice as big," Sadie said, "because one-half and one-half are one and one and a fourth is even more than that."

"On the number line, they're on different sides of one," Emma said. "That shows one-half is less."

Even for an obvious solution, students may think in different ways. Listening to their thinking often gives me new insights into students' understanding. Also, sometimes students think in ways I hadn't thought of, giving me new ways to look at the mathematics.

For this activity, I sometimes selected fractions with larger numerators and denominators, at times choosing fractions that were easy to analyze, such as 61/100 or 400/1000, but at other times choosing fractions that also offered a mental computation challenge, such as 127/260, 89/180, even 267/498. The students seemed to like stretching their thinking to decide if fractions like these were more or less than one-half.

From Online Newsletter Issue Number 2, Summer 2001

Related Publication:
Teaching Arithmetic: Lessons for Introducing Fractions, Grades 4–5
by Marilyn Burns

View Lesson

Grades 4–5

Literature-Math Connection—Moira's Birthday

Collect and use data to solve a problem. Use collected data to develop statistical concepts of mean, median, and mode.

References:

Moira's Birthday, by Robert Munsch (Annick, 1992)

Materials

Book: Moira's Birthday
Small,square sticky notes (2-by-2-inch), at least 1 per student
Snap Cubes—approximately100–150 (maybe more if your class is larger than 25)
Large sheets of newsprint, 1 sheet for each group of 4 students
Chart markers for each group of 4

Reviewed:

Vocabulary data
mean
median
mode

Summary of the Book Moira's Birthday

This book, written by Robert Munsch, is a birthday story created for Moira Green, a girl who lives in Hay River in the Northwest Territories. She asked him to create a new birthday story for her birthday party. In the story, Moira wanted to invite all the students, grades K through 6, to her birthday party. Neither of her parents thought this was a good idea and limited her to six kids. She went to school and invited six, but friends who were not invited begged to be included. By the end of the day, all of the students in grades K through 6 had been included in the birthday invitation. Of course, Moira did not tell her parents because she thought they would be upset. On the day of the party, kids began to arrive, two hundred in all, quickly filling up the house. Moira's mother worried about the food. Moira told her not to worry because she knew what to do.

Moira called a pizza place and ordered two hundred pizzas. The owner told Moira that was too many and he could only send ten. Moira also called a bakery to order two hundred birthday cakes. The baker also responded that the order was too big and he could only send over ten cakes. The ten pizzas and ten cakes arrived and were quickly devoured by the two hundred kids who came to the party. Still hungry, the kids at the party left to get more food to bring back to Moira's house. After several hours, they all showed up with food that they promptly ate, leaving Moira with the problem of a messy house, which she solved using the presents kids had brought for her birthday. After all the kids left, the remainder of her orders were delivered and dumped on her front lawn—190 cakes and 190 pizzas.

The story provides the context for thinking about the problems that Moira had with her birthday.

It also provides a context for collecting and using information to estimate how much pizza to order for a birthday party of two hundred kids. The data collected provides a context to introduce the concept of average using mean, median, and mode.

Lesson Outline

Focus or Warm-Up

Introduce the book Moira's Birthday to the class. Provide background information about the author and the story.
Read the book. When finished, pose this question: "What was Moira's problem?"

Possible student responses:

She ordered too much food.

She ordered too much cake.

Too many people came to her party.

She ordered too much pizza.

Introduction

3. Focus on the problem of too much pizza. Use these and other questions to launch a class discussion about Moira's pizza order:

How many pizzas did Moira order?
How do you think she decided on that number?
Was it a reasonable number? Explain your thinking.
What ideas do you have for what she could have done to decide on a more reasonable number of pizzas?
How many slices of pizza would it take to feed our class? How could we find out
How many pizzas would that be? How many slices do you usually find in a pizza?
How can we use information like this from our class to make an estimate of how many pizzas would feed two hundred kids at a birthday party?

4. Following this discussion, let students know that you will help them collect and organize information about slices of

pizza that they eat and use that information to learn about some new math ideas and to solve Moira's problem.

5. Ask each student to decide how many slices of pizza he or she might eat at a party like Moira's (or maybe have them consider how many slices their mom might let them eat). Have each student write his or her number of slices on a sticky in writing large enough to be seen across the room. Guide students in posting their data from smallest to largest along one section of the board, like the following example.

001112222333333333333444556

6. Using the data lined up in order from smallest to largest, pose the following question:

• What one number do you think is the best to tell about how many slices of pizza each person in our class might eat? Be ready to explain your reasons for choosing that number.

After listening to students' ideas, let them know that mathematicians also have ways to determine a number to use that would best tell about how many slices of pizza each person in your class might eat, in fact, they have three ways that you'll talk about today. As you introduce those ways, link them to the ideas students have shared.

Median

Let students know that mathematicians sometimes use the median in a set of data to solve problems. Ask students how they could figure out which response is in the middle of the set of pizza slices data posted on the board. Label the middle piece of data with the word median.

Let students know that mathematicians would call this the median in your set of data and that it can be found by ordering the data from smallest to largest and determining the data point that's in the middle of the sequence. Mathematicians sometimes use the median in a set of data to make some estimates about a group. Pose this question:

• What estimates could be made about our group using the median in this set of data?

Mode

Mathematicians also use the mode to make estimates about a group. Pose the following question about the data:

• What number of slices would describe the amount of pizza most of you would eat? Call this the mode, the most common number of slices.

Ask students for their ideas on how the data could be reorganized to easily show the number of slices chosen most often. Reorganize the data and label the mode. For example:

What do you notice about the numbers we identified as the mode and the median?
Do you think the median and mode in a set of data will always be the same? We'll keep that question in mind as we continue working with data and finding the median and mode.
Was it a reasonable number? Explain your thinking.

Mean

Let students know they are going to work as a class to figure out how many slices of pizza each person would get if they evenly distributed the number of pieces represented by your data.

Ask each student to take Snap Cubes to represent his or her number of slices of pizza. So if someone chose zero pieces of pizza, he or she would have zero cubes; if someone chose three pieces of pizza, then he or she would have three cubes. Ask everyone to stand and pair up with someone who has a different number of cubes. In pairs, have students make a stack of all cubes from the partnership. Then they should take the cubes and split them into two equal stacks of cubes or as near equal as possible. Continue the process of pairing up with partners having a different number of cubes, combining the cubes, and then splitting them into equal or near equal stacks. Continue the pairing and splitting until everyone has the same or almost the same number of cubes. In this example, students would have either two or three cubes after the process of pairing and sharing.

Pose the question:

What can this process tell us about the approximate number of slices of pizza we'd need for each person in our class?

Let students know that they just went through a process of evenly distributing cubes in the group to represent evenly distributing slices of pizza. You may want to model the process mathematicians would use to find the mean number of slices: adding up all the numbers of slices students reported (in this case, 75) and dividing by the number of students in the group (in this case, 27), which would give 2.7777 slices or a number of slices between 2 and 3.

7. Follow up with these questions:

How many of you ended up with the number of slices of pizza that you wanted, represented by the cubes?
How many of you didn't?
Who ended up with more slices or more pizza than you wanted?
If we took slices of pizza that people didn't want and gave them to those who didn't have as much as they wanted, do you think we'd end up with a group of kids who got enough pizza?

8. After taking a look at the data and these three statistical benchmarks in the data (mean, median, and mode), ask the following questions:

We found that the median of our data was three, the mode was three, and the mean was between two and three. What does this tell you about the number of pieces per person we might use to make a prediction about the amount of pizza we might eat as a class?
What did you notice about the numbers that represented the mean, median, and mode in this situation about pizza? As we collect data for other situations, we'll look to see how the mean, median, and mode compare.

Exploration

9. Distribute the newsprint and markers. Pose the following problem for students to work on as a group of four:

How could we use what we've found out about our class and the number of slices we'd eat to make a better prediction about the following problem:

What is a reasonable number of pizzas Moira could have ordered to feed the 200 kids at her birthday party?

10. Let students know that in their problem solutions, they need to include the following:

Statement of the problem to solve
Explanation of the solution process using words, diagrams, and/or symbols
Statement of the answer to the problem

Also, let students know they should be prepared to share their strategies with the class in a whole-class discussion.

11. While observing students at work, decide on a general order you will use to ask students to share their solutions, providing a scaffolded discussion for students that supports access for all students to the variety of ways the problem has been solved. You may want to alert groups that you will ask to present early in the discussion.

Summary

12. Call students together for a class discussion of their solutions to the problem. The goal of the discussion is to reach a common understanding of the problem and its solution and to see how solutions compare and how approaches to solving the problem are the same and different. As each group presents, classmates should listen with the following questions in mind:

How does this group's solution compare with ours?
How is the approach the same as ours? Different?
What questions do I have about their work?

13. In the course of discussion, revisit the ideas of mean, median, and mode, and help students identify how they used those ideas in their problem solutions.

Featured in Math Solutions Online Newsletter, Issue 34

Find more classroom lessons online at mathsolutions.com.

Visit the "Books & Resources" section and click on "Free Classroom Lessons."

View Lesson

Grades 4–5

Clear the Board

This activity is excerpted from Minilessons for Math Practice, Grades 3–5, by Rusty Bresser and Caren Holtzman (Math Solutions Publications, 2006). Amy Jackson presented this lesson to her students in Florence Elementary School in San Diego, California. In this dice activity, the class works together to generate the numbers one through twelve in order. Clear the Board offers opportunities for mental computation and helps build students' number and operation sense. Students need to think flexibly and consider many possibilities in order to find the solutions to the computation challenges involved.

Amy Jackson wrote the numbers 1 through 12 vertically on the board and asked her students if they'd like to play a game. Their response was positive.

"Great," she replied. "This game is called Clear the Board. Our goal is to systematically get rid of each number I listed. We have to get rid of the numbers in order, beginning with one first and then moving to two, and all the way to twelve.

"I'm going to roll three dice. I'll write the numbers rolled on the board so you can see what came up. Then you need to think about how you can use these numbers to make the number one. You may use any operation—addition, subtraction, multiplication, or division—or a combination of operations. When you find a way to make the number one, I'll cross off the one on the board and we'll move on to the next number, two. Ready? Here I go."

Amy rolled a 1, a 3, and a 5 and wrote these numbers on the board.

Amy was surprised that students appeared to be struggling. Why didn't they just point out that one of the dice came up with a 1? she wondered. Then she realized her directions might not have been clear enough.

"I need to clarify something," she told the class. "Even though there are three numbers, you don't need to use all of them. You can use one, two, or all three of the numbers rolled to make the number you need."

Marcello raised his hand. "How about five minus three minus one?" he suggested.

While Amy was expecting a simpler solution, she wrote Marcello's idea as an equation on the board next to the 1. Adriana suggested the need for parentheses around 5 – 3. Technically parentheses are not required in this situation; however, their use is not incorrect and adds clarity. Amy decided to include them. She then pointed out that since she had rolled a 1, they could use just that 1, and she recorded that on the board too.

1 (5 – 3) – 1 = 1, 1 = 1

"OK, now that we've made one, we can cross it off. We're on our way to clearing the board. Next up is two."

"Three take away one is two," Enrique announced. "You could also do five minus three," Jamal pointed out.

Amy wrote equations for their ideas next to the 2 and then crossed off the 2. Now that the students understood the mechanics of the activity, she gave them time to talk in groups about ways to make the rest of the numbers. Soon the board filled up with a variety of solutions for the next few numbers. While Amy was impressed with the students' flexible thinking and comfort with mental computation, she was concerned that the game would go on and on if she didn't set a limit. The students were more interested in finding multiple solutions for each number than moving on to the next number. To keep the game moving, Amy decided to limit responses to one equation for each number. In a few minutes the board looked like this:

(5 – 3) – 1 = 1, 1 = 1
3 – 1 = 2, 5 – 3 = 2
3 = 3, (5 + 1) – 3 = 3, (5 – 3) + 1 = 3
3 + 1 = 4, 5 – 1 = 4
5 = 5, 5 x 1 = 5, 5/1=5
5 + 1 = 6
(5 + 3) – 1 = 7
5 + 3 = 8
5 + 3 + 1 = 9
(3 – 1) x 5 = 10
?
?

The class got stuck on eleven, so Amy told the students it was time to roll again. Before rolling, she asked a question to assess their comfort with the various operations.

"So," she said, "I'm going to roll the three dice again. What numbers would you like to get now?"

"A five, a six, and a one," Cristina offered.

"A five, a six, and anything would work," Kendrick pointed out. "How about a four, a four, and a three?" suggested Christine. "Or a two, a three, and a six," J. P. added.

This quick question showed Amy that her students were comfortable breaking eleven apart in different ways but were not as comfortable thinking about operations other than addition. Perhaps with more time and practice, they would open up their thinking.

She rolled the dice and got two 5s and a 1. She wrote these numbers on the board below where she'd written the first roll. Then she turned to the class.

"Tough one, huh?" she teased.

"Just put five plus one plus five," Kenyatta directed. Amy wrote the equation next to the 11 on the board.

11) 5 + 1 + 5 = 11

"Aren't you supposed to put parentheses, Ms. Jackson?" Jessie challenged. "No," J. P. disagreed. "We don't need them."

"Let's check," Amy suggested. She pointed to the first 5 and the 1. "What's five plus one?" she asked the class.

"Six."

"Plus five more equals?" "Eleven."

"OK," Amy continued, "let's see what happens if we start with the other two numbers." She pointed to the 1 and the second 5. "What's one plus five?" she asked.

"Six."

She pointed to the first 5. "Plus five more?" "Eleven."

"Hmm," she concluded, "we get the same answer regardless of where we start. So we don't really need parentheses for this addition equation." She wrote on the board:

(5 + 1) + 5 = 5 + (1 + 5)

Although this wasn't the time to go into a lengthy discussion about the purpose of parentheses or the associative property, it was a nice opportunity to model the concept and notation briefly.

Amy gave the students a minute to talk to a partner about how to use these rolls to make twelve. After some computational gymnastics, the students agreed that they couldn't make twelve with those three numbers, so Amy rolled again. This time she got a 5, a 2, and a 1. She wrote these numbers on the board below the previous roll. The students took a minute to consider the possibilities. Christine raised her hand.

"I've got one," she announced. "Five plus one times two."

"Do I need to use parentheses for that equation?" Amy asked, reinforcing their prior discussion.

"Yes," Christine told her. "Put them around the five plus one."

Amy wrote the equation on the board and had the class double-check to make sure everyone agreed.

(5 + 1) x 2 = 12

Amy crossed out the 12 on the board and congratulated the students for clearing the board. She asked how many rolls it took for them to accomplish their task. Since she had written each roll on the board, the students had an easy reference. Amy wondered aloud if they thought it would take more or less than three rolls when they played next time. Leaving them with something to think about set the stage for future experiences with Clear the Board.

View Lesson

Grades 4–5

Number Theory: Differentiating Within a Unit A Lesson with Fifth Graders

This fifth-grade teacher knows that making connections among concepts and representations is a big idea in mathematics. She wants all of her students to be able to represent and connect number theory ideas. For example, she wants her students to represent square numbers visually by making squares on graph paper as well as to connect those representations to the symbolic notation y = x2 . She believes if students "owned" this connection, they wouldn't have so much trouble remembering the formula for finding the area of a square.

The teacher will incorporate this idea into the unit, but first, she wants to informally pre-assess her students to find out how they might classify numbers. She wants to know whether they can readily identify "classes" of numbers, such as square numbers, even numbers, prime numbers, and multiples. In lieu of just giving them lists of numbers and having them extend or identify the types of numbers in the lists, she'll launch the unit with a problem-solving activity. The activity will allow her to get a feel for her students' common understanding and to identify students who may need more or less support in this area. She'll observe her students carefully as they work and make notes about their thinking and writing. She'll be able to use the data to inform the lessons that follow.

She asks students to get out their math journals and pencils and gather in the meeting area. She writes the numbers 4, 16, 36, 48, 64, and 81 on the whiteboard and asks the students to copy these numbers in their journals. She then writes:

Which number does not belong?

4, 16, 36, 48, 64, or 81

She then says, "Use your journal to jot down your ideas. I want everyone to have some time to think. Please don't share until we have a green light." As the teacher says this she points to the depiction of a traffic light next to the whiteboard and places the Velcro®-backed star on the red signal. Students are given time to work independently while the room is quiet.

"It's the four!" Sheila calls out immediately after the teacher moves the star to the green signal. The teacher thinks about asking how many other students agree with Sheila, but as she wants them to eventually find more than one candidate, she decides not to have them commit to this thinking. Instead she asks, "Does anyone think they know why Sheila might think that the four does not belong?" Several hands are raised and others are nodding their heads.

She calls on Tara, who explains, "It's only one number. The rest are two."

Dewayne adds, "It's the only number less than ten; the rest are between ten and one hundred."

"It's only one digit; the rest are two-digit numbers," says Marybeth.

"Wait," says Melissa, "I got another number answer, too. It's eighty-one."

"I want you to hold on to that thought," says the teacher. "Right now, please write in your journals any other reasons why a number doesn't belong in this group. Like Tara, Marybeth, and Dewayne, you might find different reasons for why a particular number does not belong. Like Melissa, if you change the rule, you might find a different number that does not belong. You will have about ten minutes. You can work on your ideas the whole time or, if you finish early, find a partner who has finished and share. Some of you might find rules to eliminate each of the numbers, one at a time."

Already, the teacher has heard minor variations in the students' thinking. Tara seems to recognize a visual difference in the numbers, while Marybeth uses more formal language to describe this attribute. Dewayne refers to the value of the numbers and Melissa is willing to verbalize another possibility. The teacher looks around and sees everyone has spread out a bit and begun to think and write. After a couple of minutes, she notices that a few of the students have stopped after writing about the number four. She quietly asks them if they remember Melissa's number. Some do and others need to be reminded, but with a bit of coaching, all are able to identify eighty-one as different because it is an odd number.

After almost ten minutes the teacher notices that all but two of the students are talking in pairs about their work. She gives them a one-minute warning and when that time has passed,they huddle more closely and refocus as a group. The teacher asks them to draw a line under what they have written so far, and then to take notes about new ideas that arise in their group conversation. As the students share their work, several ideas related to number theory terms and concepts are heard. Jason identifies the square numbers in order to distinguish forty-eight from the other numbers. Judy also refers to square numbers when she eliminates sixteen as "the only number that is the square of another number in the list." Naomi tells the class that eighty-one is different because it is not divisible by four. Most interesting to the teacher, Naomi also says, "All of the other numbers are even, but they have to be if they are multiples of four." After each comment there is a short conversation. Sometimes the teacher asks another student to restate what has been heard, or to define a term, or to come up with a new number that could be included in the list to fit the rule.

Each time, the teacher makes sure there is time for students to take some notes and that the majority of them agree that the classification works. She secures their agreement as she points to each number in the list and says, for example, "Is this a multiple of four?"

Two students use arithmetic to find a number that is different. Benita's work is the most complex. She identifies eighty-one as different because "you can't get there with just these numbers." She explains her work further by writing the following equations on the board:

64 ÷ 16 = 4

64 ÷ 4 = 16

48 – 16 + 4 = 36

64 – 16 = 48

16 × 4 = 64

Two days later, Naomi surprises the class with a new equation: (16 ÷ 4) + (16 ÷ 4) + (36 ÷ 4) + 64 = 81. Benita is crestfallen at first, but then brightens as she adds "without using one of the numbers more than once" to her rule.

While students do not experience this activity as a pre-assessment task, it does give the teacher some important information that she can use to further plan the mini-unit on number theory. She can determine students' willingness to think divergently, to come up with different possibilities for the same number. She has an indication of their understanding and comfort level with concepts and terms such as factors, multiples, divisible, primes, and square numbers. With students' written work, she can determine the ideas and terms they included prior to the group debriefing. She can also gather evidence as to whether the students' notes are accurate and complete, contain their own additional ideas, or include drawings, lists, and definitions.

From this information she can decide how to adapt her content for different students. The complexity of ideas can vary. Some students can reinforce ideas introduced through this activity, while others can investigate additional ideas such as triangle numbers, cubic numbers, and powers. She can have some students explore ideas that will allow them to complete or create if-then statements such as "If a number is a multiple of six, then it is a multiple of _______."Divisibility rules and common multiples can be used to solve problems and relationships can be generalized through algebraic equations.

Once content variations are determined, process is considered. Some students can draw dots to represent square and triangular numbers so that they have a visual image of them while others can connect to visual images of multiples and square numbers on a hundreds chart. (See Figure 1.)

Figure 1. Visual images of four on a hundreds chart and square numbers on a multiplication chart.

The teacher can create some packets of logic problems, such as the one that follows, that require students to identify one number based on a series of clues involving number theory terminology:

What is my locker number?

The number is between 250 and 275.

It is an even number.

It is a multiple of 3.

It is not a multiple of 5.

It is divisible by 4.

My locker number is _ _ _.

Another packet could offer brainteasers that involve clues about remainders:

What's the fewest number of pennies I could have? When I put them in two equal stacks, there is one penny left over. When I put them in three equal stacks, there is one penny left over. When I put them in four equal stacks, there is one penny left over.

(You might want to get some chips to help you model the pennies.)

Some students could write rap lyrics to help them remember the meaning of specific terms. Other students could play a two- or three-ring attribute game with number theory categories as labels; they would then place numbers (written on small cards) in those rings until they could identify the labels. A learning center on codes could help students explore how number theory is related to cryptology. The teacher could think about pairs of students who will work well together during this unit and identify subsets of students that she wants to bring together for some focused instruction.

Then the teacher must think about product—how her students can demonstrate their ability to use and apply their knowledge of number theory at the end of the unit. For example, students might write a number theory dictionary that includes representations, pretend they are interviewing for a secret agent job and explain why they should be hired based on their knowledge of number theory, create a dice game that involves prime numbers, make a collage with visual representations of number theory ideas, or create their own problem booklet.

It's not necessary, or even possible, to always differentiate these three aspects of curriculum, but thinking about differentiating content, process, and products prompts teachers to

identify the mathematical skills and abilities that students should gain and connect them to big ideas;
pre-assess readiness levels to determine specific mathematical strengths and weaknesses;
develop mathematical ideas through a variety of learning modalities and preferences;
provide choices for students to make during mathematical instruction;
make connections among mathematics, other subject areas, and students' interests; and
provide a variety of ways in which students can demonstrate their understanding of mathematical concepts and acquisition of mathematical skills.

featured in Math Solutions Online Newsletter, Winter 2007–2008, Issue 28

Related Publication:
Math for All: Differentiating Instruction, Grades 3–5
by Linda Dacey and Jayne Bamford Lynch

View Lesson

Grades 4–5

Martha Blah Blah

In this lesson, excerpted from A Month-to-Month Guide: Fourth-Grade Math (Math Solutions,2009), Lainie Schuster has her fourth graders start the school year with an investigation that offers them the opportunity to work in pairs to collect, represent, and analyze data. She uses the children's book Martha Blah Blah, by Susan Meddaugh (Houghton Mifflin Company, 1996), as a springboard for the lesson. In this book, Martha, a dog who is able to talk as a result of eating a bowl of alphabet soup each day, finds herself in a pickle when the owner of the soup company reduces the number of letters in each can of alphabet soup. Fourth graders are tickled by Martha's amusing but unintelligible speech as a result of the missing letters and are delighted when Martha takes it upon herself to solve the problem of the missing letters.

After listening to the story, students pair up to investigate the frequency with which each letter in the English alphabet is used. They gather and organize their information, then summarize in writing what they notice about the letter frequencies. They create posters to show their findings and participate in whole-class discussions to share their thinking. To extend the activity, Lainie gives students pasta letters from which they form as many words as possible.

I began the lesson with a read-aloud of Martha Blah Blah. Following the reading, we had a class discussion about letter distribution in words. I asked the class, "What letters do you suppose occur most frequently in the English language? Why might that be? Do you think consonants are used more than vowels or vowels more than consonants?"

When everyone had had a chance to offer an opinion, I posed these questions: "How could we investigate the frequency of the letters we use? How could we collect the data? How could we chart the collected data? If Granny Flo, the owner of the soup company, was determined to eliminate the least-used letters from her soup, how could we help her make an informed decision (at least for talking dogs)?"

I directed the children to discuss these questions with their neighbor and come up with ideas for carrying out a letter-frequency investigation. As the children talked, I circulated around the room, listening and handing out a record sheet containing writing prompts. (See end of lesson for blackline master.)

When I felt everyone was ready, I called the class back together so students could share their ideas and discuss the writing assignment. Because I wasn't sure how much experience my students had had in data collection or working cooperatively, I felt it was important to have this discussion.

Several students said, "Count the letters on a page in our math book."

Others said, "Pick several sentences or a paragraph and see what letters are used and how often."

Still others offered, "One person can read the words while the other tallies the letters and we can take turns after each sentence."

I then inquired, "How might you chart the letter frequencies?" Almost everyone suggested that all the letters of the alphabet be written in a two-column chart with the letters in the left column and the distribution tallied in the right one. In response, I asked the children if it was necessary to write down all the letters in the alphabet or if they could write down just the ones that were in their reading selection. This led to a rich discussion about the importance of being able to quickly assess the holes in the collected data.

Then I described the task. I explained that, working in pairs, they were going to determine how often each letter of the alphabet was used. "To do this," I further explained, "you and your partner are to choose any book you like, select a short paragraph in that book, and analyze the frequencies of the letters used in that paragraph."

I gave each pair a piece of newsprint on which to record all their work. I told them to post the data they collected in whatever format they were comfortable with and to leave room on their newsprint for writing summary statements and posting the results of another task, which I would give them the next day. I also told them that they would have an opportunity to decorate their posters at the end of the investigation.

Once we agreed on what they were being asked to do, the children set off to collect and represent their data. Many chose a paragraph from a book they were presently reading. Others picked one of the books in the classroom book display. I had asked that the paragraphs be relatively short because it was the beginning of the school year, and I was more concerned about the manageability of the task than the length of the paragraph.

Before all the students were finished collecting their data, I asked for their attention, and we discussed the written part of the task. I asked the children to write two summary statements on their sheet of newsprint. I explained that a summary statement should explain what they noticed about the data they had collected. I suggested they ask themselves questions such as,"Is there a pattern of any kind in our data?" "Where are the 'lumps, bumps, and holes' in our information?" and "Which letters could Granny Flo eliminate and why?"

I had pairs work together to create summary statements but asked them to individually complete the writing prompts before discussing and comparing their opinions and answers.

As the children went back to work, I circulated around the room to offer assistance. Talking about the mathematics is one thing—writing about it can be quite another. I pushed students to use "because" statements as they wrote: "I would remove these letters because . . ."

Sometimes it was necessary to remind students to refer to their collected data as they wrote. (See Figure 1.)

Writing Prompts for Martha Blah-Blah We would suggest that Granny Flo take out the following 7 letters: Without these 7 letters, it would be difficult for Martha to say the following words: Without these 7 letters, it would be easy for Martha to say : Why?

Norah's completed Martha Blah Blah assignment.| A student's chart, made up of collected tally's from her collected data, she has has explained her collected data and what she found. | Math Solutions, Classroom Lessons

Figure 1. Norah's completed Martha Blah Blah assignment.

Since math time was almost over, I continued the lesson the next day. After students retrieved their newsprint, we began a class discussion of their findings and decisions. Most agreed that more consonants should be removed than vowels. As the children shared their findings, I asked questions such as, "Why do you think so?" and "On what are you basing your statement?" This helped them focus on the data in their charts and better articulate he "mathematical proof" on which their opinions were based.

I concluded this investigation with a word search made from a cupful of alphabet pasta. I gave each pair of students a paper cup containing twenty-five pieces of uncooked alphabet pasta. I then gave the following directions:

Pour the pasta in your cup on your desktop.
Construct as many words as you can from your letters.
Glue the pasta letters onto a piece of 9-by-12-inch construction paper with white glue.
Write the words you made underneath the pasta letters.
Mount this on the newsprint containing your data chart and summary statements.
Post your newsprint on the bulletin board.

Interest remained high for weeks, as students looked again and again at their classmates' work. They were especially curious about the frequency findings, the conclusions that were drawn, and the words that were made from the pasta letters.

Note: For additional activities focusing on Martha's dilemma, in which students analyze letter frequencies and apply their findings to the game of Boggle, see Math and Literature, Grades 4–6, by Rusty Bresser (Math Solutions Publications, 2004).

Worksheet for Martha Blah Blah activity and why seven letters should be removed | Math Solutions, Classroom Lessons

Writing Prompt for Martha Blah-Blah

Featured in Math Solutions Online Newsletter, Fall 2008, Issue 31.

View Lesson

Grades 4–5

Day-by-Day Math: Activities for Grades 3–6

Day-by-Day Math: Activities for Grades 3–6, by Susan Ohanian, is an eclectic and quirky collection of events — and the mathematical investigations, problems, or activities that are suggested by them.Each day of the year, from January 1 through December 31, lists historical events, each a lighthearted or serious moment. Some of the classroom suggestions are ideal for five-,ten-, or fifteen-minute main period "openers;" some can be used for longer class investigations; many are suitable for individual assignments. There's something in this diverse collection for everyone, which is sure to add an extra bit of oomph to your math instruction. Here are some dates and investigations that your students can explore this coming year:

January 23, 1951

The C102 jetliner makes history, flying from Toronto to Chicago to New York and back. It flies at twice the speed — 520 miles — and twice the altitude — 36,000 feet — of propeller-driven airplanes. At this altitude, planes are able to fly above unsettled weather.

Investigate

Find out how long it takes to make a typical commercial flight from New York to San Francisco. What is the hourly speed? Is the flight time from San Francisco to New York the same?

1975

During what is dubbed "The Storm of the Century," the wind chill is between –50° and –80° Fahrenheit in Duluth, Minnesota. Weather information is available from many online sources.

Investigate

Keep a weather graph charting the temperature for a month. Then find the average temperature for the month. Check an almanac to find out whether this is above or below average.

January 23, 1849

Elizabeth Blackwell, who had been turned down by 28 colleges before she found one that would let her study medicine, graduates from Geneva Medical College (now Hobart and Williams Smith Colleges) in Geneva, New York, at the head of her class and becomes the first woman doctor in the United States. For more information about Elizabeth Blackwell, check http://www.greatwomen.org/blkwele.htm.

Investigate

Look at the list of doctors in the yellow pages of the phone book. How many are male and how many are female? Can you determine whether female doctors are more apt to specialize in one field of medicine over another?

1985

The Coca-Cola Company announces it is replacing its 99-year-old recipe with a new formula. Customers react so negatively that on July 10 the same year it reintroduces the old Coke under a new name, Coca-Cola Classic.

Investigate

Every minute, people around the world drink 311,111 Cokes. How many Cokes are consumed in one week?

January 29, 1861

Kansas becomes the 34th state. The name Kansas comes from an Indian word meaning flat or spreading water. The state flower is the sunflower. The sunflower provides pioneer settlers in the Midwest with oil for their lamps and food for themselves and their stock. Native Americans roast sunflower seeds and ground them into flour for bread or pound them to release an oil for cooking and for making body paint.

Sketch of the state of Kansas, including a sunflower and a farmhouse | Math Solutions, Classroom Lessons

Investigate

Look at a live sunflower or a detailed picture of one. A sunflower has two distinct parallel rows of seeds spiraling clockwise and counterclockwise. The seeds are Fibonacci numbers, typically 34 going one way and 55 going the other way, although sometimes they are 55 and 89. Find other natural examples of Fibonacci patterns. Good places to look include pinecones, pineapples, artichokes, and African daisies. For a terrific site on Fibonacci numbers, go to http://www.ee.surrey.ac.uk/Personal/R.Knott/Fibonacci/fibnat.html.

1998

Carl Gorman, a gentle Navajo artist and one of the 400 Navajo code talkers during World War II, dies. Gorman and 28 other Navajo volunteers turned their native language into a secret code that allowed Marine commanders to issue reports and orders and to coordinate complex operations. Although the highly respected Japanese code crackers broke U.S. Army, Navy, and Air Corps codes, they were never able to break the Marine Navajo code. As Gorman's New York Times obituary notes, "Navajo is a language without an alphabet and with such a complex, irregular syntax that in 1942 it was estimated that outside of the 50,000 Navajos, no more than 30 other people in the world had any knowledge of it, none of them Japanese." Online information from the Native American museum that is part of the Smithsonian Institution is available at http://www.si.edu/nmai/nav.htm. The Navajo Code Talkers' Dictionary is available online at: http://www.history.navy.mil/faqs/faq61-4.htm.

Investigate

Team up with at least one other person and invent a code using numbers.

Related Publication:
Day-by-Day Math: Activies for Grades 3-6
by Susan Ohanian

View Lesson

Grades 4–5

One Pizza, One Penny

I was a little less than halfway through reading K. T. Hao'sOne Pizza, One Penny to Robin Gordon's fourth graders when I read what Ben Bear was thinking: "If Chris Croc can sell his cake, my pizzas can bring me a fortune! If each slice sells for one gold coin, and if I can sell 10 pizzas a day . . . that's 80 slices . . . for 365 days a year . . . ." I stopped reading and posed a question.

"Ben Bear says that he could sell 10 pizzas a day and that's 80 slices," I said, writing the numbers 10 and 80 on the board.

"How many slices are in one of Ben Bear's pizzas?" I asked. I gave students some quiet think time before asking them to turn to their partners and share their thoughts. After partners had discussed their ideas, I called them back to attention.

"I think there are eight slices in each of his pizzas," Marcos said.

"How did you figure that out?" I asked.

"I knew that ten times eight is eighty," he explained. "Ten pizzas times eight slices is eighty slices."

"Thumbs up if you agree, thumbs down if you disagree, and thumbs sideways if you're not sure," I directed. Almost everyone's thumb was turned up in agreement. I wrote 10 x 8 = 80 on the board.

"Any different ideas?" I asked.

Karen said, "I used division. It's eighty slices divided by ten pizzas and that makes eight slices in each pizza." I wrote 80 ÷ 10 = 8 on the board and acknowledged that there were indeed different ways to solve the problem.

Then I finished reading the book. The students enjoyed the colorful illustrations by Giuliano Ferri and were happy when Chris Croc and Ben Bear solved their problem of being hungry by buying food from one another, passing the one gold coin back and forth until there were no pizzas or cakes left.

I returned to the page where I had previously stopped to pose a problem and wrote on the board:

80 slices a day
1 coin for each slice
365 days

"What would you have to do to figure out how many coins Ben Bear could earn in a whole year?" I asked. I waited a few seconds and then called on Daniel.

"Multiply by each day," he said. "You'd have to do three hundred sixty-five times eighty."

"Why multiply?" I probed.

"'Cause it's like if you did eighty times two, that's eighty coins a day times two days," he explained. "But you have three hundred sixty-five days, so you multiply eighty coins times three hundred sixty-five days."

"Make sense?" I asked the class. The students nodded in agreement.

"Other ideas?" No one raised a hand, so I continued.

"I'm going to show you some numbers," I said. "One of the numbers is close to, but not the exact answer to, three hundred sixty-five times eighty." I then wrote on the board:

About 500 coins
About 2,500 coins
About 30,000 coins
About 350,000 coins
About 1,000,000 coins

Next, I asked the students to estimate which number they thought would be closest to the exact answer. With a show of hands, we discovered that 10 students thought 2,500 coins was closest, 10 students thought 30,000 was closest, 4 estimated 1,000,000, and no one thought 500 coins could be possible. I then gave students some time to talk with their partners about the reasonableness of the estimates. After a minute, I asked for their ideas.

Belinda said, "I think five hundred couldn't be closest to the answer because three hundred sixty-five times two is more than five hundred."

"What's three hundred sixty-five times two?" I asked the class. I gave the students a few seconds to think, then called on Jesus.

"It's seven hundred thirty," he reported. "First I did five plus five is ten. Then I did sixty plus sixty is one hundred and twenty. Then I doubled three hundred and that's six hundred. I added one hundred twenty plus ten plus six hundred, and that's seven hundred thirty."

"So five hundred isn't reasonable," I said. "What about some of the other numbers?"

"A million is way too big!" exclaimed Marco. Everyone seemed to agree.

"What about twenty-five hundred?" I asked. "Reasonable or not?"

"No, it's not a good number," said Rain. "If you multiply three hundred times ten you'd get three thousand. One hundred times ten is one thousand, so three hundred times ten has to be three thousand, and that's more then twenty-five hundred!" Rain's reasoning made sense to the class.

"So the only choices we have left are thirty thousand and three hundred fifty thousand," I said. Next, I asked for a show of hands to find out what students' estimates were now that they'd had a chance to hear different ideas. This time, everyone thought that the exact answer was closest to 30,000, except for Henry, who stuck with 2,500.

"To find out the exact answer to three hundred sixty-five times eighty, what can we do?" I asked.

"Use a calculator!" suggested Amber.

"Multiply," said Anton. "I already know the answer!"

"OK, we'll have Amber get a calculator and see what she finds out," I said. "Anton, you can explain how you got the answer in the meantime."

After Anton explained how he figured the answer using the standard algorithm, we checked his result with Amber. We didn't spend time exploring other ways to find the exact answer because the focus of the lesson was on using estimates to think about reasonable answers, not exact ones. When Anton and Amber reported the answer—29,200—I ended the lesson by asking the students which number was closer to the exact answer: 30,000 or 350,000.

"It's closest to thirty thousand," said Jalen. "If you round twenty-nine thousand two hundred up to the nearest friendly number, it's thirty thousand. Three hundred fifty thousand is way too big!"

This lesson gave students opportunities to estimate and think about the reasonableness of answers, both of which help build students' number sense.

From Online Newsletter Issue Number 14, Summer 2004

Related Publication:
Math and Literature, Grades 4–6, Second Edition
by Rusty Bresser

View Lesson

Grades 4–5

Nicholas's Game

I wrote ¾ on the board and asked the class, "Who can think of a fraction that has a numerator that's greater than the numerator of the fraction I wrote on the board and also a denominator that's greater than the denominator on the board?"

A few hands sprung up. I suspected that some hadn't heard or didn't understand my directions, so I repeated them to get more students involved. I pointed at the fraction I had written on the board and said, "You're trying to think of a fraction with a numerator larger than three and a denominator larger than four. Raise your hand when you have a fraction in mind." About half a dozen more hands were raised. I called on Josh.

"Four-fifths," he said. I wrote 4/5 on the board.

"Yes, this follows my rule because the four is greater than three and five is greater than four," I said. I called on other students and recorded the fractions as they offered them.

"Fifteen-sixteenths," Lily said.

"Sixteen-sixteenths," Pamela said.

"Oh, I get it now," Claire said and raised her hand. "Eight-eighths," she said.

"Six-sixths," Jeremy said, following the pattern Pamela and Claire had set.

"Five-fifths," Michael offered, grinning.

"Four-fourths," Martin then offered.

When I wrote 4/4 on the board, I didn't have to correct him because Josh complained, "That doesn't work. You can't have a four on the bottom."

Martin's face reddened. "Can I change it?" he asked me. I reminded him that everyone could change his mind at any time in math class, as long as he had a reason.

Martin said, "Well, I need a bigger number on the bottom. I'll say four-eighths." I recorded this on the board.

I then asked, "How many fractions do you think there are that follow the rule?"

"Lots," Rebecca said.

"There's an infinite amount," Andrew added.

"How do you know that there are an infinite number of possible fractions?" I asked Andrew.

He explained, "Because you can always think of bigger numbers, and you can pair different numbers together."

I then drew three columns on the board and labeled them <, =, >. I reviewed the signs and then pointed to the last column labeled with the "greater than" sign. I asked, "Who knows a fraction that follows the rule and also belongs in this column?" I waited until about half the students had raised their hands.

"My fraction, sixteen-sixteenths, works," Pamela said.

"Explain why," I said.

"It's the same as one, and three-fourths is less than one," she said with confidence. Several other students raised their hands.

"Mine works, too," Jeremy said. "Six-sixths is one."

"Who can think of a fraction for the 'greater than' column that's not equivalent to one?" I asked.

Sam raised a hand. "Seven-eighths works," he said. "I know that because three-fourths is the same as six-eighths, so seven-eighths has to be more."

I continued by asking the class for fractions for the other two columns, each time having the student explain her reasoning for the fraction she identified. Then I repeated the activity using three-eighths and then one-fourth as starting fractions. I continued the lesson until only ten minutes remained in the period. Then I stopped to give the homework assignment.

To avoid confusion when they were at home, I duplicated the directions for the homework and distributed them to the class:

Nicholas's Game

1.Rule three columns and label them <, =, >. Above the columns draw boxes for the numerator and denominator of the starting fraction.

2.To find the starting fraction, roll a die twice. Use the smaller number for the numerator and the larger number for the denominator. (If both numbers you roll are the same, roll again so that the numerator and denominator of your starting fraction are different.)

3.Write at least five fractions in each column. The numerator and denominator in each fraction you write must be greater than the numerator and denominator in the starting fraction.

4.Choose one fraction from each column and explain how you know it belongs there. For example, write: I know that is greater than because____________.

5. You may use only one fraction that is equivalent to 1 whole, such as 5/5 or 11/11 or any fraction with the same numerator and denominator.

6. If you think of a fraction that follows the rule, but you're not sure in which column it belongs, write it to the side and bring it to class for a discussion of "hard" fractions.

I explained to the students what they were to do. I emphasized the fifth rule. "This is so you'll have the chance to stretch your thinking beyond fractions that are equivalent to one," I said.

The next day, I had students report about what they had learned from the assignment. (See Figures 1–3.)

Figure 1: Delia's explanations are clear and correct.

Figure 2: As Libby's paper shows, the game can provide experience with fractions greater than 1.

Figure 3: From his paper, Josh's understanding of equivalence seems strong.

"Getting fractions that are bigger is easy," Sam said. "You just have to use fractions that have a bigger numerator. Then it's more than one, so you're sure it works."

"I rolled four-fifths," Lily said. "But I thought it was too hard, so I rolled again."

"Did anyone think of a fraction that was too hard to place in one of the columns?" I asked. No one reported having this problem.

"Let's try the activity for one of your fractions," I then said. "Raise your hand if you think that the fraction you rolled would be particularly interesting for the class." Josh suggested three-fifths. I ruled columns and started the activity. We continued for about fifteen minutes. I then added Nicholas's Game to our choice list and switched to another activity.

From Online Newsletter Issue Number 5, Spring 2002

Related Publication:
Teaching Arithmetic: Lessons for Introducing Fractions, Grades 4–5
by Marilyn Burns

View Lesson

Grades 4–5

Figuring Areas

While browsing through About Teaching Mathematics recently, I came upon an activity, Area and Perimeter, that had once been one of my favorites but that I hadn't taught in quite some time. I decided to use it with ﬁfth graders in a different way than suggested in the book and have described below what I did. The students had already completed three other activities from About Teaching Mathematics— Foot Area and Perimeter, Squaring Up, and The Perimeter Stays the Same.

I chose the activity because I felt that the students needed more experience with ﬁnding the areas of shapes. To prepare for the lesson, I duplicated for each pair of students the shapes for the activity and a sheet of inch-squared paper. I used yellow paper for the shapes so that there would be contrast when they pasted them on white paper. Also, I made an overhead transparency of each of the sheets I distributed.

To begin, I projected an overhead transparency of the inch-squared paper, which was a 9-by-7 grid. We ﬁrst talked about the area of the grid. From their study of multiplication, the students knew to multiply 7 by 9 to get the area of 63 square inches. I showed them three options for recording this area: 63 sq in, 63 sq", and 63 in². (When the students later worked on ﬁnding the areas of shapes, I nagged them regularly to identify the units and record them in one of these ways.)

I next placed a 5-by-8-inch index card on the grid, positioning it carefully so its sides were on lines and it covered 40 of the squares completely. I asked, "What is the area of the index card?" Some students multiplied 5 by 8 to get the area of 40 sq in. Others counted to ﬁnd that 23 squares on the grid weren't covered and then subtracted 23 from 63.

I removed the 5-by-8-inch index card, folded it in half the short way, and cut on the fold. I placed one half on the overhead grid. All of the students knew its area was 20 square units, some ﬁguring that 20 was half of 40 and others multiplying 4 by 5.

I then trimmed the 4-by-5-inch card so it was a 4-by-4-inch square. It was easy for the students to ﬁgure out that its area was 16 square inches. Next I cut the square in half on the diagonal, making two triangles. I placed one of them on the grid. Some students knew immediately that its area had to be 8 square inches. "It's half of sixteen, so it has to be eight," Michael said.

Others weren't sure. "Some of the squares are only halves," Alicia said.

Tracing the triangle on the squared paper and then removing it helped the students see that it was, indeed, 8 square inches.

An illustration of a right triangle, projected over squares, some whole some cut in half. The illustration challenges the student to find the whole squares and see how the halves can be added to make a whole. | Math Solutions, Classroom Lessons

Janie explained, "There are six whole squares. Then two halves make a whole and two more halves make a whole. So it's eight squares altogether."

I then projected a transparency of the shapes the students were going to explore. I explained what they were to do, also writing the directions on the board:

Figure out the area of all the shapes and label them.
Cut out the shapes, order them from least area to greatest area, and glue them onto the 12-by-18-inch white paper.
Write about what you notice, what you learned, any questions you have, and any confusion you encountered.

Students worked in pairs and I circulated, giving help as needed. I suggested to some students that they place a shape on the inch-squared paper and count the squares it covered. (I assured Sam that this wasn't "cheating.") I talked with some about how the triangles were half of rectangles and pointed out that it was easier to ﬁgure the area of rectangles. I suggested to others who were having difﬁculty to orient shapes on the grid in different ways to make the problem easier. The parallelograms were the most difﬁcult for the students, and I gave suggestions about drawing a diagonal to divide them into two triangles. We had a double period for math (an hour and a half), so there was time for all of the students to ﬁnish, although some didn't get a chance to write as much as others. If the class had been a regular-length period, I would have collected their work and returned it to them to complete the next day.

About twenty minutes before the end of class, I gave a ﬁve-minute warning. Then I called the students together to discuss what they had learned. Kiko reported that she and Carlos used the small pieces to ﬁgure out the areas of the larger ones. "We especially used the one-inch square and the triangle that was one-half of a square inch," she explained.

Nicholas explained what he learned. "I didn't know that you could cut a parallelogram in half and get two triangles the same size. That was cool."

Tamika explained the difﬁculty that she and Jason had encountered. She said, "First we thought that the big triangle was nine square inches, but then we turned it a different way on the squared paper and realized that it was eight inches." Rotating the triangle made it possible for them to ﬁgure out how many squares it covered.

Many different sized shapes are put into order from least to largest area. | Math Solutions, Classroom Lessons

Figure 1. Andrew and Hiroshi arranged their shapes from least area to greatest, as instructed.

Two students describe how they used smaller shapes to find the area of a larger parallelogram. | Math Solutions, Classroom Lessons

Figure 2. Roberto and Laura shared the writing of their discoveries.

Two students share their process to finding the area of a parallelogram. | Math Solutions, Classroom Lessons

Figure 3. In their ﬁfth statement, Maria and Lizzie were referring to the small parallelogram, not a polygon, as they noted.

Related Publication:
About Teaching Mathematics: A K–8 Resource, Third Edition
by Marilyn Burns

From Online Newsletter Issue Number 6, Summer 2002

View Lesson

Grades 4–5

One Riddle, One Answer

"Who can explain what a riddle is?" Danielle Gilligan asked her fifth graders.

"It's like a mystery you have to figure out," Jill said.

"It has clues that help you solve it," Ramon added.

"I have a book about a riddle," Danielle said. "I'm going to read the story and stop at a certain point so that you can try to figure out the answer to the riddle."

Danielle started reading One Riddle, One Answer aloud. Her students learned about Aziza, the sultan's daughter, and how the sultan's advisors all wanted their sons to get a chance to become Aziza's husband. Danielle stopped reading at the part where Aziza proposes that she write a number riddle. The princess tells her father that she would prefer to marry the person clever enough to answer her riddle. The sultan agrees.

"I'm going to show you the riddle that Aziza wrote," Danielle said. "I've written it on a piece of chart paper. We'll read the riddle together, then I want you to think about the clues silently first. You can use scratch paper to jot down your thoughts. Then, whisper your ideas about the answer to a partner."

Danielle posted the chart paper on which she'd written Aziza's riddle:

Placed above, it makes greater things small.
Placed beside, it makes small things greater.
In matters that count, it always comes first.
Where others increase, it keeps all things the same.
What is it?

Danielle first gave students time to think and take notes. Then, as the students shared their ideas with their partners, she circulated, listening to their guesses. Some thought the answer was one; a few thought that it was zero; many were completely stumped.

Danielle then continued reading until she came to the part in the story where the scholar guesses that the sun is the answer to Aziza's riddle. She stopped at this point and addressed the class.

"The scholar's guess is true for the first line in the riddle ('Placed above, it makes greater things small')," she said, "but does that answer work for each of the other clues?"

"No," Anna responded. "It doesn't make sense for the others. The sun doesn't come first when you count."

"That's right," Danielle confirmed. "An answer to a riddle must fit all the clues, not just some of them."

Danielle continued to read. Each time a suitor in the story guessed an answer to Aziza's riddle, Danielle stopped and checked with the students to see if the answer fit all the clues. When Ahmed, the farmer in the story, guessed that the answer to the riddle was the number one, Danielle again checked with the students to see if it worked with all the clues, and it did.

"But zero works too!" Amanda cried.

Danielle was taken aback. She later confided that she hadn't thought about another possible answer. "Explain what you mean," she said.

"Well, placed above a number, it works," Amanda explained. "Like, if you put zero above five when you multiply, it makes five smaller. And it works for any number you multiply it by."

"You mean like this?" Danielle asked, recording Amanda's idea on the board:

Amanda nodded and continued with her argument. "Placed beside other numbers, it makes them greater, just like in Aziza's riddle."

"Come up and show us," Danielle urged. Amanda walked up to the board and wrote:

10 20 50

"When you put zero beside these numbers, it makes them bigger," she said. "Zero next to one makes it ten; zero next to two makes it twenty. And when you count, zero comes before one on the number line!" she exclaimed.

"And on the thermometer, zero comes before the number one," Joe added.

Elise piped in, "And for the last clue, zero keeps all things the same when you add zero to it, like zero plus one is one or zero plus ten is ten."

"I'm impressed with your reasoning. I hadn't thought about zero as an answer," Danielle exclaimed.

"I disagree," Kyle challenged. "When you count, you don't start with zero, you start with one."

"But if you're counting down the days till Christmas, on Christmas there's zero days left!" Amanda countered.

Danielle didn't interfere. Getting students to argue passionately about their ideas in math class is often difficult to achieve. Giving students interesting problems to solve, like Aziza's riddle, can motivate them to develop and exchange ideas.

When the short debate was over, Danielle acknowledged that there could be more than one correct answer to the riddle. Then she finished reading the story, including a section at the back of the book where the author explains how the number one works for each clue.

"Aziza's riddle helped us think about number riddles," Danielle said. "Let's try to write a number riddle together. First, you have to think of a number to write about. Then you have to write some clues. I want you to practice by brainstorming some possible clues for the number ten."

Danielle gave the students about five minutes to work in groups. She then called the class together and wrote some of the clues the students came up with on the board:

Count your fingers on both of your hands.
If you cut me in half and multiply me by 7, you get 35.
If you multiply a number by the mystery number, you'll always end in zero.
The age of a fourth-grader.

Then Danielle gave feedback on the clues. "When you write a riddle, the first thing you do is brainstorm a list of possible clues, just like we did for the number ten," she said. "It's OK if answering one of the clues gives away the secret number, but try to be tricky with your clues, like Aziza was with her riddle."

When the class was finished brainstorming clues for the number ten, Danielle gave the homework assignment of writing one riddle sentence for the number one-half. She explained, "Use pictures, words, and numbers to explain why the answer to your riddle sentence is one-half."

The next day, Danielle asked for volunteers to share their riddles. Some students revealed some very sophisticated thinking about one-half, while others exposed misconceptions.

Keaton shared first, "If you have a glass of water, the water hits the middle," he read, showing a picture he drew of a glass half-filled with water.

Then Nicholas read, "This number is between zero and one and if you multiply this number by two you get one." On the back of his paper, he explained why 1/2 x 2 = 1: If you multiply it by 2 you get 1 because 1/2 + 1/2 = 1. (See Figure 1.)

Here are some other clues that students wrote and shared:

I am half of 7, subtract 3.
The fraction you're guessing is greater than 1/4 and less than 1.
If you double 1/4, you get this number.

Danielle then said, "Now that you've had some experience with writing clues for the numbers ten and one-half, you're going to each choose a number, brainstorm clues for that number, and then write a riddle."

"How many clues does the riddle have to have?" Lisette asked.

"Aziza's had four clues, so try to write a riddle with at least four," Danielle responded.

Soon, the class began to hum with conversations as students shared their ideas with one another. As Danielle circulated, she encouraged students to think of subtle rather than obvious clues. After about thirty minutes, she pulled the class back together.

"Let's have some volunteers read their riddles. After they read their clues, we'll try to guess the answer."

The following are a couple of riddles that students shared (see Figures 2 and 3).

Related Publication:
Math and Literature, Grades 4–6, Second Edition
by Rusty Bresser

View Lesson

Grades 4–5

Number Relationships

Question: Here is a set of numbers: {2, 3, 5, 7, 11, 13, 17}. How are these numbers alike?

Students may offer that the numbers are all less than 20. Accept this, but push students to think about the factors of the numbers. Following are several possible responses: All have a factor of one. All have exactly two factors (one and itself). Each can be represented by two rectangular arrays. All of them are prime.

Question: What is the smallest number that has both four and six as factors?

This question has one right answer (the least common multiple for the numbers 4 and 6 is 12),but students may arrive at the answer in different ways. It's always possible to find a common multiple for two numbers by multiplying them (4 x 6 equals 24, and 24 is a multiple of both).But because 4 and 6 both share 2 as a factor, the least common multiple is less than the product of this pair of numbers. If numbers do not have a common factor, however, then the least common multiple is their product.

To help students think about these ideas, consider presenting additional questions for them to ponder:

Can you find pairs of numbers for which the least common multiple is equal to the product of the pair?

Can you find pairs of numbers for which the least common multiple is less than the product of the pair?

What do you notice about the least common multiple for pairs that have common factors?

What about pairs that do not have common factors?

Question: The weather is reported every 18 minutes on WFAC and every 12 minutes on WTOR. Both stations broadcast the weather at 1:30. When is the next time the stations will broadcast the weather at the same time?

This question provides students with a real-world context—telling time—for thinking about a situation that involves numerical reasoning. The problem also provides a problem context for thinking about multiples.

Question: Do you think that it makes sense to split a day into twenty-four hours? Would another number have been a better choice? Why or why not?

This question, which doesn't have a right answer, challenges students to think about a common, real-world application of number. You might also ask students if they think it makes sense to have an amount other than 60 minutes in each hour, perhaps 100 minutes, for example. What effect would such a decision have on how time is displayed on watches and clocks?

Grades 7–8

Question: How is each number below different from each of the others?

81 √81 36 14

List students' reasons as they offer them, encouraging them to incorporate math vocabulary into their reasons, such as divisor, factor, and divisible by. Discuss the meanings of the math terms they use and the relationships among them. For example, suppose one student says, "The number fourteen is the only number that doesn't have nine as a factor," and another student says, "The number fourteen doesn't belong because it's the only number that's not divisible by nine." Use these two statements to discuss the relationship between the terms factor and divisible by.

This question can be asked for any set of four numbers. As an extension, ask students to choose four numbers for others to consider. Then have them list all the ways the numbers differ from one another. Use their sets of numbers for subsequent class discussions. Finally, have the student who suggested the numbers describe any differences that the class didn't find.

Question: The students in Mr. Mila's class want to know how old he is. Mr. Mila told them, "My age can be written as the sum of consecutive odd numbers starting from one." How old might Mr. Mila be?

Adding consecutive odd numbers produces the sums of 4, 9, 16, 25, 36, 49, 64, 81, 100, and so on. Of these, only some are reasonable predictions for Mr. Mila's age—25, 36, 49, and 64. All of these sums, however, are square numbers. Using different-colored square tiles or by coloring on squared paper, represent square numbers as squares to help students see that they can be represented as the sum of odd numbers. Start with one tile or square colored in. Then, in a different color, add three squares around it to create a 2-by-2 square, then five squares to create a 3-by-3 square, and so on.

Talk with students about extending this pattern.

Question: Four students in Mrs. Burge's math class were comparing locker numbers. They made the following observations:

Our four locker numbers are relatively prime to one another.

Exactly two of our locker numbers are prime.

What might the students' locker numbers be?

Discuss the terms prime and relatively prime and the distinction between them. Then have students work on answering the question. Post students' answers and, for each, have the class verify whether the numbers meet the given criteria. Finally, ask students to write their own definitions of prime and relatively prime. Have them share their ideas, first in pairs and then with the whole class.

Question: For homework, Kim Lee was practicing adding integers. He looked at one problem and said, "I know the sum will be negative." Based on Kim Lee's statement, what do you know about the problem?

This question aims to help students generalize about the relationship between the sign of the sum and the numbers in an integer addition problem. Students may need to make a list of integer addition problems whose sums are negative and look for commonalities among them in order to answer this question.

From Online Newsletter Issue Number 19, Fall 2005

View Lesson

Grades 4–5

The Game of Target 300

In this lesson, fourth and fifth graders gain experience multiplying by ten and multiples of ten as they make choices about the numbers to use to reach the target amount of three hundred.

I began the lesson, "Today I'd like to share a game with you. In this game you will be multiplying by ten, twenty, thirty, forty, or fifty." I wrote the following on the board:

x 10

x 20

x 30

x 40

x 50

"You'll play with a partner. The goal of the game is to be the player closest to three hundred. It's OK to get less or more than three hundred, but the goal is to be the closest."

Allie asked, "Does that mean that one person could have two hundred eighty, and be twenty off, and the other person could have three hundred ten, and three hundred ten would win because it's closer to three hundred?"

I asked the class, "What do you think is the answer to Allie's question?" Hands went up.

Rachel said, "You said it was OK to go over three hundred. So the player with three hundred ten wins."

"You're right," I said and continued, "You'll each take six turns. When it's your turn, roll a die. Then decide if you'll multiply the number you rolled by ten, twenty, thirty, forty, or fifty. Remember, you want to get closest to three hundred, and you must take all six turns."

"Oh, this will be fun!" Steve said. "Do we have to write?"

I responded, "Yes. To show you how to do the recording part, I'd like to have a partner play the game with me." Hands immediately shot into the air. I called on Ben because I knew he had a good grasp of multiplying.

"When you play this game, each of you will need your own recording sheet," I said as I drew two two-column charts on the board, one for me and one for Ben. "I'll put Ben's name on one side of my chart and mine on the other," I said as I labeled my chart. Ben did the same on his.

I went first so I could model out loud my thinking process as well as how to record. I rolled a 1. I said, "One isn't a very large number. If I multiply one by ten that will only give me ten. I'll still have two hundred ninety to go in five turns. That seems like a lot. Maybe I should multiply by thirty; one times thirty equals thirty. Thirty is closer, but I still have two hundred seventy to go. I think I'll multiply by fifty; one times fifty equals fifty. That's closer still and it means I'm only two hundred fifty away. Do you agree that one times fifty equals fifty?" I asked Ben. Ben nodded. I recorded my turn on my side of the chart.

Once Ben had recorded my turn on his chart, I handed him the die, indicating it was his turn. Ben rolled a 2. "I'll multiply Ben handed the die back to me. This time I rolled a 4. "I am going to multiply by twenty. That gives me eighty for this turn. This time when I record, I'll record four times twenty equals eighty, then I'll add the fifty from my first turn to the eighty I just got, for a running total of one hundred thirty," I explained as I wrote my score on my chart.

"How come you didn't multiply four times fifty?" wondered David. "That would have given you two hundred. Add the two hundred to the fifty from your first turn and that would be two hundred fifty. You could almost win on your second turn."

Several students put their hands up to respond. I called on Cindy. "Each player has to take six turns. This is Mrs. Wickett's second turn and she has to take four more turns. If she got two hundred fifty by the end of her second turn, then she could only get fifty more to get three hundred! She'd have to always roll really low numbers and multiply by ten. She'd probably get some low, some medium, and some high, and go way over three hundred."

Some students didn't follow Cindy. I decided to move on rather than continue to discuss this point. "I think Cindy's idea will be clearer after you've had the chance to watch the rest of this game and play for yourself," I said. I handed the die to Ben. Ben rolled a 1.

He said, "I'm going to multiply one times thirty to get thirty. Now I have fifty." Ben recorded his turn on his chart and I did the same on my chart. He gave me the die.

"I rolled a six!" I said.

"You could multiply six times fifty and get three hundred!" Mario said. "Too bad you have to take six turns!"

"Mario is right. What would work better?" I asked the class. Hands immediately went up. I called on Allie.

"I think ten because if you multiplied six times ten that would be sixty," Allie shared. "Then you'll have one hundred ninety. Subtract that from three hundred and you still have three turns to get one hundred ten more points."

Ben and I recorded my turn on our charts. I handed the die back to Ben. He rolled a 5.

"Look, I can win!" he said. "I just multiply five times fifty. That equals two hundred fifty. Two hundred fifty and fifty is three hundred! I win unless you get three hundred too, then we would tie," Ben explained.

Several students moaned. Ben looked surprised.

"Ben, you still have three more turns! You have to take them," Leigh reminded Ben.

"Oops!" Ben said. "I forgot. I'll multiply by ten. That only equals fifty, so my total is one hundred. That's way better." Ben recorded his turn on his chart and handed me the die.

After our next two turns, I had 290 and Ben had 260. "I'm in much better shape than Mrs. Wickett!" Ben said.

"What do you think about Ben's statement that he's in better shape than me?" I asked the students.

"Well, you have to roll a one and multiply it by ten to get exactly three hundred. That's not likely," Steve said.

"Why not?" I asked.

"It just doesn't seem like that will happen," Steve said.

Rachel said, "I think all the numbers on the die have the same chance of being rolled. There are six sides on a die. One is on only one side of the die so it has one out of six chances of being rolled."

"Mrs. Wickett can only get exactly three hundred one way, by rolling a one," Tom added. "But Ben needs forty points. He could get forty by rolling a one and multiplying by forty, or getting a two and multiplying by twenty, or getting a four and multiplying by ten."

I said, "I'll roll the die and let's see what happens." I rolled and got a 3. Ben looked delighted.

"Well you just have to make the best of it and multiply by ten," Cindy said.

"That gives me a final total of three hundred twenty," I said. Giggling with delight and anticipation of getting exactly 300, Ben rolled. He got a 3.

"Oh!" he said with a surprise. "I didn't get a one, a two, or a four." He paused for a moment to think the situation over.

"Ben, you can still win," Rachel said.

"I know, I could multiply three by ten and get two hundred ninety," Ben said. The class cheered and Ben did a little victory dance. I waited for a few moments for the students to settle down and then showed them what else to record when they played. I wrote on the board under my chart:

Ben won.

Ben was 10 points away from 300.

Mrs. Wickett was 20 points away from 300.

I also wrote prompts for students who might need them:

__________ won.

__________ was __________ points away from 300.

The students played the game with great enthusiasm and involvement as partners participated in every turn.

Related Publication:
Teaching Arithmetic: Lessons for Extending Multiplication, Grades 4–5
by Maryann Wickett and Marilyn Burns

From Online Newsletter Issue Number 4, Winter 2001–2002

View Lesson

Grades 4–5

One Time Only

Upper elementary students beneﬁt from activities that help reinforce their understanding of factors, multiples, and prime numbers. In this two-person game, students take turns identifying factors of successive numbers, continuing until one of them can no longer contribute a new number.

Directions for Playing the Game

Player 1 writes down a number greater than one and less than 100.
Player 2 writes down a factor of the ﬁrst number underneath it.
Player 1 writes down a factor of this new number.
Each player, taking turns, writes down a factor of the preceding number.
If a player writes down a prime number (i.e., it is not divisible without a remainder by any other integers except one and itself), the next player adds seven to it and writes down the resulting sum as his or her turn.
The player who can no longer contribute a new number loses the game.

Additional Rules

Once a number has been written down, it can't be used again.
The number one can't be used at all.

Caren Introduces the Activity

"Today I have a game for you," I announced to the sixth graders in Lyndsey Lovelace and Shea Carrillo's class. "It's called One Time Only. To play the game you need a partner. One of the partners begins by picking a number greater than one and less than 100. So you can see how it works, the whole class will be my partner for this ﬁrst game. There are just a few rules, and I'll explain them while we play."

I wrote 36 on the overhead.

"Now it's your turn," I said. "You need to think of a factor of 36. Can anyone tell me a number that goes evenly into 36? Another way to think about it is by skip counting. Which numbers can you skip count by and get to 36?"

By introducing several ways to think about factors, I hoped to explain the game more quickly. If I'd just asked for a factor of 36, students who weren't sure what a factor was or who weren't sure about the difference between a factor and a multiple might not have been able to participate. As the students used the terminology in the context of the game, they'd become more comfortable with it.

"So, what do you think?" I asked. "Can anyone tell me a factor of 36?"

"How about six?" offered Fred.

"Is six a factor of 36?" I asked the class.

Several students nodded or vocalized their assent. I pushed for more of a commitment. "Who can explain why they think six is a factor of 36?" I asked.

Jessie raised her hand. "Because six times six is 36," she explained.

"Also," added José, "if you count by sixes you'll say 36. Like 6, 12, 18, 24, 30, 36."

"All right," I said, "I'm convinced that six is a factor of 36." I wrote 6 under 36 on a projected transparency. "Now I need to ﬁnd a factor of six to add to the chain of numbers we're making here. I think I'll say two." I wrote 2 under the 6. "Okay, now it's your turn to think of a factor of two," I said.

"Two," said Derek. "Two times one is two."

"Well, yes," I responded, "two is a factor of itself, but one rule is that you can't use the same number twice. That's why the game is called One Time Only. If a number is already written down you can't use it again. Can anyone think of a factor of two that's not already up here?" I asked, pointing to the overhead.

"One," said Ali.

"Well, that brings up another rule in One Time Only. You can't use one. You're correct, Ali, that one is a factor of two. But in this game you're not allowed to use one. So you can't use a number that's already up there and you can't use one. Those are the two main rules of this game. Can you think of any other factors of two?"

"How about four?" asked Chrissy.

"How do you know four is a factor of two?" I inquired.

"Because two times two equals four," Chrissy explained. Chrissy had confused factors and multiples. I was glad she had made the multiplication connection, but I needed to prompt her a bit to get her back on track.

"I know that two is a factor of four, because I can count to four by twos," I said to her. "But it doesn't work the other way around. Four isn't a factor of two, because you can't count to two by fours."

"Oh, yeah," Chrissy replied.

"Does anyone know what we call four in this situation?" I asked the class.

"A multiple!" exclaimed Neal. "If you can times a number to get the number it's a multiple. Like 36 is a multiple of six because six times six is 36."

"All right," I continued, "so are there any factors of two besides two and one?"

"Can we use fractions?" asked Howard.

"Sorry," I told him, "but factors need to be whole numbers, like the numbers you use to count. Take a minute and talk at your tables," I suggested. "See if you can think of any other factors of two."

I let the students talk brieﬂy and then I called them back to attention. "Did any tables ﬁnd any other factors of two?" I asked. The class consensus was no. "So, do you think there are any other factors of two?" I prodded, checking to see if the students were really convinced.

"Not if we can't use fractions," Ana qualiﬁed.

"Well," I told the class, "you're right. There are only two factors of two, two and one. Does

anyone know what you call a number that only has itself and one as factors?"

"Prime?" Greg ventured in a barely audible tone.

"Prime!" several students announced with authority after hearing Greg.

"Yes, those are prime numbers." I wrote prime next to 2 on the overhead. "In One Time Only when you hit a prime number you add seven to it. So what's two plus seven?"

"Nine," several students responded.

I wrote 9 on the overhead under the 2.

"Okay, now it's my turn, and I need to think of a factor of nine. I'll say three," I said, as I added 3 to the list on the overhead. "Now you need to ﬁnd a factor of three."

"It's prime," announced Natalie with authority.

"If it's prime, what happens?" I asked.

"Add seven," José reminded us. "So it's ten."

I wrote prime next to the 3 and put a 10 below it.

"How many times can you use plus seven?" Jessie asked.

"There's no limit," I explained. "Anytime you're playing and a prime number comes up, you just add seven to it. It's my turn again and I need to put a factor of ten that's not already listed. I'll say ﬁve."

"Oh no," exclaimed Alejandro, "another prime number for us."

I raised my eyebrows in feigned surprise as I looked at the numbers on the overhead. "Wow, it is a prime number." I agreed. "You keep getting prime numbers on your turn. I wonder if that always happens in this game. Maybe there's a pattern." While I knew that this particular pattern didn't always happen, I took the opportunity to spark a little curiosity. I hoped that in subsequent games students would pay more attention to patterns in general as they played. Looking for patterns is a powerful way to build number sense, particularly when students have opportunities to think about the patterns and their relationships to numbers and operations. I referred to the string of numbers on the overhead, which now looked like this:

2 prime

3 prime

5 prime

"Okay," I said to the class, "it's your turn and since ﬁve is prime, what do you need to do?"

"Add seven," Jasper replied.

"Right," I agreed, "so now it's 12." I wrote 12 on the overhead. "Hmm," I said, "I need to ﬁnd a factor of 12 that's not already up here." I paused for a few seconds to give students a chance to review the numbers and think about factors of 12. I also wanted the students to see that math involves taking time to think.

"I know," I brightened, "I'll say four." I wrote 4 on the overhead beneath the 12. "Now you need to ﬁnd a factor of four that's not already up here. Talk at your tables for a minute or two and see what you can come up with."

"We're stuck," Ali soon announced.

"What do you mean?" I asked.

"Well," Fred explained, "we're not allowed to use one. Four and two are used already. There are no other factors, so we can't go."

"Does everyone agree with Fred and Ali?" I asked, looking around.

The nods and yeahs were unanimous.

"Then I guess the game is over," I said. "This time I won, because I was the last player to add a number to the list. You want to get your partner stuck so she or he is unable to add a number to the string. But winning isn't really the important part of this game. You're going to play a bunch of times, and sometimes you'll win and sometimes you'll lose. The important part of the game is the mathematical thinking that you do."

I played one more game with the whole class. This second game went more quickly, because I didn't need to stop to explain the rules and vocabulary. After two games, I was satisﬁed that the students understood the rules and knew how to determine a winner. The factor concept had been reinforced, the term multiple had been introduced in context, and the students knew how to identify prime numbers. The students were ready to play with their partners. In addition to having practice with multiplication facts, students who play One Time Only search for winning strategies by thinking about relationships among numbers and factors. In so doing, they build their number sense.

From Printed Newsletter Issue Number 26, Fall/Winter 1999-2000

Related Publication:
Developing Number Sense, Grades 3–6
by Rusty Bresser and Caren Holtzman

View Lesson

Grades 4–5

Volume of Rectangular Prisms

In previous lessons, students built rectangular prisms using cubic units and determined the volume of the prisms by counting cubes. Students started to devise methods for finding the volume of any rectangular prism without counting. In this lesson, students continue their work on developing a method for determining the volume of any rectangular prism. They share their methods with each other and discuss similarities and differences between the methods. One goal of the lesson is to help students articulate how volume can be determined by finding the number of cubes in each layer of a prism. If students know the number of cubes in one layer, they can multiply that amount by the number of layers, or height of the rectangular prism. The method of multiplying the dimensions is then connected to the idea of layers.

Professional Development Sessions

1.2, 1.3, 1.4, 1.5, 6.2, 7.2, 8.3

Video Clips

Refer to the demographics table in Classroom Discussions: Seeing Math Discourse in Action, Grades K–6, page xxxvi.

Time

approximately 45 minutes

Materials

interlocking cubes
3-by-4-by-5 rectangular prisms, 1 per group of 3–4 students

Vocabulary

cubic units, rectangular prism, volume

Common Core State Standards for Mathematics

Measurement and Data: Standard 5.MD Understand concepts of volume and relate volume to multiplication and addition.

Identifying the Mathematical Goals

Students will:

learn a method for finding the volume of a rectangular prism based on the number of layers in the prism
connect the method of multiplying the dimensions to finding the number of cubes in the base layer (l x w) and
multiplying by the height h
review the units used when determining volume of rectangular prisms (cubes or cubic units)

Anticipating Confusion

Students may confuse measuring volume with measuring surface area.
Students may not visualize a prism as consisting of layers of cubes.
Students may refer to volume devoid of units of any kind and not realize the importance of the type of unit.

Asking Questions

What does it mean to find the volume of a prism?
How can we use multiplication to find the number of cubes in one layer of a rectangular prism?
How can we use multiplication to find the total number of cubes in a rectangular prism?
Explain why your method works to find volume.
How is multiplying the dimensions to find the volume similar to using the layers approach?
What type of units are needed to measure volume? Why do we use that unit instead of others such as . . .
(fill in depending on response from students).

Planning the Implementation

1. Begin this lesson by reviewing the terms rectangular prism, volume, and cubic unit. Ask students, "What does each term mean?" Conduct a brief whole-class discussion about each definition.

2. Connect to the previous class periods where students spent time finding the volume of rectangular prisms: "Over the past few days, many of you have developed methods for finding the volume of any rectangular prism. Today, I'd like for us to talk as a whole class about our methods." Write the following where everyone can see it: Method for finding the volume of a rectangular prism. Say, "As a group, develop a method for finding the volume of a rectangular prism." Let students work in groups of three or four for about eight to ten minutes. Give the groups interlocking cubes to help them develop their methods.

3. Post each group's method where everyone can see it. Withhold comments or corrections. If groups have identical methods, post the methods both times.

4. Say, "We have several different methods. Before we think about whether they work for all prisms, let's test them with one prism. With your partner, see if you can apply each of the methods displayed to a three-by-four-by-five-unit prism. If you get stuck, think about how you might edit the method so that it works. If the method does work, think about why it works and whether it will work for all rectangular prisms." Give students time to do this with their partners.

5. Conduct a whole-class discussion to discuss certain methods.

a. Briefly discuss which methods may need a little revision or editing. If a student makes a suggestion on how to revise a method that he or she did not write, check back with the students who wrote it. For example, "What do you think about that suggestion? Do you think it makes sense to add it to what you wrote? Why?"

b. Pick the methods you would like everyone to discuss based on the mathematics. Namely, focus the discussion on methods that involve layering—finding the number of cubes in one layer by multiplying length × width, then multiplying this by the number of layers, which is the height of the prism. Any time a student uses language associated with layering, ask at least two other students to repeat it. Ask students if they agree or disagree that the method would work for all rectangular prisms and why.

c. If a group has suggested multiplying length × width × height, first have students discuss why this method works. Ask other students to explain why they agree or disagree that this method would work for all rectangular prisms. Then ask, "How is this method similar to the layering method? How is it different?" Share students' ideas.

6. Summarize the key mathematical points. Say, "We have discussed several different methods but all of these methods have one thing in common. They all involve finding the volume by determining the number of cubes in one layer and then multiplying that by the number of layers."

Featured in Math Solutions Online Newsletter, Issue 39

View Lesson

Grades 4–5

Great Estimations

In this lesson, the marbles and beans, along with the book Great Estimations by Bruce Goldstone, are used to provide students with an opportunity to explore and apply strategies for estimation. Also, collections of grid paper with different-sized grids, measuring cups of various sizes, a few unifix cubes, and balance scales and masses provide engaging estimation tools for students.

Preparation

Before class, place one cup of kidney beans in each quart-size sandwich bag, filling enough bags for one bag per pair of students. Place the bags of beans in a large paper bag along with a pint-size and quart-size jar of marbles. Make sure you've counted the marbles in each jar.

Materials

quart-size zip top sandwich bags, 1 per pair of students
kidney beans, 1 cup per pair of students
pint-size jar of marbles
quart-size jar of marbles
large paper bag
the children's book Great Estimations by Bruce Goldstone (Square Fish, 2010)
collections of grid paper (different-sized grids such as inch, half-inch, centimeter), measuring cups (various sizes), unifix cubes, and balance scales and masses

Lesson Outline

Focus or Warm-Up

Place the large paper bag on a table. Pull the pint-sized jar of marbles from the bag. Hold it so all students can see it. Ask students, "About how many marbles would you estimate are in the jar? To estimate means to make your best guess."
Listen as students make various guesses out loud. Write on the board Estimates and beneath it record the suggestions. This might inspire a few additional estimates.
Ask students to share their reasoning.

From Maryann's Classroom:

In my class, Tuheen shared, "Some marbles are hidden so I don't think I can make a good guess. I think there are more than twenty-three marbles because I could get about ten marbles in a handful and I know there are more than two handfuls in the jar. Two tens equal twenty so there must be more than twenty-three. But I don't know how many handfuls there could be. I was trying to think of how many of my fists could be in the jar, then I remembered that my fist is bigger than the handful so that won't work." Isabela shared, "I tried to picture the marbles if I dumped them out. It would be a big mess and it didn't help me think about how many, only about the mess!"

Introduction

4. After a moment, say something like this to your students, "Today we're going to explore a book about estimating called Great Estimations by Bruce Goldstone. As I read the book, listen carefully for strategies you could use to estimate. When you hear a strategy, tell me and I'll record it on the board. Later, we'll use one of the strategies to help us estimate the number of marbles in the jar. Finally, you'll have the chance to work with a partner to make some estimates."

5. Begin by reading aloud and sharing the illustrations on pages 3, 4, and 5.

From Maryann's Classroom:

Yainid shared the first strategy. "We have to see what ten of something looks like, then try to figure out how many tens." I recorded Yainid's suggestion on the board. Tait said, "Hey, Yainid's idea is sort of like Tuheen's idea. He thought about ten marbles, like the book says, but then he couldn't figure out how many tens." Several students nodded in agreement.

6. Continue reading and recording estimation strategies as students notice them. Following is the list of strategies students may suggest:

Possible Strategies

Find what ten looks like.
Find what one hundred looks like.
Find what one thousand looks like.
Compare what you are estimating to ten, one hundred, or one thousand.
Make equal rows.
Weighing.
Clump counting.
Box and count.
Layers.

Exploration 1

7. Ask students to choose strategies from the list to estimate the number of marbles in the jar. Record their reasoning on the board as appropriate.

From Maryann's Classroom:

Gaby thought making rows of ten would be helpful. I asked her to make a row of ten. Kaitlin said, "I noticed that when Gaby took out ten marbles it made a difference in the level of marbles in the jar. I think if we took out another ten marbles the jar would be about one-fourth empty." Garrett chimed in, "If Kaitlin is right, we could multiply twenty by four. I used twenty because that would be how many marbles were taken out of the jar for it to be one-fourth empty. I multiplied by four because there are four-fourths in the whole jar. That would be a good estimate of how many marbles were in the jar when it was full."

I asked Kaitlin to try her idea for us by taking out ten more marbles to make a total of twenty marbles removed from the jar. The jar looked like it was more than three-fourths full so Kaitlin took out six more marbles for a total of twenty-six marbles. We agreed that it now looked about three-quarters full or one-fourth empty. Kaitlin said, "Using Garrett's idea, I think there are one hundred four marbles in the jar. Twenty-six plus twenty-six equals fifty-two so there are fifty-two marbles in one-half. Fifty-two and fifty-two equal one hundred four marbles." I recorded on the board:

26 + 26 = 52

52 + 52 = 104

Austin said, "I agree with Kaitlin, but I changed twenty-six to twenty-five by subtracting one. I know that four twenty-fives is one hundred. It's like quarters in a dollar. Then I added four more because I changed twenty-six to twenty-five. I have to put back the one. Four times one equals four. One hundred plus four equals one hundred four." I recorded:

26 – 1 = 25

4 × 25 = 100

4 × 1 = 4

100 + 4 = 104

Safaa shared, "In my brain, I changed twenty-six to twenty plus six. Then I counted, 20, 40, 60, 80. I put the eighty in my memory. Then I counted 6, 12, 18, 24. I took the eighty out of my memory and added eighty and twenty-four. That's one hundred four." I recorded on the board:

26 = 20 + 6

20, 40, 60, 80

6, 12, 18, 24

80 + 24 = 104

8. Refocus the students' attention on the initial estimates shared. Ask the students, "How does our class estimate compare with the first estimates shared?"

From Maryann's Classroom:

In my class, our initial estimates were 1000, 23, 500, 145, 1,000,000, 779, 333, and 2057. Our class estimate was 104. Tim said, "Most of the first estimates are a lot bigger than what we just figured out."

"I just picked a number," said Rocio.

Jose offered, "When we had a way to think about it we could make a better estimate than just picking a number."

9. Finally, tell the students how many marbles you had counted when you filled the jar. To verify your count and to reinforce the value of using a strategy to make an estimation, count the marbles by placing them into groups of ten.

From Maryann's Classroom:

I had counted 112 marbles in the jar. My class counted 11 groups of ten with two extra to figure this out. The class strategy for estimating 104 marbles got them very close to the actual total of 112 marbles.

Exploration 2

10. Next, pull the quart-sized jar of marbles from the bag and hold it up. Ask, "Here is a quart-sized jar of marbles. How can you use what you know about the number of marbles in a pint to help you estimate the number of marbles in a quart?"

From Maryann's Classroom:

Malachi shared, "There are two pints in one quart. So if we know how many in a pint we can just double that to get a good estimate for how many in a quart." Using this information, the class quickly concluded that a quart jar would hold about 224 marbles: 2 × 112 = 224.

Exploration 3

11. Show students the bags of kidney beans, explaining that they'll be working with a partner to use a strategy for estimation from the book to estimate the number of beans in their bag.

12. Hold up the estimation tools: grid paper, measuring cups, unifix cubes, and balances and masses. Ask: How might these tools help you carry out the strategies listed on the board?

From Maryann's Classroom:

In my class, the students shared that the grid paper could be used with the box-and-count strategy, the balance and masses could help with the weighing strategy, and the measuring cups could help with thinking about how many tens or hundreds fit in a measuring cup or even a unifix cube if the items were small like popcorn or lentils.

Katie had a new idea. She suggested that we could use the small geoboard rubber bands as a way to help with the clumping strategy. The class agreed this was a good idea and geoboard rubber bands were added to the estimation tools.

13. Give partners time to work together on recording their estimates and notes about what tool they used to make their estimate. When students finish, ask them to record their information on a class chart:

Estimation Tool Estimate

groups of ten to figure 104

how many in ¼ pint

Summary

14. Gather students for a discussion. Focus the discussion on the similarity of the estimates. Each bag had approximately the same number of kidney beans. In most cases, the estimates were similar even though partner pairs used different estimation tools. This usually delights the students.

From Maryann's Classroom:

The students in my class concluded that differences in the estimations came from varying sizes of beans, how precisely the beans filled an area of measurement, how carefully people measured and counted, and how the number of beans in the bags varied.

Rylie asked, "Can we do this again tomorrow using different bags of stuff?" I nodded my head. I had already prepared bags of objects: quart-size bags of lima beans, cotton balls, teddy bear counters, macaroni, real pennies, and popcorn, along with snack-size bags of plastic counters, cotton swabs, base ten unit cubes, paper clips, and lentils.

Featured in Math Solutions Online Newsletter, Issue 37

View Lesson

Grades 4–5

Post-Assessment Tasks for Grades 3–5

Open-ended problems can make for excellent post-assessment. Wondering how you can design effective post-assessment tasks for your students? This lesson gives a four-step plan, including a 3–5 sample task and corresponding authentic student responses. The lesson is adapted from Math for All: Differentiating Instruction, Grades 3–5, by Linda Dacey and Jayne Bamford Lynch. Visit www.mathsolutions.com to learn more.

Step 1I: Design a Task That Will Capture a Broad Range of Responses

Open-ended tasks allow students to control some of the difficulty level themselves. In the example task below, students may limit their consideration to only a few shapes or by focusing exclusively on two-dimensional shapes. Similarly, students may choose to use drawings, charts, or diagrams to communicate their ideas, or they may rely more on prose.

Sample 3–5 Task

What do you know about shapes? Write and draw to communicate your ideas.

Step II: Talk with Students About Task Expectations

As a whole class, create a list to guide students' work on the task.

Sample 3–5 List

Focus on shapes.
Use words and drawings to explain what you know.
Use geometry vocabulary.
Organize your ideas.
Give several samples.
Think about real-world connections.

Step III: Have Students Respond to the Task

After a brief discussion about the task expectations, students are normally eager to begin their task. Some students might think for a minute or so before beginning to record their ideas, but most begin immediately. Following are examples, including authentic student work, of how students responded to the above task.

Some students used shape templates, while others preferred drawing freehand. Most students began by drawing a shape on their paper and then writing some words above or below it. A few students began by writing an idea or the name of a shape, which they then illustrated.

Response Sample 1: Two-Dimensional Shapes

Third grader Lisa's response focused on two-dimensional shapes. She classified shapes by their number of sides and provided the correct name for three-, four-, five-, six-, and eight-sided shapes. Though she did not name the shapes that she drew within her quadrilateral category, she did include a trapezoid, a square, and a parallelogram. She provided one example of a triangle, a pentagon, and a hexagon.

Third grader Lisa's response

Fourth grader Tai included references to concave shapes and polygons, and made connections between two-dimensional and three-dimensional figures. He also introduced pyramids, right angles, and the term parallel. He was excited as he worked. He recorded one idea and then his eyes lit up as he thought of another. As these ideas were not necessarily related, he often recorded a thought and then drew a ring around it to separate it from his other recordings.

Fourth grader Tai's response

Rafael's response was particularly interesting. Rafael was a strong visual learner. He often made diagrams to summarize the events in a story and his language often reflected his visual preference. Just that morning the teacher was listening to another student explain to Rafael why they should play soccer that afternoon instead of going on a bike ride. After Rafael heard the other student's reasoning, he replied, "OK, I see what you mean." Along with illustrating and labeling many geometric shapes and concepts, Rafael drew tools that he associated with geometry. Note his two representations that connect metric and English units along with the geoboard, compass rose, and protractor.

Fifth grader Rafael's response

Step IV: Review Students' Responses and Plan Next Steps

Discover what each student chose to include; perhaps it is what he knows best, or what she believes is most important, or what he finds most interesting. Also note what concepts students did not provide evidence for, or for which the evidence is

incomplete or inaccurate. Share your findings with other teachers. Look at the similarities and differences across grade levels. Following are observations and plans that teachers made upon reviewing responses to the sample task.

Sample Teacher Reviews and Plans

Teachers were amazed at the differences across the grade levels. The third-grade teacher was surprised by how much more complex the students' responses were in the upper grades. He noted that in his third grader Lisa's response (see Response

Sample 1), the sides within her triangle, pentagon, and hexagon had approximately the same length and the figures were drawn with a base parallel to the bottom of the page. Such orientations are common; in fact, many students do not identify some of these figures when their sides are not congruent or when they are not placed in traditional positions.

The teachers decided to include this work in students' portfolios. Next year, they wanted the students' teachers to have these artifacts to help them determine readiness for future work in geometry. They were also interested in watching the evolution of students' work over time. Perhaps they could repeat the assignment later in the year, and the next year they might use it as both a pre- and post-assessment.

Featured in Math Solutions Online Newsletter, Issue 32

Related Publication:
Math for All: Differentiating Instruction, Grades 3–5
by Linda Dacey and Jayne Bamford Lynch

View Lesson

Grades 4–5

A Remainder of One

I began the lesson by writing on the board: 48 ÷ 3. "What does this mean?" I asked. "I'm interested in all of your ideas." The students had a variety of ideas.

"It says forty-eight divided by three," Mason said.

"It means how many times can three go into forty-eight," Noelle added.

Emma suggested a word problem. "Suppose you have forty-eight apples and you share them among three people," she said. "Then you have to figure out how many apples each person gets."

"I know that the answer is sixteen," Clay said.

"How do you know that?" I asked.

Clay responded, "Because sixteen and sixteen is thirty-two, and then if you add sixteen more you get forty-eight."

"It's like three times sixteen is forty-eight," Jason added. I recorded the boys' ideas numerically.

48 ÷ 3 = 16

3 x 16 = 48

Next Greg said, "If you have forty-eight things and you put them in three baskets, then you figure out how many are in each basket."

No one had mentioned that the groups had to be equal, an important idea when thinking about division, and I decided to use Greg's suggestion to push that idea. I drew three baskets on the board and asked Greg, "So you would divide the forty-eight things among these three baskets?" He nodded. I wrote the number 40 in the first basket and the number 4 in each of the other two.

"Is this OK?" I asked. Some of the students giggled.

"No," Brooke said. "You have to have the same amount in each basket." The others nodded.

"You mean that the groups have to be equal?" I asked, paraphrasing Brooke's idea to use the terminology of equal. The students nodded again.

"Yes, that's very important," I confirmed. "When we talk about division, it's important that we're talking about dividing things into equal groups." I erased the numbers I had written in the baskets and wrote 16 in each.

All of the students' ideas had related to sharing forty-eight into three groups; none of them had offered a suggestion about making groups of three. I gave a stab at this other interpretation of division—dividing forty-eight into groups of three, rather than into three groups. I asked, "What about if there were forty-eight people and I asked them to organize themselves into groups of three? How many groups would there be?" Some hands shot up immediately. I waited until more students raised their hands, and then I asked everyone to say the answer together, quietly.

"Sixteen," they responded.

"It's the same thing," Brooke said. "You just have to divide forty-eight by three."

"Suppose these are people," I said, drawing three small circles on the board in a group. I continued drawing until I had sixteen groups of three circles.

"But this is different than sharing forty-eight apples among three people," I said, pointing at the three baskets with the number 16 written in each.

"They both work," Noelle said. The others nodded, but I'm not sure they all understood that there are two ways to think about division—sharing (or partitioning) and grouping. Danielle, the students' teacher, said that she'd been having trouble getting them to think about division as grouping, that the contexts they offered generally related to sharing. More experience with grouping contexts would be useful for this.

Shifting the Conversation to Include Remainders

I then wrote on the board: Remainder of 1.

I asked, "Can you think of any division problems that have a remainder of one in the answer? Turn and talk to your neighbor and see if you can think of any." The room got noisy as the students conferred. After a moment, I called them to attention and had them report. They came up with a long list that included problems like the following:

10 ÷ 3 = 3 R1

8 ÷ 7 = 1 R1

11 ÷ 5 = 2 R1

25 ÷ 8 = 3 R1

5 ÷ 4 = 1 R1

Mason noticed that if the dividend was one more than the divisor, as in 8 ÷ 7 and 5 ÷ 4, the answer was always 1 R1. Then the list evolved into problems that always had an answer of 1 R1:

7 ÷ 6 = 1 R1

12 ÷ 11 = 1 R1

9 ÷ 8 = 1 R1

100 ÷ 99 = 1 R1

1,000 ÷ 999 = 1 R1

1,000,000 ÷ 999,999 = 1 R1

To make a shift from problems with this attribute, I asked them to think of problems that had the answer of 2 R1. The list they came up with included these:

7 ÷ 3 = 2 R

13 ÷ 6 = 2 R1

9 ÷ 4 = 2 R1

15 ÷ 7 = 2 R1

5 ÷ 2 = 2 R1

I continued until I had listed about a dozen or so problems. As I recorded, I noticed that the first numbers were all odd. I shared this with the class. "What I notice about these," I said, "is that the first numbers in the problems, the dividends, are all odd. I wonder if it's possible to get a quotient of two remainder one if the dividend is even, say ten?" I wrote on the board:

10 ÷ ? = 2 R1

I hadn't thought about this before, which is always a risk when posing a problem to a class. But after some students made a few unsuccessful tries, Alexis came up with the answer of dividing by 4 12 . I wrote her idea on the board:

10 ÷ 4½ = 2 R1

Alexis explained, "If you want a remainder of one, you have to find a number that you can multiply by two and get nine."

"Why nine?" I asked.

Alexis replied, "Because if you have a problem where you divide nine by a number and get two, then if you divide ten by the same number, you'll have a remainder of one."

Then I asked what would happen if I changed the problem. I wrote on the board:

100 ÷ ? = 2 R1

At this point, a troop of parents came into the room. They were on a school tour, getting ready for their own children to go to kindergarten. I explained the problem we were working on and invited them to try to solve the problem with us, which produced a few looks of panic in their eyes. I assured them that it would be a few years before their children brought home math work like this.

The students made several false starts in thinking about this answer and we analyzed each of their suggestions before we decided that 49½ was right.

From Online Newsletter Issue Number 20, Winter 2005–2006

View Lesson

Grades 4–5

Guess My Number 3-5

Guess My Number invites children to consider the structure of the number system while engaging in a logic game. Students try to guess a secret number from within a given range of possibilities. Guess My Number also presents an opportunity to reinforce mathematical symbols such as the "greater than" and "less than" signs. Through this activity, students learn the usefulness of number lines as tools for solving problems. Guess My Number appears in Rusty Bresser and Caren Holtzman's Minilessons for Math Practice, Grades 3–5 (Math Solutions Publications, 2006).

As Patty Stark's fifth graders settled into their seats, I went to the board and drew a box with a question mark inside it.

"Good morning," I greeted the class. "I've got a secret number for you to guess. Since it's early in the morning I'm going to make it pretty easy for you. I'll tell you the number is somewhere between one and one hundred."

"Could it be one hundred?" Martina asked.

"Yes," I responded. "It could be any number between one and one hundred, including one or one hundred. You can guess a number and I'll tell you if my secret number is greater than or less than your guess."

I drew a number line on the board to help the students keep track of their guesses.

"How about fifty?" Latoya asked.

"The secret number is less than fifty," I told her as I wrote this information on the board. I also marked 50 on the number line with an arrow, indicating all the numbers fifty and above were too large.

Before I took any more guesses I decided to have a brief discussion about strategies for guessing. I had deliberately picked an easy number to start with so we could focus on the mechanics and thinking involved with the game.

"I'm a bit curious, Latoya," I said. "Is there a particular reason you chose fifty?"

"Yeah," Latoya responded. "I knew your number was between one and one hundred, so I picked fifty because it's in the middle."

"So how does that guess help you?" I pushed.

"Because it splits the numbers. Since you said the secret number is less than fifty, I know it's in the bottom half of the numbers."

I summarized Latoya's strategy with accompanying references to the number line. "I think I get it," I told the class. "All the possible numbers are between one and one hundred. So if you guess a number right in the middle, you can figure out which half the secret number is in and then you can just throw away the other half and not have to worry about it."

I proceeded to take some more guesses. Within a minute the number line looked like this:

I then asked students to pair up and briefly discuss two things—what they thought they already knew about my secret number and what number they'd like to guess next. I wrote the two prompts on the board to help them stay focused. Also, I was giving them a preview of the discussion to come.

What do you already know about my secret number?

What next guess would you like to make? Why?

After a few minutes I called for the students' attention. "So can anyone tell something you know about my secret number?" I asked.

"It's more than forty," Hilario offered.

"It's between forty and fifty," David added.

"It's in the forties," Brenda posited.

"So you already know a lot about the secret number," I validated. "With all this information, what number would you like to guess next? If you raise your hand to guess a number, you also have to be willing to explain why you think that number is a helpful guess."

I called on Destinee. "Forty-five," she volunteered.

"Why is forty-five helpful?" I asked.

"Because," Destinee explained, "we know the secret number is in the forties, and forty-five is in the middle of the forties."

"It's just like Latoya's idea," Reynaldo chimed in. "You can get rid of half the numbers that are left."

"Aha," I responded, "so you're using your logical thinking to help eliminate a bunch of possibilities with one guess. Well, I'll tell you that the secret number is less than forty-five. Talk to a partner again about what you know about the number now and what guess you'd like to make next."

I let the students talk to each other as I added the new information to the number line. Many students wanted to use the same strategy and pick the number that was halfway between 40 and 45. We took a brief detour to establish that 42.5 was the midpoint between 40 and 45, but I explained that this Guess My Number game involved whole numbers only, so they needed to choose either 42 or 43.

Christina guessed forty-two. I told her that the secret number was greater than forty-two and recorded this information on the board. Then Kenny guessed forty-nine. Some students expressed frustration with his guess since they already knew the number was less than forty-five. I stopped briefly to have a talk about maintaining a safe environment.

"This is a new game we're playing today," I told the class. "Part of learning and trying new things is making mistakes. It's really important that everyone in the class feels safe enough to share his or her ideas and sometimes make mistakes. That's how we learn. If you disagree with someone or you have a different idea, that's fine. Just make sure you communicate that in a way that won't hurt anyone's feelings. Do you know what I'm talking about?" I asked the class as I looked at each student.

"Yes," the students murmured.

I added Kenny's guess to the board and moved on.

"How about forty-three?" Lisa suggested.

I wrote 43 on the board and circled it.

"Yes," I congratulated. "My number is forty-three. I'm impressed with everyone's thinking. It could have been any of one hundred different numbers, and it took you only eight guesses to get it. That shows you used a lot of good mathematical thinking."

Before I left the class, I called on a pair of students to lead the class in another round of Guess My Number.

Extending the Activity

Guess My Number works equally well with fractions, decimals, or percents. Giving the students some visual tools is essential. Using a number line helps students compare numbers and order the numbers.

A 1–100 chart is another tool that works nicely for Guess My Number. Tell students that the secret number is somewhere on the 1–100 chart. Cross numbers off the chart as they are eliminated. Familiarity with a 1–100 chart gives upper-elementary students a distinctive edge when it comes to mental computation and understanding our number system. When students have a visual model of the chart in their heads, they can easily jump around using tens. They also have a useful geometric model (the 10-by-10 square) to get a feel for how numbers are related to one another. Playing Guess My Number with a 1–100 chart gives students further exposure to the chart and pushes them to articulate some of the number relationships inherent in it.

Featured in Math Solutions Online Newsletter, Spring 2007, Issue 25

Related Publication:
Minilessons for Math Practice, Grades 3-5
by Jennifer M. Bay-Williams and Sherri L. Martinie

View Lesson

Grades 4–5

The Three-Store Problem

A man goes into a store and says to the owner, "Give me as much money as I have with me and I will spend $10." It is done, and the man does the same thing in a second and third store, after which he has no money left. How much did he start with?

The article explains that the problem was given to a class of eight-year-olds. The children worked on the problem over a number of days, and their teacher reported that "after a while the mathematics got too difficult for some of the children." Still, they all continued to participate in different ways, and some children were able to reach a solution. Marilyn Burns presented the problem to a class of fifth graders.

I was intrigued by the problem and before the lesson, as the article suggested, I took the time to solve it myself. (I suggest that you do this, too.) Then, to begin the lesson, I gathered the students on the rug and showed them the journal. "I found a problem in this issue that I thought was interesting," I told them. "I'll be interested in your reaction to whether this problem is too easy, just right, or too hard for fifth graders."

I read the problem aloud and said, "Please think about this by yourself for a moment. As you're thinking, I'll write on the board what the man said to each of the three store owners." I wrote on the board:

Give me as much money as I have with me and I will spend $10.

A few of the students had questions about the problem. "Did he give all of his money away after the third store?" James wanted to clarify.

"Yes," I confirmed.

"And he spent ten dollars each time?" Keely asked.

"Yes," I said, "he spent ten dollars in three different stores." I added to what I had written on the board:

Give me as much money as I have with me and I will spend $10.

Store 1

Store 2

Store 3

I then said to the students, "Turn and talk with your neighbor about how you might approach solving the problem. I'll interrupt you in a few minutes so that we can share ideas." The room became noisy as the students began to talk.

After a few moments, Scott rushed up to me. "I think I know something about the third store," he said. "The guy would have to have five dollars when he went in there, because if the storekeeper gave him five dollars, then he would have ten dollars. And then when he spent ten dollars, he'd be broke." Scott grinned and then sat back down next to Gabriel, his partner.

Next, Mara and Natanya came up to me. Mara had a question. "Did he have to spend ten dollars at the last store?" she asked.

"Yes," I replied.

"See, I told you," Natanya said.

"But what if he didn't have ten dollars to spend, even after the storekeeper gave him the money?" Mara persisted.

"That would have been a different situation," I responded. "In this story, the man had enough money each time to spend ten dollars, but after he spent ten dollars at the third store, he had no money left at all." Mara seemed to accept this explanation and she and Natanya sat down together again.

Soon I interrupted the students for a whole-class discussion. "I think it will be useful to hear one another's ideas," I told them. "Let's talk about the problem together, and then you'll return to your desks and work on the problem with paper and pencil."

Hassan shared first. "I tried ten dollars, but it didn't work because he still had ten dollars at the end."

"How did you figure?" I asked.

Hassan explained, "In the first store, the guy gave him ten dollars, so he had twenty. So when he spent ten, he still had ten. And the same thing happened in the two other stores."

Gissele said, "I tried twenty dollars, and that was even worse."

"What do you mean by 'worse'?" I asked.

Gissele giggled. "He wound up with ninety dollars. In the first store, the storekeeper gave him twenty, and that was forty. He had thirty dollars left after spending ten. At the second store, the man gives him thirty dollars, so he has sixty dollars, and that gives him fifty left after spending ten. And in the third store, fifty plus fifty is one hundred, so he spends ten and has ninety dollars left."

I then directed the students' attention to the list of problem-solving strategies posted in the room.

Problem-Solving Strategies

Look for a pattern.

Construct a table.

Make an organized list.

Act it out.

Draw a picture.

Use objects.

Guess and check.

Work backward.

Write an equation.

Solve a simpler (or similar) problem.

Make a model.

"Hassan tried ten dollars and Gissele tried twenty dollars," I said. "Which of these strategies do you think they were using?"

"Guess and check," Kaisha said.

"Sort of act it out, too," Cara added.

"How many of you picked an amount and then checked to see if it would work?" I asked.

About a third of the students raised their hands.

"I tried nine dollars, but he had two dollars left at the end," Michael said.

"I tried eight dollars, but he was six dollars in the hole at the end," Elissa said.

"He was in the hole if you started with six dollars," George said. "I don't know how much exactly, but it's a lot."

"With seven dollars, too," Travon added.

Alexandra had a different idea. "We think that it's not a whole-dollar amount," she said. "We tried some of those numbers, too, and they didn't work. So it has to be some dollars and some cents."

"So now what are you going to do?" I asked.

"We'll try some other amounts near the ones that were close," she said.

"What do you mean by 'close'?" I probed.

"Well, he was in the hole with eight dollars but not with nine dollars, so maybe it's in between," Alexandra said thoughtfully.

"I did the work-backward method," Scott said, returning to the list of strategies. He explained to the class what he had told me before, that the man had to have five dollars going into the third store in order to have nothing left. Scott added, "So then I tried to figure out how much he had going into the second store to come out with five dollars."

Alvin raised a hand, excited. "I figured that out!" he said. "You have to add ten to the five and then divide by two. Five plus ten is fifteen, and half of that is seven dollars and fifty cents."

Several students protested. "I don't get it."

"Why did you divide it in half?"

"That's confusing."

"Is he right?" Keely asked me.

"Would you explain your idea some more?" I asked Alvin.

"OK," he said. "If he had seven fifty, and the store man gives him seven fifty, then he has fifteen dollars, right?" The others nodded. "Then he spends ten and has five left," Alvin continued. "So if you go the other way, add the ten back onto the five, and then take half, you know what he had when he went into the store."

Some of the students now nodded in agreement; others were still confused or unsure. I decided that it was time for them to continue working on their own. I said, "I think this is a good time for you all to get back to work. It's fine if you want to work with a partner, but each of you should do your own paper. Listen to what you should write." I waited a moment until all eyes were looking at me.

"Keep track of all of the figuring you do," I said. "Even if something turns out not to be right, leave it there. The information can help you later, and it can also help me understand how you were thinking. When you figure out the answer, explain in words how you finally got it. And finally, write about whether you think this problem was too easy, just right, or too hard for fifth graders."

"What's the problem called?" De'anna wanted to know. The students were used to writing titles on their papers.

"You can decide what to name it," I said.

"Can three of us work together?" Travon asked.

"That's fine," I said, "as long as you each do your own paper."

No one else had a question and the students went back to their desks to work. The room was productively noisy, and the students stayed engrossed.

After he solved the problem, James came to me with his usual question: "Do I have to write?"

Writing was always a struggle for James.

I looked at James's paper and saw that he had solved the problem numerically. I asked him to explain what he had written, and he did so clearly. "But Alvin helped me," he said.

"That's OK," I replied. "It's fine to use other people's ideas as long as they make sense to you."

"I kind of did it my own way, too," James said. "I guessed and checked, but Alvin worked backward and that was easier."

"That's how you can start your writing," I suggested. "You can explain that you first guessed and checked, and then you used Alvin's idea." James returned to his seat to complete his paper.

As the students worked, I started a T-table on the board, labeling the first column Start and the second column End. I recorded on the table the amounts that the students found as they worked, recording the end amounts as negative numbers when the man was in the hole and positive numbers when the man was ahead. After several entries, the table looked like this:

Start	End
$10.00	+ $10.00
$20.00	+ $90.00
$ 9.00	+ $ 2.00
$ 7.00	– $14.00
$ 8.00	– $ 6.00
$ 7.75	– $ 8.00

Elissa decided to rewrite the table with the start numbers in order and in $.25 intervals from $5.00 up to $10.00. Several others did, too.

I called the class back to the rug for a concluding conversation, and the interchange was lively. The general consensus was that guessing and checking, working backward, acting it out, and looking for a pattern were the most used problem-solving strategies. Elissa noticed that when the start amount changed by $.25, the end amount changed by $2.00. Alexandra wondered if the correct answer would double if you doubled the $10.00 in the problem to $20.00.

Not all of the students had the time to write about whether the problem was too easy, just right, or too hard. But our class discussion revealed that most of the students thought that the problem was just right for fifth graders.

Scott said, "It makes you think and it's a good challenge."

"And it's not boring," Gabriel added.

"I think it was hard," Michael said. "For a long time everything I tried was wrong."

Some students commented on their papers. George wrote: I thought this problem was just right for fifth graders because it doesn't take too long and you can't finish it in 1 min. Travon wrote: This was hard because there is no very helpful pattern that I know of. Cara wrote: I think it's just right and hard because you think a lot but it takes a long time. Kaisha had a different point of view. She wrote: I think it was pretty easy.

Figure 1. James's work.

Figure 2. Lauren's work.

Figure 3. Anthony's work.

Figure 4. Emma's work.

From Online Newsletter Issue Number 18, Summer 2005

View Lesson

Grades 4–5

Spaghetti and Meatballs for All!

Cheryl began the lesson by reading Spaghetti and Meatballs for All! aloud to the class. In the story, Mr. and Mrs. Comfort invite 32 family members and friends for a reunion and set eight square tables to seat four people at each, one to a side. As guests arrive, they all have their own ideas about how to rearrange the tables so that different-size groups can sit together. Mrs. Comfort protests, knowing that there will be seating problems later, but her protests are ignored. The party becomes a cheerful confusion of rearranged tables, chairs, plates, glasses, and food. It all works out in the end, however, when Mrs. Comfort was proved right after all.

When Cheryl ﬁnished reading the story, she asked the class, "What was Mrs. Comfort worried about?"

Nicole answered ﬁrst, "There wasn't going to be enough room, because when you push tables together you lose chairs," she said.

"What do you mean?" Cheryl asked.

"It's like, if you put two tables together, you lose seats where the tables touch. It's hard to explain." Nicole drew two tables in the air, pointing to the sides where they met. Cheryl sketched two squares on the board, drawing an arrow where the sides touched. "You mean lose chairs here?" she asked. Nicole nodded. (See ﬁgure 1).

After hearing other students' ideas about Mrs. Comfort's problem, Cheryl said, "Let's use Color Tiles to explore different ways to arrange just four tables. We'll start with just four tables."

Cheryl gave the class guidelines for arranging the square "tables." "When tiles touch," she said, "they must do so along an entire side. Parts of sides or only corners touching is not okay." She demonstrated on the overhead projector. (See ﬁgure 2.)

Cheryl also arranged the tiles in a way that didn't follow her rule and had students explain why. (See ﬁgure 3.)

Then she completed the instructions. "In your group, share the tiles I've placed on your table and ﬁnd different ways to arrange four tiles. Be sure to follow my rule." Cheryl had put out about 70 tiles for each group of four students.

As the students worked, Cheryl circulated around the classroom, observing the students and answering questions as needed. When everyone had had a chance to work on the problem, she interrupted the students and asked for their attention.

"What arrangements did you make?" Cheryl asked. "Who would like to describe an arrangement so I can build it with tiles on the overhead?"

"You can make a straight line," Brandon reported.

"Like this?" Cheryl asked, arranging four tiles into a 1-by-4 rectangle. Brandon nodded.

"Make a square with all four of them," Rachel said. Cheryl built a square using four tiles.

"I did a three and a one," Nicole said.

"What do you mean?" Cheryl asked.

"One little table like one of Nathan's," Nicole explained, "and then a 1-by-3."

"You can make four separate tables," Nathan said.

"You could make a T," Zak said. "Put three in a line and one underneath the middle one."

"I made that too, but mine's upside down," said Eric.

Cheryl built Eric's arrangement under Zak's and pointed out to the class that when you can ﬂip, rotate, or slide a shape to ﬁt exactly on another shape, the shapes are congruent. "We'll consider congruent shapes to be the same," she explained.

When the students' arrangements ﬁlled the overhead, Cheryl asked, "What if the only arrangements we used were single rectangular tables made from four tiles? What shapes should we remove?"

"The four separate tables I suggested," Nathan said.

Rifka added, "And the one that looks like the letter T."

"You also have to take off mine," Nicole said. "It's not a single rectangle."

When Malkia suggested removing the square, conversation erupted. Some students remembered that a square was a rectangle, but others didn't. Cheryl clariﬁed, "A square is a special kind of rectangle because its sides are all the same length. But, like a rectangle, a square still has four 90-degree angles and opposite sides parallel."

Cheryl wanted to be sure the students had a way to label the rectangles they built. She sketched a 1-by-4 rectangle on the board. "I can record this in two ways," she said, and recorded under the rectangle:

Cheryl then drew a 2-by-2 square and labeled it.

Cheryl pointed to the 2-by-2 square table and asked, "If one person sits on a side of a small square table, and no one sits at the corners or on the cracks between tables, how many people can sit here?"

"Easy, eight," replied Nicole. "Just count two people on each side times four sides."

"When you count the number of people who can sit at the table, you're actually ﬁnding its perimeter," Cheryl explained. "That's because each person sits at one side of the smaller squares and takes up one unit of length. So the perimeter of the 2-by-2 rectangle is 8 units."

"The perimeter of the 1-by-4 table is 10," Eric noticed.

Cheryl had the others check Eric's statement and also ﬁgure the perimeter of several other rectangles. She then introduced another problem.

"Let's think back to Mr. and Mrs. Comfort's party," Cheryl began. "Suppose that Mrs. Comfort had decided that all 32 people should sit at one large, solid rectangular table and she wanted to ﬁgure out how many small square tables to rent. See if you can ﬁnd all the possible rectangular tables of different sizes and shapes that can seat 32 people."

"Does each table have to ﬁt exactly 32?" JT wanted to know.

"Yes," Cheryl replied.

"How many tiles do we use?" Malkia asked.

"It will depend on the tables you build," Cheryl responded.

"Can we work with a partner?" Nicole asked.

"Yes," Cheryl replied, "but keep your own record."

There were no more questions. Cheryl gave one last direction. "Use the tiles, but draw your solutions on a piece of paper. Be sure to record the dimensions of each table and the number of people it will seat."

Observing the Children

For the rest of the class period, Cheryl observed the students at work and gave assistance when needed.

She watched Kathleen make a 16-by-2 rectangle. "Hmm," Kathleen said aloud as she worked, "let's see, 32 people. This should work, because 16 times 2 is 32." Kathleen frowned with concentration as she counted the sides of the squares. Then she looked up at Cheryl in surprise.

"I don't get it," she said. "I counted 36 seats. But that doesn't make sense, because 16 times 2 is 32. Maybe I counted wrong." She counted the sides again.

"Still 36. Huh." Kathleen shrugged, mixed the 16 tiles back into the pile in the middle of her table, and began building another rectangle.

"What are you doing?" Cheryl asked her.

"Well, I must have messed up 'cause the ﬁrst one I made didn't work, so I'm going to try something else," Kathleen replied.

"What are you going to try?" Cheryl asked.

"I don't know. I'm just going to mess around and see what happens," she said.

Cheryl watched as Kathleen began placing tiles in a long row one square wide. She kept counting the sides one by one each time she added a new tile. Finally, she smiled.

"It works! This one seats 32 people. It's a 1-by-15. Now to record it." Kathleen began sketching the rectangle on her paper.

Alex was sitting across from Kathleen. "I found that one too," he said. "Now I'm trying a 2-by something."

"Oh," Kathleen responded and then began to build a rectangle that was four squares wide.

Nathan came up to Cheryl. "I'm not drawing rectangles on my paper like everyone else," he said. "I decided to use Xs instead. But Luke told me it was wrong. Can't I draw Xs if I want to?" Nathan showed Cheryl his paper.

Cheryl asked Nathan to explain what he had done. Satisﬁed that he understood what he was doing, Cheryl said, "What you've done makes sense to me."

Nathan ran back to Luke. "I told you she'd say it was okay," he said.

Cheryl continued around the classroom. By the end of the period, she saw that all of the students had found some of the rectangles and some had found them all. She asked the children to put away the tiles and she collected their papers. Cheryl planned to continue the lesson the next day.

The Next Day

The next morning, Cheryl gave the class an extension to consider. "What's the least expensive way to seat 32 people at one large rectangular table? And what's the most expensive way? To answer, some of you will need to ﬁnd more table arrangements."

After about 10 minutes, Cheryl interrupted the students to begin a class discussion. "What options would the Comforts have to seat all 32 people at one table?" Cheryl asked. Students' hands shot up.

"They'd have a bunch, eight to be exact," Rachel said. Most students nodded or murmured their agreement.

"Can someone describe the dimensions of the tables that would work?" Cheryl asked. "I'll record them on the board."

Eric reported, "One-times-15, 2-times-14, 3-times-13, 4-times-12, 5-times-11, 6-times-10, 7times-9 and 8-times-8." After Cheryl recorded the dimensions, she went back and sketched each corresponding rectangle.

"Oh, I see a pattern!" Anfernee said. "Can I show it?" Cheryl nodded, and Anfernee came to the board. She said, pointing, "From the top to the bottom it goes 1, then 2, then 3, then 4, then 5, and so on, up to 8."

"And the other side goes down," Ann Maria added.

"Oh, yeah, I didn't see that," said Anfernee. "Yeah, 15, 14, 13, and on and on." He sat back down.

"Shouldn't the list keep going?" Cheryl asked. "Shouldn't a 9-by-7 rectangle come next?" (See ﬁgure 6.)

"You've got that one already," Malkia said.

"Yeah, 9-by-7 and 7-by-9 are the same," Nicole added.

"All the ones after 8-times-8 are repeats," Kirsten said, "so you can't count them."

"Let's think about how many square tables Mr. and Mrs. Comfort would have to rent for each large rectangle," Cheryl said. "How many would they have to rent for a 15-by-1 table?"

"Fifteen. Easy," several students responded.

"What about 2-by-14?" Cheryl continued. "How many tables would the Comforts have to rent for that arrangement?"

"Twenty-eight," many children called.

"What about the 3-by-13 arrangement?" Cheryl asked. The class quickly caught on to what Cheryl was doing.

"You're just multiplying," Rifka said. "Just do that for all of them – 28, 39, 48, 55, 60, 63, and 64."

"What do you notice about the shapes of the tables?" Cheryl then asked.

Malkia said, "The 8-by-8 is a square, and all the rest are rectangles."

"But the 8-by-8 is a rectangle too, remember?" Erin reminded Malkia.

"Look," Brandon said. "If they arrange a long, skinny rectangle for 32, then they can do it with only 15 tables. It's cheapest that way."

"And they'd save space, too, since the 1-times-15 takes up the least amount of space," added Sharnet.

"You'd need a long room, though," Nicole added, "like for a king's banquet."

Cheryl then stopped the conversation and gave a writing assignment to assess each student's thinking. She wrote three questions on the board for the children to answer:

What patterns were useful as you worked?
What table arrangements are the most and least economical?
What do you notice about the areas and perimeters of the arrangements you made?

The students worked on the assignment for the rest of the class.

View Lesson

Grades 4–5

In the Next Three Seconds . . . Predictions for the Millennium

Rowland Morgan's In the Next Three Seconds . . . Predictions for the Millennium (New York: Puffin, 1997) is a collection of predictions about everyday and not-so-everyday events that will take place in the next three seconds, the next three minutes, the next three hours, days, weeks . . . all the way up to the next three million years. Here, fifth graders explore just one of the predictions made in the book and use estimation, multiplication, and division to make a prediction of their own. This lesson appears in the new book Math and Nonfiction, Grades 3–5, by Stephanie Sheffield and Kathleen Gallagher.

I held the book so the students could see the cover as they came and sat down in the meeting area. The cover shows a large circle, which on careful inspection you can see is the outline of a pocket watch. Inside the circle are illustrations and words radiating from a central picture of the Statue of Liberty. The students squinted to read the small print surrounding the Statue of Liberty.

"Read us the one about the Statue of Liberty," Roxanne asked.

I read, "In the next three seconds, Italians will drink a stack of cases of mineral water as high as the Statue of Liberty."

"That's a lot," she sighed.

I read a few more entries from the book's cover to give the class a taste of what the book is like. When I opened the book, I purposely skipped the introduction, which gives directions for making your own predictions. Each two-page spread after the introduction covers a specific period of time, but always in increments of three: three seconds, three minutes, three hours, three days, three nights, three weeks, three months, three years, three decades, three centuries, three thousand years, and three million years. On the first two-page spread, I read almost all the entries for what could happen in the next three seconds. We learned that in the next three seconds, Russians will mail more than four thousand letters or parcels and that Americans will buy fifty-six air-conditioning units. The students were interested to learn that "ninety-three trees will be cut down to make the liners for disposable diapers."

"Every three seconds they cut down that many trees?" Olivia asked.

"They don't cut down trees at night," Rick countered.

Cameron disagreed, saying, "It isn't night on the other side of the world and they could be cutting the trees down there." I thought this might be a good time to talk about how the author had made his predictions.

"Do you think people really cut down exactly ninety-three trees every three seconds?" I asked.

"No," Burton said, "who could count all the trees being cut down all over the world at exactly the same three seconds? The author must be guessing."

I turned back and read the introduction, which explains the role of counting throughout history, from caterers counting for imperial banquets in ancient China to the sophisticated counting we do today with computers. "The facts in this book are worked out from this great new wealth of information," I explained.

"Do you think the author's predictions are meant to be exact or estimates?" I asked. The children agreed that the predictions must be estimates, and I moved on to read the next page, which is about what will happen in the next three minutes. There is more to this book than could possibly be read in one sitting, so I read just enough to give the students the flavor of each section.

I then presented the problem I wanted the students to solve. "If you wanted to make a prediction about things that take three minutes or three hours, what is something you could easily count without leaving this room?" I asked. I suggested that they talk to someone sitting near them about their ideas. "Together, see if you can come up with something you could count in the room that would help you make a prediction about that same event in the future."

The students talked together and raised their hands excitedly to report on their ideas.

Cameron said, "We think we could count how many times someone blinks their eyes in a minute and figure it out for three minutes or three hours."

"That sounds workable," I said. "Any other ideas?"

Ranna suggested, "We could count how many times you breathe. That would be easy!" We talked together and decided to choose these two options, breathing and blinking. Then we discussed how to gather the information we needed to make the predictions.

"We have to count how many times our partner breathes or blinks in three minutes," Anthony explained. "Then we can figure out how many times they could breathe or blink in three hours."

"I think it will be boring counting someone blinking for three minutes," Andres mused. "Can't we just count for one minute and multiply by three?"

"Absolutely," I said. "You and your partner need to decide on the prediction you'd like to make, and then take turns counting each other's breathing or blinking. Then think about the calculations you'll have to do to make your predictions." The students returned to their seats with their partners.

Ethan and Cecelia made a plan for timing. "I'll time one minute and you count me blink," Cecelia told Ethan. "Then we'll multiply that by three." Cecelia motioned to Ethan to start counting. He made a tally mark every time she blinked until she motioned again for him to stop. Cecelia counted up her blinks and found that she had blinked twenty times. Next they traded jobs and Cecelia made tally marks for Ethan's blinks—twenty-two times in one minute.To find their average number of blinks per minute they added twenty plus twenty-two and divided by two. Next they multiplied twenty-one by three to find out how many times, on average, they blinked in three minutes.

Cameron and Mason's average blinks in a minute were only five. They multiplied by three to get fifteen blinks in three minutes, then multiplied sixty by three. Confused, I asked, "Where did the sixty come from?"

Cameron answered, "There's sixty minutes in an hour, so sixty minutes times three hours is one hundred eighty minutes. Then we multiplied that times five, because we blink five times every minute. That gives us nine hundred blinks in three hours." (See Figure 1.)

Students calculate their blink rate in three minutes, three hours, and so forth. | Math Solutions, Classroom Lessons

Figure 1. Cameron and Mason clearly understood how to calculate the number of times they could blink in three minutes, three hours, and so forth.

Ingrid, Douglas, and Ranna took turns timing one minute and counting each other blinking.They used twelve blinks per minute as their average. Ranna explained what they did: "First we multiplied twelve by three and found out we blinked thirty-six times in three minutes. Then we knew that there are sixty minutes in an hour, so we timesed sixty by three and got one hundred eighty."

"That's how many minutes in three hours," Douglas cut in.

"Right," Ranna continued. "So we multiplied one hundred eighty times thirty-six and got six thousand five hundred forty." I noticed that they had made an error when adding partial products, incorrectly adding eighty plus sixty, because one of the zeroes in the tens place looked like a six. Because of this error, their other calculations were off. (See Figure 2.)

Three students attempted to compute their blink rate for 3 minutes, 3 hours, so forth, but made an error early on. | Math Solutions, Classroom Lessons

Figure 2. Ingrid, Douglas, and Ranna made a computation error in one of the early steps and had to recalculate.

To follow up on this lesson, students could research other pieces of information, such as these:

the number of cartons of milk drunk in the next three days or weeks
the number of teeth lost by students in three days
the number of pencils used by students in three weeks

Related Publication:
Math and Nonfiction, Grades 3–5
by Stephanie Sheffield and Kathleen Gallagher

From Online Newsletter Issue Number 16, Winter 2004–2005

View Lesson

Grades 4–5

True, False, and Open Sentences

In this beginning lesson, students first explore arithmetic sentences to decide whether they are true or false. The lesson then introduces students to sentences that are neither true nor false but are algebraic equations, also called open sentences, such as x + 3 = 7 or 2 x= 12. The activity appears in Maryann Wickett, Katharine Kharas, and Marilyn Burns's new book, Lessons for Algebraic Thinking, Grades 3–5 (Math Solutions Publications, 2002).

I wrote on the board:

8 + 4 = 5 + 7

5 = 4 + 1

6 • 0 = 6

For each, I had a student read it aloud, tell if it was true or false, and explain why. Few students knew how to read the third sentence. I explained, "You can use a dot in this way instead of the times sign that you usually use for multiplication."

"I know about the third problem now," Tawny said. "You read it, 'six times zero equals six,' and that's false."

I then asked the students to write examples of arithmetic equations that were true and some that were false. A few minutes later I interrupted them. I drew two columns on the board, one for true mathematical sentences, and a second for false mathematical sentences. I said, "When I call on you, read one of your mathematical sentences. Don't tell if you think it is true or false. We'll guess and see if you agree with our guess." I called on Rayna.

She said, "You multiply six times three and divide that by two. Then comes the equals sign. On the other side you do four plus five."

I paused to give students time to think and then asked Rayna to come up to the board and write her equation. She wrote:

6 x 3 ÷ 2 = 4 + 5

After a few moments, most students were clear that it was correct. I wrote her equation in the True column.

After several other students shared their equations, even though more of the students wanted to do so, I moved on with the lesson. As the students watched, I wrote the following on the board:

5 +? = 13

"Is this equation true or false?" I asked. The class was quiet. Finally a few hands went up. I called on Jazmin.

"It could be either," Jazmin said. "We don't know what the box is, so we don't know if it's true or false."

"How could we make it true?" I asked.

"Write eight in the box because five and eight equals thirteen," Lizzie said. I did as Lizzie instructed.

5 +? = 13

"Is there any other number I could write in the box that will make the sentence true?" I asked. "I don't think so," Truc said. "I think the only way to make it true is to put eight in the box." "I think you could make it work by fractions," Chase said. "You could put sixteen over two as the answer, and that would make it true." As I wrote 16/2 on the board, several hands went up.

"Sixteen over two looks different, but it's really the same amount," Jessie said.

"It's still eight," Tina added.

I replied, "The equation would be true as long as whatever we put in the box is equivalent to eight." No one had any further comments.

"Mathematical sentences like this one are called open sentences," I said. "They're neither true nor false because there's a part of the sentence, the box in my equation, that isn't a number. The box is called a variable, because you can vary what number you put into it or use to replace it." I wrote open sentence and variable on the board. I planned to use this vocabulary regularly to help students become familiar and comfortable with it, just as periodically throughout the lesson I interchanged "equation" with "mathematical sentence."

"Would 'seven times six equals box' be an open sentence?" Jazmin asked. I wrote on the board:

7 • 6 =?

Most thought that it was. Lucy said, "Forty-two should go in the box if you want the problem to be true. If you put thirty-nine in the box instead, then it's false."

"What about 'four plus box equals twelve'?" Joshua asked. I wrote on the board:

4 +? = 12

"Joshua wants to know if this is an open sentence," I said. "Put your thumb up if you think this is an open sentence, down if you think it isn't, and sideways if you're not sure." All thumbs went up.

"I agree that it's an open sentence," I said. "Why is it?"

"I know," Turner said. "Because whether or not it's true or false depends on what goes in the box."

"What would make it true?" I asked. "Show me with your fingers." The students put up eight fingers each.

"What would make it false?" I asked.

Terry said, "Anything would make it false except for eight, so all other numbers make it false."

I then said to the class, "With your partner, for a few minutes write some other open sentences." After a few minutes, I asked for the class's attention. I called on Lizzie.

"How about 'fifteen thousand plus one equals box'?" Lizzie said. I wrote on the board:

15,000 + 1 =?

The others showed thumbs up to agree that it was an open sentence. "What number can we write in the box to make Lizzie's open sentence true?" I asked. I called on Diego.

"Fifteen thousand and one," he said.

"Who would like to come up to the board and write fifteen thousand one?" I asked. Few hands were raised. Some children weren't sure that they could write the number correctly. I called on Keith.

"It won't fit in the box," he said.

"I can make the box bigger," I responded. I did so and Keith came to the board and correctly wrote 15,001.

I then called on Kenny to give another open sentence. "'Triangle minus four equals three'," Kenny said.

I wrote on the board:

?– 4 = 3

Again, the students showed their agreement. "Who knows what number to put into the triangle to make the open sentence true?" I asked.

"Seven," Dana said. The others agreed.

It's important for students to learn that we can use different symbols for variables. I was pleased that Kenny had volunteered the use of a triangle. If no student had, however, I would have written an open sentence as Kenny did, talked about it with the students, and thenintroduced other symbols as well. Since Kenny made his suggestion, I built on it at this time. Underneath Kenny's equation, I wrote:

? – 4 = 3

I said, "I think that my open sentence is the same as Kenny's in one way and different in another way. Who can explain what I'm thinking?" Hands shot up.

"You used a box instead of a triangle," Tawny said.

"Yes, I used a box for the variable and Kenny used a triangle for the variable," I said, taking the opportunity to use the word variable.

"But the numbers are the same," Terry said. I then wrote on the board:

x – 4 = 3

"What about this equation?" I asked. "Is it an open sentence?" Some thought it was and others weren't sure.

"Who would like to use your own words to explain what an open sentence is?" I called on Tony.

"It's a sentence that has a box or something that stands for a number," Tony explained. "It depends on what number you put in whether or not it's true."

"So what do you think about the sentence I wrote with the x instead of a box or triangle?" Most of the students thought it was an open sentence; three weren't sure.

"Who would like to explain why you think it's an open sentence?" I said. I called on Terry.

He said, "It has something that stands for a number that's missing. I think you can use whatever you want. An x is OK, so is a box, so is a triangle."

"I agree with Terry," I said.

"Can you use any letter?" Lucy asked.

"Yes," I replied. "Actually, you could use any symbol you'd like. But mostly we see boxes, triangles, and letters used for variables in equations." I continued until the end of the period,having students give their open sentences and asking the others to think about what numberto use for the variable to make the open sentence true. By the end of class, the students all seemed comfortable with categorizing true, false, and open sentences and figuring out how to make open sentences true.

From Online Newsletter Issue Number 7, Fall 2002

Related Publication:
Lessons for Algebraic Thinking, Grades 3–5
by Maryann Wickett, Katherine Kharas, and Marilyn Burns

View Lesson

Grades 4–5

Ratio

The Golden Ratio is a ratio of length to width and is approximately 1:1.618. This ratio not only appears in art and architecture, but also can be observed in nature and in the human body. The Golden Ratio is the ratio of a person's total height to height from their feet to their navel.

How does your total height compare to the height from your feet to your navel?

Is it close to the Golden Ratio?

Investigate other lengths, such as the distance from the waist to the ﬂoor and from the top of the head to the waist, to see whether a similar ratio exists between those measurements. Enter your data on a chart. How does your data compare to other members of your class? Things to consider How will you compare your data with other students in your class?

See the PDF for a more complete version of this lesson.

From Investigations, Tasks, and Rubrics to Teach and Assess Math by Pat Lilburn and Alex Ciurak.

Related Publication:
Investigations, Tasks, and Rubrics to Teach and Assess Math, Grades 1–6
by Pat Lilburn and Alex Ciurak

View Lesson

Grades 4–5

Learning from Student Writing

In the previous issue of the Math Solutions newsletter (Number 23, Spring/Summer 1998), I described the Put in Order lesson that I had taught to help fifth graders learn to compare and order fractions. Throughout the year, I continued helping the class learn ways to compare fractions. As always, I learned a great deal from the students, especially from their written work. Most revealing to me was the variety of strategies that students developed for comparing fractions. Below, I describe some of what I learned from their writing and offer suggestions for how you can use writing with your students.

In my early years teaching mathematics, I taught students to compare fractions the way I had learned as an elementary student—convert the fractions so they all have common denominators. However, in my more recent teaching of fractions, I do not teach one method. Instead, I prod students to think, reason, and make sense of comparing fractions, helping them learn a variety of strategies that they can apply appropriately in different situations. While changing fractions so that they have common denominators is one useful strategy, it's not the only nor most efficient one.

To help students learn to compare fractions, I used several types of lessons. I gave students real-world problems to solve, such as sharing cookies or comparing how much pizza different people ate, and had class discussions about different ways to solve the problems. I gave them experiences with manipulative materials—pattern blocks, color tiles, Cuisenaire rods, and others—and we explored and discussed how to represent fractional parts. I taught fraction games that required them to compare fractions, and we shared strategies. At times I just gave them fractions, and we discussed different ways to compare them.

We talked a good deal about fractions. By expressing their own ideas and hearing ideas from others, children expand their views of how to think mathematically. Also, talking and listening helps prepare them for writing, which I have them do individually several times a week in class and often for homework as well. My students had many opportunities to explain in writing how they compared fractions.

I began one class lesson by asking the students to think about two fractions—6/8 and 4/5."Which is larger?" I asked them. "And how did you decide?" On this day I didn't have them discuss their ideas but instead asked them to write individually so that I could see how each student thought. (How would you decide which of the two fractions is larger?)

The students' papers showed a variety of methods. Laura wrote: 6/8 < 4/5 because 6/8 = 12/16 and 4/5 = 12/15 and you have the same numerator so it makes it easier in a way. 12/15 is bigger than 12/16 so 4/5 is bigger than 6/8. I wasn't sure from my first reading how Laura was thinking. This is common when I read student work. It's hard to follow others' reasoning, especially when their thinking differs from ours. I read Laura's explanation again and realized that she had converted the fractions so that they had common numerators. She didn't explain how she knew that 12/15 was larger than 12/16; that seemed to be obvious to her. But her method worked and was efficient.

A student explains with visuals how she found six-eights to be less than four-fifths. | Math Solutions, Classroom Lessons

Laura converted the fractions so that they had common numerators.

I read Brian's paper next. He had reasoned the same way that Laura had but expressed his thinking differently and with more detail. He wrote: I know that 6/8 is = to 12/16 because 6 x 2 = 12 and 8 x 2 = 16. 4/5 = to 12/15 because 4 x 3 = 12 and 5 x 3 = 15. 16 is a bigger number but a smaller fraction and 15 is a smaller number but a bigger fraction witch makes 6/8 = 12/16 , 4/5 = 12/15. While Brian's language wasn't precise, it indicated that he understood that the larger the denominator, the smaller the fractional pieces. Brian's paper was easier for me to understand, mostly I think because I had reasoned through Laura's paper first.

Jenny, however, reasoned differently. She compared both fractions to one whole. She wrote: 6/8 is less than 4/5 because 6/8 is 2/8 away from 1 whole and 4/5 is 1/5 away from the whole. 2/8 is equal to 1/4. 1/4 is bigger than 1/5 so 4/5 is bigger than 6/8 because it has a smaller amount left to get to the whole. Jenny's method also shows her understanding of equivalent fractions, that 2/8 is the same as 1/4. Jenny's explanation was clear and correct. Also, it was easy for me to understand because her approach mirrored the way I had thought about the problem.

A student explains her sense-making and how she found common numerators.| Math Solutions, Classroom Lessons

Jenny converted the fractions so that they had common numerators.

As Jenny did, Donald compared both fractions to one whole and showed his understanding of equivalent fractions. But he also thought about common numerators. He wrote: I know that 6/8 is less than 4/5 because 4/5 = 8/10 which is 2/10 away from the whole. And 6/8 is 2/8 away from the whole. And 8/10 is more than 6/8 because 10's are smaller than 8's which would make it closer a whole which make it more. Donald's explanation had some grammatical errors. He meant "10ths" and "8ths," not 10's and 8's. His use of "it" twice weakened the last sentence, and he left out the word "to" where it needed to be. The sentence would have been clearer if Donald had written: And 8/10 is more than 6/8 because 10ths are smaller than 8ths, which would make 8/10 closer to a whole, which makes it more. Donald needed to be reminded regularly to reread his papers before handing them in, and although this paper represented an improvement in his writing, more improvement was still needed.

Mariah relied on common denominators. She wrote: I know that 6/8 < 4/5 because I know that 6/8 also = 30/40 and 4/5 also = 32/40 and 30/40 is less than 32/40 so 4/5 > 6/8. 30/40 is 2/40 away from 32/40. I got this because I used common denominators. Mariah also showed how she had arrived at the converted fractions. She wrote:

4/5 8/10 12/15 16/20 20/25 24/30 28/35 32/40

6/8 12/16 18/24 24/32 30/40

All of these students' answers were correct and their explanations acceptable. They were doing what made sense to them as they tried to reason about the fractions.

Using Student Writing in the Classroom

After reading through the students' papers, I have discussions with individuals, especially if I'm having difficulty understanding their reasoning or if their reasoning was incorrect or incomplete. Sometimes I focus on their writing errors; other times I keep the focus just on the mathematics. Making this decision depends on the paper, the student, and the mathematics involved.

Then I have some students share their papers with the class so that students can benefit from one another's thinking. For example, I asked Mariah to show the class how she arrived at fractions with common denominators. Her method wasn't conventional, but it was mathematically correct and effective. She explained, "I kept changing them to equal fractions. I knew when I hit fortieths that it would work for six-eighths."

Mariah's explanation sparked a class discussion about other ways to convert fractions to ones with common denominators. Several students were eager to explain the methods they used. Raul was particularly animated. He said, "If you multiply 8 times 5 you get 40 and you can use that as the denominator. Then multiply 6 times 5 to get 30 and have that numerator for six-eighths and then multiply 4 times 8 and use 32 for the numerator for four-fifths."

Mariah nodded, seeming to understand. "But I like my way better," she said softly.

"It's okay for you to use your own method," I responded. "It's also important that you learn different ways to think about fractions." (I constantly remind children to look at many different strategies to develop and extend their mathematical repertoire.) Then I wrote two fractions on the board—3/4 and 2/3—and asked each student to take out a sheet of paper and convert these to common denominators in two ways – Mariah's way and Raul's way. I had them compare their work with partners and then had volunteers demonstrate each method so that Mariah and Raul could judge if their methods had been correctly applied.

I had others read their papers as well and began to compile a class list of strategies for comparing fractions. I acknowledged students' ideas—Mariah's way, Brian's way, and so on—and then gave the strategies general names—convert to common denominators, see which is closer to 1, convert to common numerators, etc. I wrote the list on chart paper and kept it posted in the classroom for students to refer to.

In my years of teaching, not all classes have come up with all strategies. (This was the first class in which common numerators was such a prevalent strategy.) If there's a strategy that I want students to know about and no one presents it, then I present and provide practice with it. But I present it as one of many, not as the "best" or "right" way to compare fractions. The "best" or "right" way depends on the context, the numbers, the purpose, or some combination of these factors.

A Suggestion for Your Classroom

You might try sharing one of my students' methods with your class. Reproduce one of them, give copies to students in pairs, and have them see if they can figure out why it makes sense. Have them explain the method in their own words. Then give them practice applying it to other fractions. In this way, you can use the work of some of my students to help develop your own students' skills and understanding. But, if possible, share the ideas from your own class. Honoring your students' thinking is a way to involve them as important contributors to their learning and to the learning of their classmates.

From Printed Newsletter Issue Number 24, Fall/Winter 1998–1999

View Lesson

Grades 4–5

Using Protractors in Middle School

From her past experiences teaching middle school students about angles, Cathy Humphreys knew that students often have difﬁculty learning how to use protractors. Often they don't see the need for the tool, so Cathy does not introduce protractors until after the students have had concrete experiences measuring angles several ways.

When Cathy distributed protractors to her class in San Jose, California, she told the students, "The protractor is a useful tool for both measuring angles and drawing angles of speciﬁc sizes." She asked the students to work in pairs and explore the protractors.

"It may be helpful to use a right angle as a reference," she suggested, "since you already know a right angle is ninety degrees."

After a while, Cathy called the class to attention and asked the students to share what they had noticed. Then Cathy gave them the challenge of ﬁguring out how to use the protractors and writing directions that someone else could follow. She said, "Your directions should tell how to measure angles and also how to draw angles of different sizes. You can include drawings if they will help make your directions clear."

Before the students began, Cathy wrote protractor and angle on the overhead for their reference and asked them what other words about angles they might use. She listed all the words the students suggested: acute, right, obtuse, straight, degrees.

Students expressed their thinking in different ways. Jenny and Sara, for example, wrote the following directions for measuring an angle: First, you make an angle. Then you place the bottom line of the angle on the line of the protractor. Then you put the dot on the vertex of the angle. Then you ﬁnd out what degree the angle is. If you can't ﬁgure it out, you do this. First you ﬁnd out if your angle is obtuse or acute. If it's obtuse you use the top line, if it's acute you use the bottom line. Then you get the arrow and take it up to the number and the number that the line hits is the degree of your angle.

Cuong and Cheryl wrote: One rule you must always remember is you must always have the bulls eye on the straight line on the bottom. If the angle goes to the right you must read the bottom numbers. But if it goes to the left you must read the top numbers.

Ron M. and Ron S. wrote: You place the vertex of the angle you are measuring in the middle of the hole. The hole is on the bottom of the protractor. When measuring, let's say that it is 69 degrees. On the protractor it does not say 69°. Only 50, 60, & 70. What you do is measure and count by the lines on top of the protractor.

Will and Pat designed their work as a pamphlet. They titled it The Protractor Manual (see Figure 1). They wrote: There are 2 things a Protractor does for you. it 1. measures angles and 2. It makes new angles. To measure an angle you put the rough side of the protractor down then put the vertex of the angle in the little hole in the middle, (shown on pages 3 and 4) and if the angle is acute you use the numbers on the bottom on the right but if it is acute but it is pointing to the left you use the left side and the top (shown on pages 3 and 4). To make angles you use the bottom part of the Protractor (shown on pages 3 and 4) to make any angle you disire.

Figure 1.

In Figures 2 and 3, students explained in different ways how to use a protractor.

Figure 2.

Figure 3.

From Online Newsletter Issue Number 4, Winter 2001–2002

Related Publication:
Writing in Math Class: A Resource for Grades 2–8
by Marilyn Burns

View Lesson

Grades 4–5

Division Partner Game

In this partner game, fourth grade students divide by 1-digit numbers to determine if the quotient has a remainder.

The key to learning mathematics is understanding the "why" behind the "how". HMHInto Math emphasizes the importance of establishing conceptual understanding and reinforces that understanding with procedural practice. The learning model asks students to first develop their reasoning before connecting their understanding to concepts and skills.

HMH Into Math is more than just a solution, it's a vision for student growth.

HMH Into Math was built to ensure growth for each and every student.

With confident educators and empowered students, the journey toward a true depth of understanding and a culture of growth in every mathematics classroom becomes an achievable reality.

HMH Into Math incorporates learning activities that encourage productive perseverance to transform mathematics fear into mathematics enthusiasm.

View Lesson

Grades 4–5

The Lobster Problem

Money is a useful model for helping students make sense of tenths and hundredths, but students often have difficulty extending their knowledge to make sense of thousandths and ten thousandths. The Lobster Problem presents students with a problem-solving experience that helps them learn about extending decimals beyond hundredths and provides them practice with identifying decimals that come in between other numbers. The lesson appeared in Carrie De Francisco and Marilyn Burns's Teaching Arithmetic: Lessons for Decimals and Percents, Grades 5–6 (Math Solutions Publications, 2002).

I asked the students, "According to a grocery's digital scale, a lobster weighs more than two and fifty-six–hundredths pounds but less than two and fifty-seven–hundredths pounds. How much do you think the lobster weighs?" On the board I wrote:

2.56 lb.

2.57 lb.

Marcus said, "There's nothing in between. Fifty-seven–hundredths comes right after fiftysix–hundredths." No one had a different idea.

"What if the scale said the lobster weighed between two pounds and three pounds? How much could the lobster weigh?"

Shannon answered, "The lobster could weigh two and a half pounds."

"Could it weigh anything else?" The room was quiet.

"What if the scale registered the lobster at a weight between two and one-half pounds and three pounds? How much could the lobster weigh then?" Still there was no response.

I changed the question again, this time using a context that was more familiar. "Suppose that Matthew had more than two dollars and fifty cents, but less than three dollars. How much money could Matthew have?"

Jonathan responded, "He could have two dollars and fifty-one cents or two dollars and seventy-five cents or anything in between."

"What do you mean by 'anything in between'?" I asked.

Jonathan continued, "Matthew could have any amount more than two fifty but not more than three dollars, like two sixty, two seventy, all the way up to three dollars."

"Does anyone have another way to answer my question?" I asked.

Elaine suggested, "Matthew could also have different combinations of money between two sixty and two seventy. He could have two sixty-one, two sixty-two, all the way up to two seventy. And he could have even more combinations between two eighty and two ninety."

I then asked, "Is two dollars and ninety cents the most Matthew could have before exceeding three dollars?"

"No, he can have two ninety-one, two ninety-two, up to two ninety-nine," Laura answered. "So the most he could have is two dollars and ninety-nine cents."

"How do we know that?" I asked.

Luis explained, "You start with two-ninety and you keep adding cents until you get to the next dollar."

"Can anyone explain it another way?" I asked.

"It's like you have two dollar bills and nine dimes and if you add one more dime, you'll have three dollars, but you can't go over three dollars," Maria responded. "So you need to add pennies or nickels to your ninety cents until you get close to three bucks."

"What is the least amount of money Matthew can have?" I asked.

Ian said, "Two fifty-one." Others agreed.

I continued, "So Matthew could have many different combinations of money between two fifty and three dollars just by adding pennies, or cents."

The students seemed clear about this, so I presented another version of the lobster question. "What if the digital scale registered the lobster at a weight between two and five-tenths pounds and three pounds? How much could the lobster weigh?" On the board I wrote:

2.5 lb.

3 lb.

I continued, "With your partner, think about our discussion about money. Decide if there could indeed be measurements between two and five-tenths pounds and three pounds and, if so, discuss the possible weights of the lobster." The students began to discuss in pairs. Many students felt that the lobster could weigh anywhere between 2.51 pounds and 2.99 pounds, much as Matthew could have had any amount of money between $2.51 and $2.99.

I then brought the students' attention back to the original question. "According to a grocery's digital scale, a lobster weighs more than two and fifty-six–hundredths pounds but less than two and fifty-seven–hundredths pounds. How much do you think the lobster weighs?" I pointed to the board where I had written 2.56 lb. and 2.57 lb. "Brainstorm with your partner possible weights in between these. Make sure you both agree on the numbers you come up with, know how to say them, and are able to explain your thinking."

Several pairs of students tried to make a connection between money and weight but quickly realized that there isn't anything smaller than a penny in our money system. Many students were able to make the assumption that they could add another decimal place behind the last number as they had done when the lobster weighed more than 2.5 pounds — 2.561 or 2.562 pounds, for example. However, they were having difficulty reading the possible solutions.

To help students read their decimals, I listed three decimals on the board:

2.5

2.56

2.562

"Let's read the first number on the board aloud together," I said. The students did so easily. I repeated this for the second number, and again the students were able to do this.

"What about the last number?" I asked. "Any ideas?"

Luis tried. "It's two and five hundred sixty-two something," he said.

"You're right. Now what about the 'something' part?" I asked.

"I remember," Jenny said. "When we had the big cube be one, then the little cube was one thousand." Jenny remembered our earlier exploration with base ten blocks.

"One-thousandth," I corrected, emphasizing the that the end. I wrote on the board:

one-thousandth

"So if you put together what Luis and Jenny said, the number is two and five hundred sixty two–thousandths." I also wrote the number on the board using words to help students who find it easier to learn by reading than by listening.

two and five hundred sixty-two–thousandths

We read the number aloud, and then I asked the students for solutions to the question about the in-between weights. As students called out answers, I wrote them on the board. Most agreed that the least the lobster could weigh was 2.561 pounds and the most it could weigh was 2.569 pounds.

Then Blaire asked, "Couldn't the lobster have a lot more weights than that?"

"Can you come up and show what you think?" I asked. Blaire agreed. She came up and wrote 2.561 on the far left side of the board and 2.569 on the far right. Then, under the number 2.561, she listed 2.561 nine times. At the end of the first 2.561 in her list, she added a 1 to make it 2.5611. At the end of the second 2.561, she added a 2. Blaire continued down the list, creating a sequence of numbers each with four decimal places:

2.5611

2.5612

2.5613

2.5614

2.5615

2.5616

2.5617

2.5618

2.5619

Blaire said, "I think this can go on and on." When Blaire wrote the numbers in a column and then added the digits from 1 through 9, it opened up for the others a new way of thinking about decimals.

"Does anyone have an idea about how to read the first number in Blaire's list?" I asked.

Madison said, "If you follow the pattern, then you do ten times thousandths, but I don't know what it is."

Misha said, "I bet it's ten thousandths."

"How would you read the number?" I asked.

She replied, "That's hard."

I said, "Listen as I read the first number." I pointed at each digit in 2.5611 as I said, "It's two and five thousand, six hundred eleven–ten thousandths. When you have four digits after the decimal point, the number refers to ten thousandths."

Isaac asked, "So what's the answer? What can the lobster weigh?"

"What do you think?" I replied.

"I don't think there's one correct answer," he said. "I think there are tons of answers."

To end the class period, I gave a homework assignment. I said, "Think about what we discussed today and decide if you agree with Isaac and Blaire. Answer this question." I wrote on the board:

Are there an infinite number of possible weights between 2.56 lbs. and 2.57 lbs.? Explain your thinking and give examples.

(See Figures 1–3 on the following pages.)

Justin agreed with Blaire and was clear about the infinite number of weights between 2.56 lbs. and 2.57 lbs. | Math Solutions, Classroom Lessons

Figure 1. Justin agreed with Blaire and was clear about the infinite number of weights between 2.56 lbs. and 2.57 lbs.

When asked to write, Luis showed that he didn't understand Blaire's explanation.| Math Solutions, Classroom Lessons

Figure 2. When asked to write, Luis showed that he didn't understand Blaire's explanation.

Matthew wrote that there are weights between 2.56 lbs. and 2.57 lbs., but his examples showed his lack of understanding. | Math Solutions, Classroom Lessons

Figure 3. Matthew wrote that there are weights between 2.56 lbs. and 2.57 lbs., but his examples showed his lack of understanding.

Related Publication:
Teaching Arithmetic: Lessons for Decimals and Percents, Grades 5–6
by Carrie DeFrancisco and Marilyn Burns

From Online Newsletter Issue Number 10, Summer 2003

View Lesson

Grades 4–5

Venn Diagrams and Percents

I drew a large Venn diagram on the chalkboard and labeled each of the three intersecting circles: Has taken musical instrument lessons, Doesn't like papaya, Street address has exactly three digits. The students' interest piqued when I wrote my initials on a 1-by-2 inch Post-it Note and placed it in the intersection of all three circles.

I explained, "Each circle describes something that's true about me, so I put my initials in the intersection. That's the only place on this Venn diagram that's inside each of the three sets." My explanation reviewed terminology about Venn diagrams.

"What three things do you now know about me?" I asked.

"You play an instrument, you don't like papaya, and your house number has three numbers in it," Rose said.

"Now I'm going to make some guesses about our class," I said. I wrote on the board:

45% play musical instruments
25% don't like papaya
33% live at addresses with exactly three digits

"Also," I continued, "I think only one other person in the class belongs in the center with me. Since there are twenty-ﬁve of us all together, what percent do I predict will be in the intersection?"

Jason said, loudly and conﬁdently, "Each person is worth 4 percent because there are twenty-ﬁve fours in one-hundred."

"Two people would be 8 percent," Lauren added. I wrote on the board:

8% will be in the intersection of the three sets

I then gave each student a Post-it Note to use for his or her initials. Then I asked Maryann to go up to the board and place hers on the Venn diagram.

"I'm kind of confused," Maryann said. "I take music lessons and my address has exactly three digits, but I don't know if I like papaya. I think I need another Post-it."

Aaron had a thought. "Since you don't know whether you like papaya or not, you just need to be in the circles that say you live in a house with three numbers and that you take music lessons." He came up and helped Maryann place her Post-it Note.

"Is there anyone who doesn't belong in any of the circles?" I asked. Jeffrey raised his hand.

"So, should Jeffrey throw out his Post-it Note?" I asked.

"He's still part of the class," Lauren commented.

"I think I go outside," said Jeffrey as he went up and placed his Post-it outside of the three circles. I nodded my agreement.

I then placed my ﬁnger in the section that was inside the circle labeled "Has an address of exactly three digits" but not in any other set.

"What's true about someone who would put his or her Post-it right here?" I asked. Louise correctly identiﬁed that the person had a street address with three digits.

"What else do you know about that person?" I asked.

Everyone thought for a moment. Then Louise piped up, "They don't like papaya and they don't play an instrument."

I then said, "Now you'll all place your Post-it Notes where they belong. If you're not sure, consult with a friend." I had the students come up to the chalkboard in small groups. Some children knew exactly where to place their Post-it Notes, and others were hesitant. But animated conversation helped each student decide.

After all of the students had posted their initials, we counted the Post-it Notes to verify that there were twenty-ﬁve of them. I allowed a minute for silent inspection of the results. We then tallied the information and I recorded it on the board:

15 have taken music lessons
9 have addresses with exactly three digits
5 dislike papaya

"Let's ﬁgure out what percent of our class actually ﬁts each category," I said. "Don't reach for your calculators — I'd like us to ﬁgure these out in our heads." Calculators are available to students at all times, so this wasn't a typical direction. However, I wanted the students to reason mentally and talk about their thinking. I felt the discussion would help students who weren't as conﬁdent. To help, I wrote on the board what Jason had already said:

1 person = 4 percent

I asked, "How many people would represent 50 percent?"

"Twelve and a half," said Lauren thoughtfully, "but you can't have a half a person, so I guess we can't have exactly 50 percent in any category."

"We can't have 10 percent either," Tom said. "Nope, that would be two and a half people."

"Let's see how well I did with my guesses," I said. "Fifteen out of twenty-ﬁve of us take music lessons. Is it more than 50 percent or less than 50 percent?" The benchmark of one-half is useful for calculating exact percentages.

"It's more than half, but not so far away," Paul said. "I think it's 60 percent."

"How do you know?" I asked.

Paul answered, "I know that ﬁfteen-twenty-ﬁfths can be reduced to three-ﬁfths, and I know that each ﬁfth is 20 percent. So I needed three 20 percents."

Isaac joined in. "I just multiplied ﬁfteen by four since I knew that each person is worth 4 percent," he said.

Tom added, "I know that 100 percent of the people would be twenty-ﬁve, so ten people, or 40 percent, are not in the circle. Sixty percent are."

"Well, how did I do?" I asked.

"Not so good," Alan said. "You guessed less than half of us and it was more than half."

Sarah countered, "But she was only 15 percent off."

"Fifteen percent is practically four people off," David said, "and in a class of twenty-ﬁ ve, that's not too bad."

We then ﬁgured out that 36 percent lived at three-digit addresses and 20 percent disliked
papaya.

Next I distributed newsprint and markers and asked the students to draw Venn diagrams with three intersecting circles, label each, and guess how many of the class would fall into each category. At the end of class, I collected the papers. The following day, I redistributed them and had every student sign in on everybody else's Venn diagram. Students then calculated the percentages of people who ﬁt each category. There was a great deal of collaboration, and the class discussion that followed was rich and productive.

From Online Newsletter Issue Number 3, Fall 2001

Related Publication:
Math Homework That Counts, Grades 4–6
by Annette Raphel

View Lesson

Grades 4–5

Adding and Subtracting Decimals

In this partner game, fifth grade students practice adding and subtracting decimals.

HMH Into Math is more than just a solution, it's a vision for student growth.

HMH Into Math was built to ensure growth for each and every student.

With confident educators and empowered students, the journey toward a true depth of understanding and a culture of growth in every mathematics classroom becomes an achievable reality.

HMH Into Math incorporates learning activities that encourage productive perseverance to transform mathematics fear into mathematics enthusiasm.

View Lesson

Grades 4–5

Introducing Multiplication of Fractions

Teaching multiplication of fractions is, in one way, simple—the rule of multiplying across the numerators and the denominators is easy for teachers to teach and for students to learn. However, teaching so that students also develop understanding is more demanding, and Marilyn Burns tackles this in her new book Teaching Arithmetic: Lessons for Multiplying and Dividing Fractions, Grades 5–6 (Math Solutions Publications, 2003). In the following excerpt from Chapter 2, Marilyn builds on what students know about multiplying whole numbers to begin developing understanding of what occurs when we multiply fractions.

I began the lesson by posting the chart of "true" statements about multiplying whole numbers that I had previously generated with the class:

Multiplication is the same as repeated addition when you add the same number again and again.
Times means "groups of."
A multiplication problem can be shown as a rectangle.
You can reverse the order of the factors and the product stays the same.
You can break numbers apart to make multiplying easier.
When you multiply two numbers, the product is larger than the factors unless one of the factors is zero or one.

I planned to use these statements as a base for helping the students think about multiplying fractions. To begin, I pointed to the first statement:

1. Multiplication is the same as repeated addition when you add the same number again and again.

"Do you think this is true when we think about fractions?" I asked. I wrote on the board:

6 x 1/2

"Talk with your neighbor about how you might make sense of this problem with repeated addition," I continued.

After a few minutes, I called on Juanita. She said, "I think you can do it by adding one-half over and over again. I did one-half plus one-half, like that, six times. I think the answer is three." I wrote on the board:

"How did you get the answer of three?" I asked.

Juanita responded, "One-half plus one-half is one whole, and you can do that three times, and you get three." I wrote:

Eddie added, "It's like if you had six times something else, you could add the something else six times, and that's what Juanita did with the one-halves."

"So, does this first statement work for multiplying with a fraction?" I asked. The students nodded their agreement, and I wrote OK next to the first statement.

I then pointed to the second statement:

2. Times means "groups of."

"Does it make sense to read 'six times one-half' as 'six groups of one-half'?" Most of the students nodded.

Saul added, "The answer is still three." I wrote OK next to the second statement and then pointed to the third statement:

3. A multiplication problem can be shown as a rectangle.

I asked, "Can we draw a rectangle to show six times one-half?" The students weren't sure.

"Suppose the problem were six times one," I said, writing 6 x 1 on the board. The students were familiar with using rectangles for whole number multiplication. I sketched a rectangle, saying as I did so, "One side of the rectangle is six units long and the other side of the rectangle is one unit long." I labeled the sides 6 and 1 and then divided the rectangle into six small squares.

"See if this rectangle helps you think about how I might draw a rectangle to show six times one-half," I said.

Kayla said, "Just cut it in half."

"Which way should I cut the rectangle?" I asked.

"Sideways," Kayla said. I split the rectangle as Kayla suggested, then erased the 1 and replaced it with 1 written twice. Also, I shaded in the bottom half to indicate that it wasn't part of the problem.

"The top half of the rectangle is six units by one-half unit and shows the problem six times one-half. The bottom shaded half shows the same problem again, but we don't need to consider both. How many squares are there in the unshaded rectangle? Does this still give an answer of three?"

Damien explained, "Two halves make a whole, and you do that three times, so the six halves make three whole squares. Three is still the answer."

"But what about if both the numbers are fractions?" Julio challenged.

"Let's try one," I said. I wrote on the board:

I decided to show the students a way to think about representing the problem with a rectangle. "When I draw a rectangle for a multiplication problem with fractions, I find it easier first to draw a rectangle with whole number sides. So, for this problem, I think about a rectangle that is one by one," I said. I drew a square on the board, labeled each side with a 1, and continued, "This rectangle is a square because both factors are the same. It shows that one times one is one. Now watch as I draw a rectangle inside this one with sides that each measure one-half." I divided the square, shaded in the part we didn't need to consider to show the 1/2-by-1/2 portion in the upper left corner, and labeled each side 1/2.

I said, "The part that isn't shaded has sides that are each one-half of a unit. How much of the one-by-one square isn't shaded?"

"One-fourth," several students responded.

Saul was skeptical. He asked, "You mean that one-half times one-half is one-fourth?" I nodded.

"I don't get it," he said.

"But do you agree that the unshaded rectangle has sides that are each one-half?" I asked. Saul nodded.

"But you're not sure that the answer of one-fourth is correct?" I asked. Again, Saul nodded.

"Let's see if the other statements can help you see why," I said. I knew that if the students thought of the problem as "one-half of one-half," they would agree with the answer of one-fourth. I planned to develop this idea, and I used the next statement to do so.

I pointed to the next statement:

4. You can reverse the order of the factors and the product stays the same.

Craig commented, "That should work." Others agreed.

"But it doesn't matter for one-half times one-half," Brendan said. "If you switch them, you still have the same problem."

I said, "Right, so let's think about this statement for the first problem we solved—six times one-half. What about if we think about the problem as one-half times six?" I wrote on the board:

I continued, "If we think about the times sign as 'groups of,' then one-half times six should be 'one-half groups of six.' But that doesn't sound right. It does make sense, however, to say 'one half of a group of six,' or 'one-half of six,' and leave off the 'groups' part. Both sound better, and they're still the same idea. What do you think 'one-half of six' could mean?"

"It's the same," Sabrina said. "One-half of six is three, so one-half times six is three, and that's the same as six times one-half."

I said, "Let's think about one-half times one-half the same way. What is one-half of one-half?"

I heard several answers. "A fourth." "A quarter." "One-fourth."

"So what do you think about reversing the order of the factors when the factors are fractions?" I asked.

The students agreed that it would work, so I wrote OK next to Statements 3 and 4.

"Let's look at the fifth statement," I said, pointing to it:

5. You can break numbers apart to make multiplying easier.

"Talk with your neighbor about how you could apply this statement to the problem six times one-half."

After a few moments, I called on Brendan. He said, "It works. You could break the six into twos, and then you do two times one-half three times. Two times one-half is one. One plus one plus one is three. So it works." I wrote on the board:

Anita said, "We split the six into four and two. Half of four is two and half of two is one and two plus one is three. It works." I recorded:

I wrote OK next to the statement. "We have one statement left," I said, pointing to it:

6. When you multiply two numbers, the product is larger than the factors unless one of the factors is zero or one.

I asked, "Does this statement hold true for six times one-half?"

"It doesn't work," Sachi said. "Three is smaller than six, so it doesn't work."

"Could we change the statement so that it does work?" I asked.

Julio said, "It should say at the end 'unless the factors are zero or one or a fraction.'" I edited the statement as Julio suggested:

6. When you multiply two numbers, the product is larger than the factors unless one of the factors is zero or one or a fraction.

I thought about how to respond. If one of the factors is a fraction less than one, then Julio's idea works. But if the fraction is more than one, Julio's idea might not work. I wanted to acknowledge Julio's idea but also give him the chance to refine it. I posed a problem that had a fraction as one of the factors for which the answer was greater than both of the factors. I said, "That's a good idea, but I think it needs a little more information. Think about this problem—six times three-halves. That's the same as six groups of three-halves." I wrote on the board:

"Talk with your neighbor about what the answer would be to this problem," I said. After a couple of minutes, I called on Craig.

"We got nine," he said. "We knew that three-halves is the same as one and a half, and one and a half plus one and a half is three, and three plus three plus three is nine, so the answer is nine." I recorded Craig's idea on the board:

Craig continued, "And nine is bigger than six or three-halves. So the statement doesn't work. It works the way it used to be, but it doesn't work the way you changed it."

"I think I can fix what I said," Julio said. "The fraction has to be smaller than one. So any number that is zero or one or in between makes an answer that is smaller than the factors."

"So what should I write to change the statement?" I asked.

Julio said, "At the end, write 'zero or one or a fraction that's smaller than one.'" I wrote on the board:

6. When you multiply two numbers, the product is larger than the factors unless one of the factors is zero or one or a fraction smaller than one.

Julio and others nodded. I wrote OK next to the revised statement.

This was just the first lesson I planned to teach about multiplying fractions. In later lessons, I further developed the students' understanding and skills.

Related Publication:
Teaching Arithmetic: Lessons for Multiplying and Dividing Fractions, Grades 5–6
by Marilyn Burns

From Online Newsletter Issue Number 12, Winter 2003–2004

View Lesson

Grades 4–5

When Is a Fraction Worth One-Half?

After the class had experience comparing two fractions by using one-half as a benchmark, I wrote on the board:

⁶⁄₁₂

"Raise your hand if you can explain why this fraction is equivalent to one-half," I said. I waited until every student had raised a hand. While the question was trivial for most students, I planned to build on their understanding and have a class discussion about different ways to determine that a fraction is equivalent to one-half. Also, I planned to compile the explanations they'd offer into a list and represent them algebraically, thus giving the students experience with using variables to describe general numerical relationships.

Before calling on any students to respond, I gave a direction. "Be sure to listen to what others say and see if your idea is the same or different. If you have a different way, then raise your hand again. I'm interested in seeing how many different ways we can come up with to explain what makes a fraction equivalent to one-half."

Jake reported first. "You add the top twice and see if it makes the bottom," he said.

I knew, or thought that I knew, what Jake was stating. But his response gave me the chance to push for more clarity from him.

"Tell me what you mean with the fraction I wrote on the board," I said.

"You go six plus six and you get twelve," he said.

"Oh," I said. "You added six to itself." Jake nodded his agreement.

"That works," I confirmed. "Can you say your idea again, but this time use the words numerator and denominator instead of top and bottom?"

Jake said, "You go numerator plus numerator and see if the answer is the denominator." I then wrote on the board:

If the numerator plus the numerator equals the denominator, then the fraction is worth ¹⁄₂.

"Who has a different way to decide if a fraction is worth one-half?" I asked. I called on Rosie.

She said, "Do the numerator times two and see if the answer is the denominator." I wrote on the board:

If the numerator times 2 equals the denominator, then the fraction is worth ¹⁄₂.

"I have another way," Donald said. "If the numerator goes into the denominator two times, then the fraction is one-half." I wrote:

If the numerator goes into the denominator two times, then the fraction is worth ¹⁄₂.

I said to the class, "My hand gets tired writing down your ideas. One of the benefits of mathematics is that we have symbols to describe ideas and don't have to use words all of the time. The symbols are like shortcuts. See if you understand how I can record Jake's, Rosie's, and Donald's ideas in mathematics, not English."

I turned to the board, explaining as I wrote. "Instead of writing numerator and denominator over and over, I'll just use a shortcut for each: n and d. Who can explain why this makes sense?" I had written:

ⁿ⁄_d

n = numerator

d = denominator

Carl answered, "You just used the first letter."

"Look at this fraction," I said, writing ³⁄₄ on the board. "For this fraction, n is three and d is four."

"But it's not one-half," Steven said.

"No, it's not," I agreed.

"I don't get why n is three and d is four," Gena said.

"Who can explain?" I asked. I called on Peter.

"Because three is the top number in the fraction, so it's the numerator," he said. "And four is the bottom number, so it's the denominator."

"But the fraction you wrote — n over d— isn't a real fraction," Gena said.

The idea of algebraic variables was new for these students and I tried to explain. "It's not a specific fraction," I said. "Suppose you went home and told your mom that your teacher gave you homework. If it were math homework, then you'd be referring to me. But if it were science homework, or a book report, or something for art, then you'd be talking about a different teacher. 'Teacher' is a general description; 'Ms. Burns' would be a specific description. In the same way, 'n over d' is a general name that could mean any fraction, but 'six-twelfths' refers to a specific fraction because you now know what numbers you're thinking of for the numerator and denominator."

I wasn't sure if Gena understood, but I pressed on. "Watch as I translate Jake's idea into a mathematical shortcut. If it makes sense to you, it sure will save us some writing energy." I wrote on the board next to the sentence I had written to describe Jake's idea:

Jake: If n + n = d, then ⁿ⁄_d = ¹⁄₂.

I continued, "And watch what I could write for Rosie's idea." I wrote:

Rosie: If n x 2 = d, then ²⁄_d = ¹⁄₂.

"What could I write for your idea, Donald, using n's and d's?" I asked.

Donald thought for a moment and then asked, "Can I come up and write it?" I agreed. He came up and used the notation for whole number division to record. He wrote:

d divided by n equals 2.

This notation for division isn't standard to algebraic representation, which I wanted Donald and the others to know. But I also wanted to honor Donald's contribution, which was correct in concept, but not in convention. "What you wrote makes sense to me," I said. "See if this way also describes your idea. It's the way you'll usually see your idea in math books." I wrote:

Donald: 2

I then returned to the discussion of other ways to see if a fraction were equivalent to one-half. I called on Gena, who now seemed more confident.

Gena said, "If the numerator is half of the denominator, then it works." I wrote on the board:

Gena: If n ¹⁄₂ = of d, then ⁿ⁄_d = ¹⁄₂.

George had another idea. "If you can divide the denominator by two and get the numerator, then it's one-half." I recorded:

George: If d ÷ 2 = n, then ⁿ⁄_d = ¹⁄₂.

I then said, "Raise your hand when you have in mind another fraction that's equivalent to one-half." After a moment, all but Jonathan and Connie had raised their hands.

"Do you have a fraction, Jonathan?" I asked. He nodded and raised a hand. So did Connie.

I started around the room having students tell me fractions, and I recorded their suggestions on the board. When I called on Addison, he said, "One hundred–two hundredths." There was an outbreak of giggles followed by a rash of other fraction suggestions that caused even more giggles: "Five hundred–one thousandths." "One thousand–two thousandths." "One million –two millionths." I wrote each of these on the board:

¹⁰⁰⁄₂₀₀ ⁵⁰⁰⁄_1,000 ^1,000⁄_2,000 ^1,000,000⁄_2,000,000

Ali took another direction when it was her turn. "Seven and a half–fifteenths," she said. I wrote on the board:

Others followed with similar fractions.

I then asked, "So how many fractions do you think you could write that are equivalent to one-half?"

"Infinity!" several answered at the same time.

I said, "I agree that there are an infinite number of fractions that are equivalent to one-half.

Representing your ideas algebraically as I did on the chart is a handy way to refer to many, many fractions."

I then gave the class an assignment. "I'm going to write ten fractions on the board," I explained. "Decide if each is equal to one-half, less than one-half, or more than one-half. On your paper, explain your reasoning for each."

I listed on the board the ten fractions the students were to consider:

1. ⁴⁄₈

2. ⁶⁄₁₃

3. ³⁄₅

4. ³⁄₆

5. ⁷⁄₁₀

6. ⁸⁄₁₅

7. ²⁵⁄₅₀

8. ²⁵⁄₅₁

9. ²⁵⁄₄₉

10. ^1,000⁄_2,000

See Figures 1 and 2 on the following pages for examples of how students worked on this assignment.

Figure 1. Brett used a chart to present the answers.

Figure 2. Tommy's paper showed his understanding.

From Online Newsletter Issue Number 4, Winter 2001–2002

Related Publication:
Teaching Arithmetic: Lessons for Introducing Fractions, Grades 4–5
by Marilyn Burns

View Lesson

Grades 6–8

Dr. Seuss Comes to Middle School Math Class

Last year Carrie DeFrancisco wanted to celebrate Dr. Seuss' birthday with her sixth-, seventh-, and eighth-grade students. The students were studying simplifying and evaluating expressions and solving one- and two-step equations. Carrie centered activities on the Dr. Seuss classic Green Eggs and Ham. "As you know, today our school is celebrating Dr. Seuss' birthday. I…

View Lesson

Grades 6–8

Using Questions in Math Lessons: Grades 5-8

In their book, Good Questions for Math Teaching, Why Ask Them and What to Ask, Grades 5–8, Lainie Schuster and Nancy Anderson provide a rich collection of questions for teachers to use in math lessons. Asking questions helps make math lessons dynamic and thought provoking and encourages students to make important mathematical connections. The topics…

View Lesson

Grades 6–8

A Drop of Water

After reading A Drop of Water aloud, I drew on the board a large blank four-column table for recording data. I labeled the first column Circle Number and then asked what a diameter of a circle was. Ariella said, "It goes all the way across the circle." Madison added, "It has to go through the…

View Lesson

Grades 6–8

What's Happening Here?

In this lesson, children reflect on the idea that when a number less than one is multiplied by a whole number, the answer will be smaller than the starting number. This fact conflicts with the belief of many students that multiplying a number always makes it larger. Materials calculators, 1 for each pair of students…

View Lesson

Grades 6–8

Eighteen Flavors

When I read "Eighteen Flavors" aloud, I asked the class why there was a "sniffle" at the end of the poem. "Because you can't eat ice cream off the ground!" Lori said. "Does it surprise you that these eighteen scoops would be on the ground?" I asked. "No, because that's too many for the cone…

View Lesson

Grades 6–8

Figuring Percents

Cathy wrote the following problem on the board: A school has 500 students. If a school bus holds 75 students, is there enough room on one bus for all of the school's left-handed students? Cathy ﬁrst had the students report on a class graph whether they were right-handed or left-handed. They analyzed the data and…

View Lesson

Grades 6–8

Numerical Expression Game

In this game, sixth grade students roll number cubes to create a numerical expression that has a value as close as possible to the given number. The key to learning mathematics is understanding the "why" behind the "how". HMH Into Math emphasizes the importance of establishing conceptual understanding and reinforces that understanding with procedural practice. The…

View Lesson

Grades 6–8

Hatchet

I began by asking the class who had read Hatchet. A large number of students raised their hands, and Nelson briefly summarized the book. At my request, he stopped at the point when Brian drops his hatchet into the lake. I then presented the following problem to the class: When Brian dropped his hatchet to…

View Lesson

Grades 6–8

Determine the Missing Angle

In this game, seventh grade students use angle measures and side lengths of triangles to determine the missing angle measure and to draw the accurate triangle using a protractor. The key to learning mathematics is understanding the "why" behind the "how". HMH Into Math emphasizes the importance of establishing conceptual understanding and reinforces that understanding with…

View Lesson

Grades 6–8

Writing Equations

In this game, eighth grade students practice writing equations in scientific notation and standard form. The key to learning mathematics is understanding the "why" behind the "how". HMH Into Math emphasizes the importance of establishing conceptual understanding and reinforces that understanding with procedural practice. The learning model asks students to first develop their reasoning before connecting…

View Lesson

Grades 6–8

Bulging Backpacks

To begin the lesson, I told the class, "Each of you will estimate the weight of each student's backpack and then we will find the actual weights, using a bathroom scale. We'll work together to create a graph known as a scatter plot. Then each of you will create and interpret a personal scatter plot…

View Lesson

Grades 6–8

Algebra Zapping Zombies A Lesson for Grades 6–8 Carmen Whitman

Overview of Lesson In this lesson students translate among representations so that their understanding can advance from words to tables to graphs to equations. The concepts are covered in the context of playing a video game where Zombies are zapped to score points and advance a level in the game. Introduce to students the background…

View Lesson

Grades 6–8

Basic Facts

This lesson is excerpted from Cheryl Rectanus's new book, So You Have to Teach Math? Sound Advice for Grades 6–8 Teachers (Math Solutions Publications, 2006). The focus of the book isn't primarily about classroom lessons but rather on responses from a master teacher to questions raised by middle school math teachers. (See a sample in…

View Lesson

Grades 6–8

Decimal Nim

I began by introducing the class to one version of the game Race to One. I explained the rules, "To play this decimal game, you first clear the calculator so that the display reads zero. Then, taking turns, each player adds either one-tenth or two tenths to the total on the display. The winner is…

View Lesson

Grades 6–8

First, Second

After reading Daniil Kharms's First, Second to a class of eighth graders, I wrote the following on the board: A tall man, an average man, and a short man each walked with different length paces. The tall man walked five meters in five paces. The average man walked two meters in five paces. The short…

View Lesson

Grades 6–8

The Incredible Shrinking Teacher

Nancy Anderson's middle school students had prior experience studying ratios and proportions: they were familiar with the concept of a ratio as a comparison of two measures; they had solved problems that required them to determine which two measures to compare and to reason about how they compared; and they had used the process of…

View Lesson

Grades 6–8

The Rocket Pattern

In this introductory lesson on algebraic thinking, students explore several stages of a rocket built with pattern blocks, record the number of blocks for each stage on T-charts, and find rules to describe the pattern. This activity appears in Ann Lawrence and Charlie Hennessy's new book, Lessons for Algebraic Thinking, Grades 6–8 (Math Solutions Publications,…

View Lesson

Grades 6–8

Roll of Thunder, Hear My Cry

Mildred D. Taylor's Roll of Thunder, Hear My Cry (Puffin, 1991) is the story of a young African American girl, Cassie, and her family living in Mississippi in the 1930s, during which time African Americans were threatened, ridiculed, and burned alive. In this investigation, students are able to develop a more personal understanding of what…

View Lesson

Grades 6–8

Closest to One Thousand

The following lesson is adapted from Len Sparrow and Paul Swan's Learning Math with Calculators. The book is organized into two sections: the first addresses a broad range of teachers' questions and concerns about using calculators for teaching math; the second is a collection of classroom-tested activities using calculators, all chosen for the purpose of…

View Lesson

Grades 6–8

Scaling Up!

Overview of Lesson In this lesson students work on multiple measurement concepts, including measuring length, scale, ratio, and proportion. Students apply all the concepts in an activity that involves enlarging a picture to scale so that it fits on a piece of graph paper. Students must take measurements, determine the ratio they will need to…

View Lesson

Grades 6–8

Data Analysis

Asking "good" questions—questions that help students make sense of math—lies at the heart of good math teaching. In Good Questions for Math Teaching: Why Ask Them and What to Ask, Grades 5–8, Lainie Schuster and Nancy Anderson provide teachers with questions across seven math strands. The questions are open-ended and offer opportunities for a range of…

View Lesson

Grades 6–8

Up to One Million

Annette Raphel taught this lesson to her seventh-grade students in Milton, Massachusetts. The lesson provided a meaningful way for her students to manipulate data, promoted the students' number sense, and gave them much-needed experience with large numbers. At the end of the lesson, Annette includes a bibliography of children's books that are useful for other…

View Lesson